An AC source of frequency ω when fed into a RC series circuit, current is recorded to be l. If now frequency is changed to ω4 (keeping voltage same), the current is found to 1/2. The ratio of reactance to resistance at original frequency ω is
2
12
1√2
None of these
The correct Answer is:B
To solve the problem step by step, we will analyze the given information about the RC circuit and the changes in frequency and current.
Step 1: Understand the Circuit and Given Information
We have an RC series circuit with:
- Initial frequency: ω
- Current at frequency ω: I
- New frequency: ω4
- Current at frequency ω4: I2
Step 2: Write the Expression for Current
The current I in an RC circuit is given by:
I=VZ
where Z is the impedance of the circuit.
Step 3: Calculate Impedance at Frequency ω
The impedance Z at frequency ω is given by:
Z1=√R2+X2C
where XC is the capacitive reactance given by:
XC=1ωC
Thus,
Z1=√R2+(1ωC)2
Step 4: Write the Expression for Current at Frequency ω
Using the impedance, the current at frequency ω can be expressed as:
I=VZ1=V√R2+(1ωC)2
Step 5: Calculate Impedance at Frequency ω4
At frequency ω4, the capacitive reactance becomes:
X′C=1ω4C=4ωC
Thus, the new impedance Z2 is:
Z2=√R2+X′2C=√R2+(4ωC)2
Step 6: Write the Expression for Current at Frequency ω4
The current at frequency ω4 is:
I2=VZ2=V√R2+(4ωC)2
Step 7: Set Up the Equation
From the two expressions for current, we have:
I2=V√R2+(4ωC)2
and
I=V√R2+(1ωC)2
Step 8: Equate the Two Expressions
We can equate the two expressions for current:
V√R2+(1ωC)2=2⋅V√R2+(4ωC)2
Cancelling V and squaring both sides gives:
R2+(1ωC)2=4(R2+(4ωC)2)
Step 9: Simplify the Equation
Expanding and simplifying leads to:
R2+1ω2C2=4R2+64ω2C2
Rearranging gives:
−3R2=63ω2C2
Thus,
3R2=63ω2C2
Step 10: Solve for XCR
From XC=1ωC, we can express the ratio:
XCR=1R⋅ωC
Substituting ωC=2R gives:
XCR=12
Final Answer
The ratio of reactance to resistance at the original frequency ω is:
XCR=12
We have an RC series circuit with:
- Initial frequency: ω
- Current at frequency ω: I
- New frequency: ω4
- Current at frequency ω4: I2
The current I in an RC circuit is given by:
I=VZ
where Z is the impedance of the circuit.
The impedance Z at frequency ω is given by:
Z1=√R2+X2C
where XC is the capacitive reactance given by:
XC=1ωC
Thus,
Z1=√R2+(1ωC)2
Using the impedance, the current at frequency ω can be expressed as:
I=VZ1=V√R2+(1ωC)2
At frequency ω4, the capacitive reactance becomes:
X′C=1ω4C=4ωC
Thus, the new impedance Z2 is:
Z2=√R2+X′2C=√R2+(4ωC)2
The current at frequency ω4 is:
I2=VZ2=V√R2+(4ωC)2
From the two expressions for current, we have:
I2=V√R2+(4ωC)2
and
I=V√R2+(1ωC)2
We can equate the two expressions for current:
V√R2+(1ωC)2=2⋅V√R2+(4ωC)2
Cancelling V and squaring both sides gives:
R2+(1ωC)2=4(R2+(4ωC)2)
Expanding and simplifying leads to:
R2+1ω2C2=4R2+64ω2C2
Rearranging gives:
−3R2=63ω2C2
Thus,
3R2=63ω2C2
From XC=1ωC, we can express the ratio:
XCR=1R⋅ωC
Substituting ωC=2R gives:
XCR=12
The ratio of reactance to resistance at the original frequency ω is:
XCR=12
Topper's Solved these Questions
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Assignment (Section - C ) (Objective Type Questions) ( More than one option are correct)
2 Videos
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Assignment (Section - D) (Linked Comprehension Type Questions)
3 Videos
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Assignment (Section - A) ( Objective Type Questions ( One option is correct))
40 Videos
- ATOMSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ATOMSEXERCISE - ASSIGNMENT SECTION J (Aakash Challengers )
5 Videos
Similar Questions
An a.c. source of angular frequency ω is fed across a resistor R and a capacitor C in series. The current registered is I. If now the frequency of the source is changed to ω/3 (but maintaining the same voltage), the current in the circuit is found to be halved. calculate the ratio of reactance to resistance at the original frequency ω.
View SolutionAn AC voltage source is applied across an R-C circuit. Angular frequency of the source is ω, resistance is R and capacitance is C. The current registered is I. If now the frequency of source is changed to ω2 (but maintaining the same voltage), the current in the circuit is found to be two third. calculate the ratio of reactance to resistance at the original frequency ω .
View SolutionAn ac source of angular frequency ω is fed across a resistor R and a capacitor C in series. The current registered is I. If now the freqency of source is chaged to (ω)/3 (but maintainging the same voltage), the current in the circuit is found to be halved. Calculate the ration of hte reactance to resistance at the original frequency ω.
View SolutionFor an LCR series circuit with an ac source of angular frequency ω.
View SolutionFor an LCR series circuit with an aac source of angular frequency ω.
View SolutionA series combination of resistor (R), capacitor (C) is connected to an AC source of angular frequency ω. Keeping the voltage same, If the frequency is changed to Ω3, the current becomes half of the original current. Then, the ratio of the capacitance reactance and resistance at the former frequency is
View SolutionIn a series LCR circuit, operated with an ac of angular frequency ω , the total impedance is
View SolutionAt a certain frequency ω1, the reactance of a certain capacitor equals that of a certain inductor. If the frequency is changed toω2=2ω1, the raito of reactance of the inductor to that of the capacitor is :
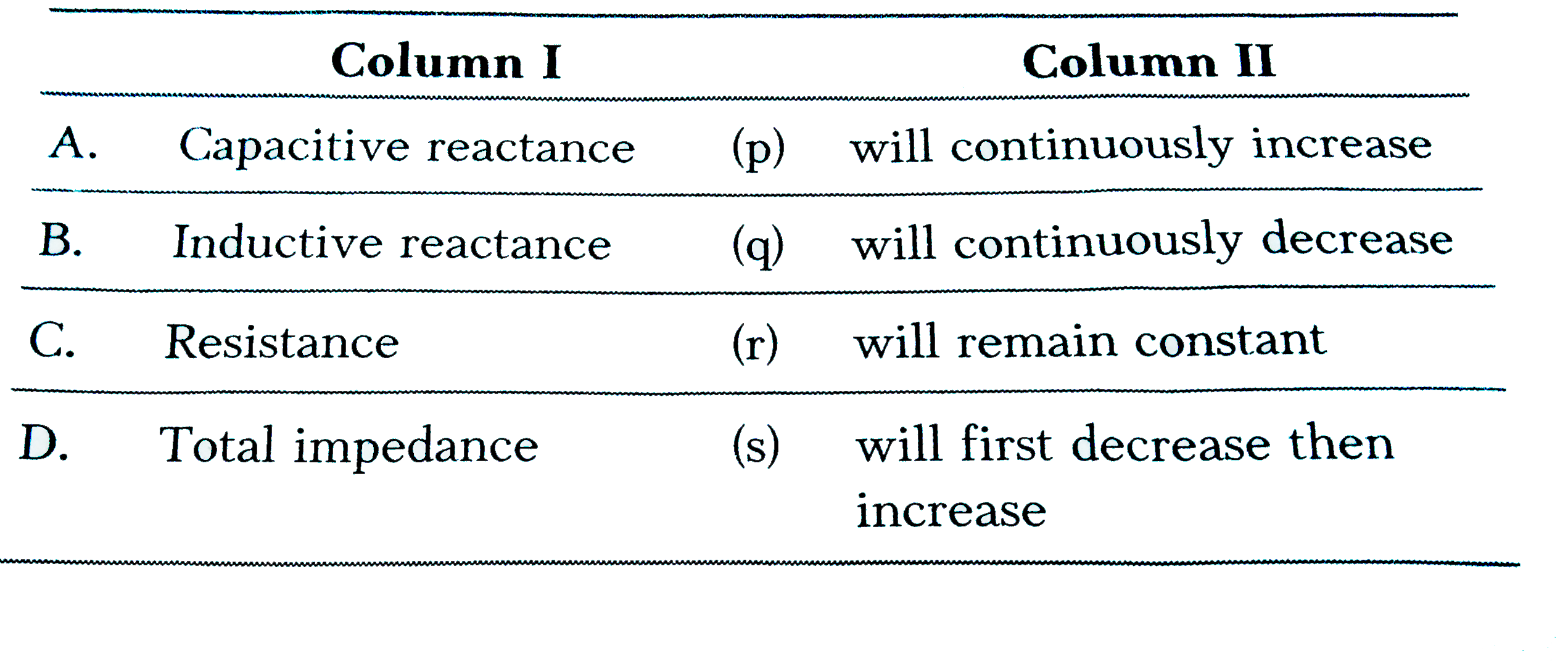
View SolutionAngular frequency ω in an AC, L-C-R series circuit is gradually increased. Then, match the following two columns.
View SolutionAssertion : In an L−R series circuit in AC, current in the circuit will decrease with increase in frequency.
Reason : Phase difference between current function and voltage function will increase with increase in frequency.
View Solution
