A force F is applied at the centre of a disc of mass M. The minimum value of coefficient of friction of the surface for rolling is
F2Mg
F3Mg
2F5Mg
2F7Mg
The correct Answer is:B
To solve the problem of finding the minimum value of the coefficient of friction for rolling when a force F is applied at the center of a disc of mass M, we can follow these steps:
1. Identify the Forces Acting on the Disc:
- A force F is applied at the center of the disc.
- The frictional force f acts at the point of contact with the surface to prevent slipping.
- The normal force N acts vertically upward, equal to the weight of the disc, N=Mg.
2. Set Up the Equations of Motion:
- For the disc to roll without slipping, the condition a=Rα must hold, where a is the linear acceleration of the center of mass, R is the radius of the disc, and α is the angular acceleration.
- The net force acting on the disc in the horizontal direction is given by:
F−f=Ma
3. Torque About the Center of Mass:
- The torque τ about the center of mass due to the frictional force is:
τ=fR
- According to Newton's second law for rotation, this torque is also equal to the moment of inertia I times the angular acceleration α:
fR=Iα
4. Moment of Inertia of the Disc:
- The moment of inertia I of a disc about its center is given by:
I=12MR2
5. Relate Angular Acceleration to Linear Acceleration:
- Using the relationship α=aR, we can substitute α into the torque equation:
fR=(12MR2)(aR)
- Simplifying this gives:
fR=12MaR
- Dividing both sides by R:
f=12Ma
6. Substituting for Acceleration:
- From the linear motion equation F−f=Ma, substitute f:
F−12Ma=Ma
- Rearranging gives:
F=32Ma
- Therefore, solving for a:
a=2F3M
7. Substituting Back to Find Friction:
- Now substitute a back into the friction equation:
f=12M(2F3M)=F3
8. Finding the Coefficient of Friction:
- The frictional force must also satisfy the condition f=μN, where N=Mg:
μMg=F3
- Thus, the coefficient of friction μ is:
μ=F3Mg
Final Answer
The minimum value of the coefficient of friction for rolling is:
μ=F3Mg
- A force F is applied at the center of the disc.
- The frictional force f acts at the point of contact with the surface to prevent slipping.
- The normal force N acts vertically upward, equal to the weight of the disc, N=Mg.
- For the disc to roll without slipping, the condition a=Rα must hold, where a is the linear acceleration of the center of mass, R is the radius of the disc, and α is the angular acceleration.
- The net force acting on the disc in the horizontal direction is given by:
F−f=Ma
- The torque τ about the center of mass due to the frictional force is:
τ=fR
- According to Newton's second law for rotation, this torque is also equal to the moment of inertia I times the angular acceleration α:
fR=Iα
- The moment of inertia I of a disc about its center is given by:
I=12MR2
- Using the relationship α=aR, we can substitute α into the torque equation:
fR=(12MR2)(aR)
- Simplifying this gives:
fR=12MaR
- Dividing both sides by R:
f=12Ma
- From the linear motion equation F−f=Ma, substitute f:
F−12Ma=Ma
- Rearranging gives:
F=32Ma
- Therefore, solving for a:
a=2F3M
- Now substitute a back into the friction equation:
f=12M(2F3M)=F3
- The frictional force must also satisfy the condition f=μN, where N=Mg:
μMg=F3
- Thus, the coefficient of friction μ is:
μ=F3Mg
The minimum value of the coefficient of friction for rolling is:
μ=F3Mg
Similar Questions
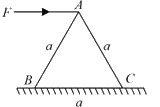
A horizontal force F is applied at the top of an equilateral triangular block having mass m. The minimum coefficient of friction required to topple the block before translation will be
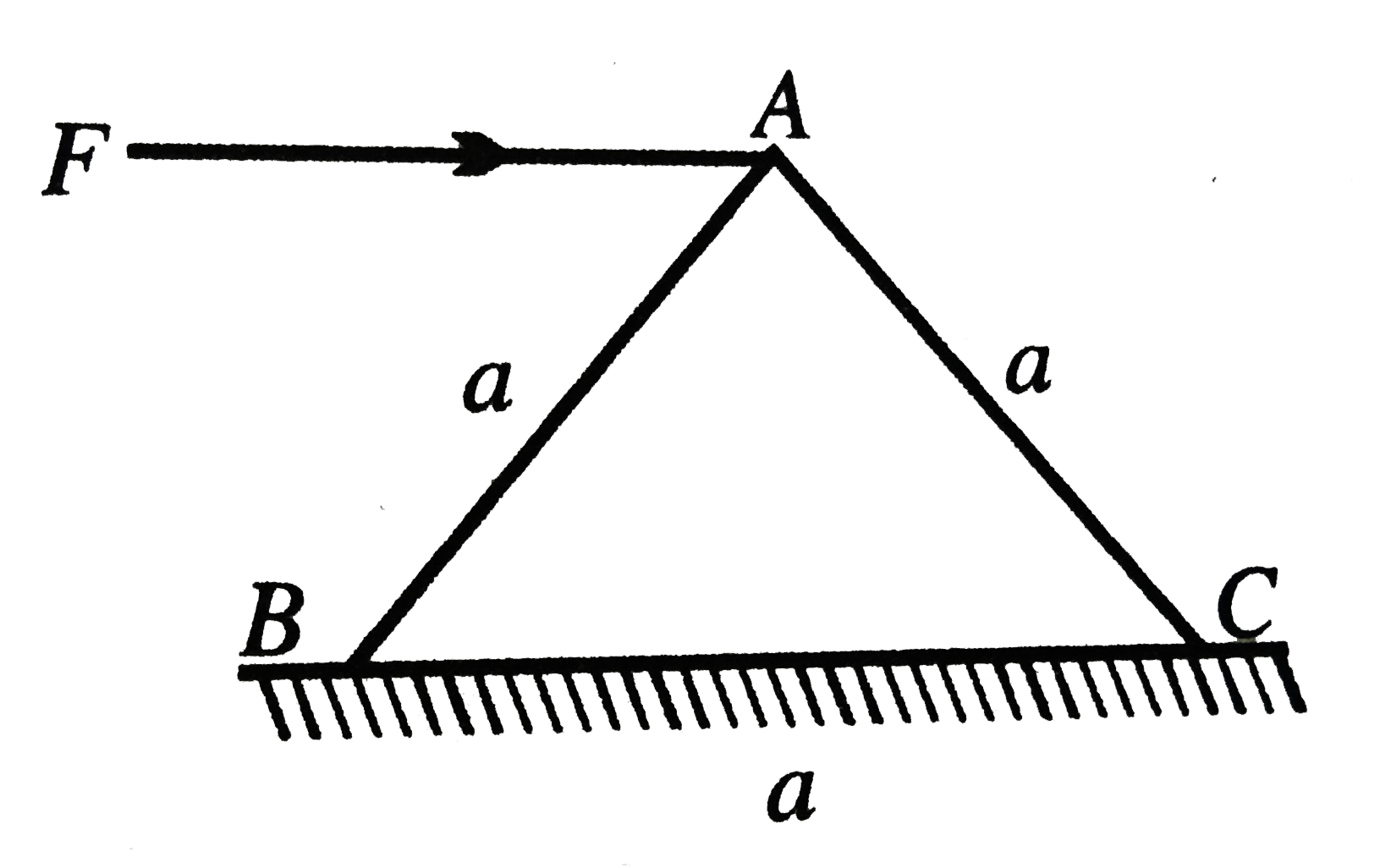
View SolutionA disc of mass M and radius R is placed on a rough horizontald surface. A light rod of length 2R is fixed to the disc at pointt A as shown in figure and force 32 Mg is applied at the other end of the rod. Find the minimum value of coefficient of friction (upto on decimal place ) between disc and horizontal surface, so that disc starts to roll without slipping .
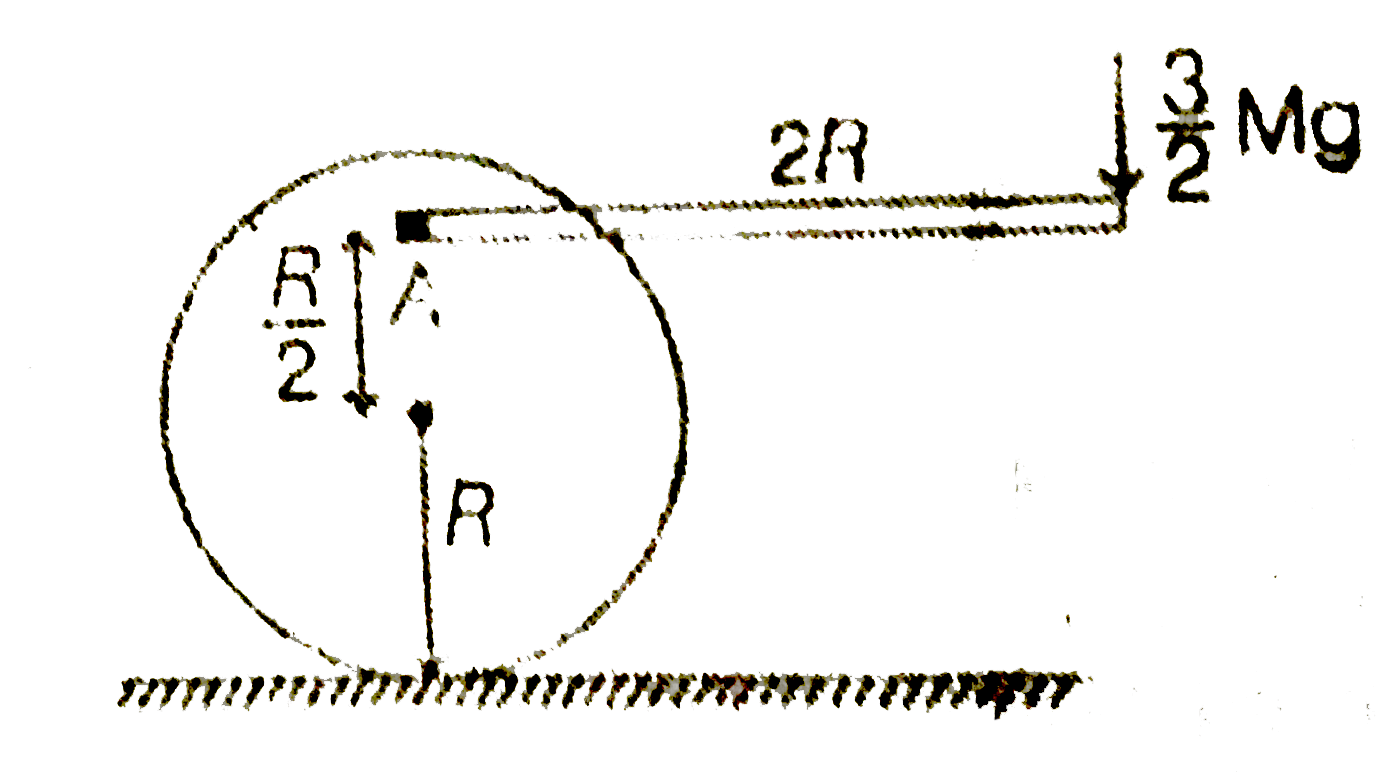
View SolutionA horizontal force F is applied at the top of an equilateral triangular block having mass m. The minimum coefficient of friction required to topple the block before translation is μ,find 100μ?(√3=1.74)
View SolutionA solid sphere of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between sphere and plank to prevent slipping.

A Horizontal force F is applied on the plank. What is the maximum value of F, if μ is coefficient of friction between sphere movment and plank and there is no slipping ?
View SolutionA force of 100 N is applied on a block of mass 3 kg as shown in figure. The coefficient of friction between the surface and the block is 14 .Then the friction force acting on the block is
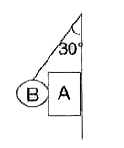
View SolutionIn the shown, A and B of equal mass are in equilibrium. Only surface between A and wall is rough. The minimum value of coefficient of friction is √n. The value of n is
View SolutionA force F is applied at the top of a ring of mass M and radius R placed on a rough horizontal surface as shown in figure. Friction is sufficient to prevent slipping. The friction force acting on the ring is:

View Solution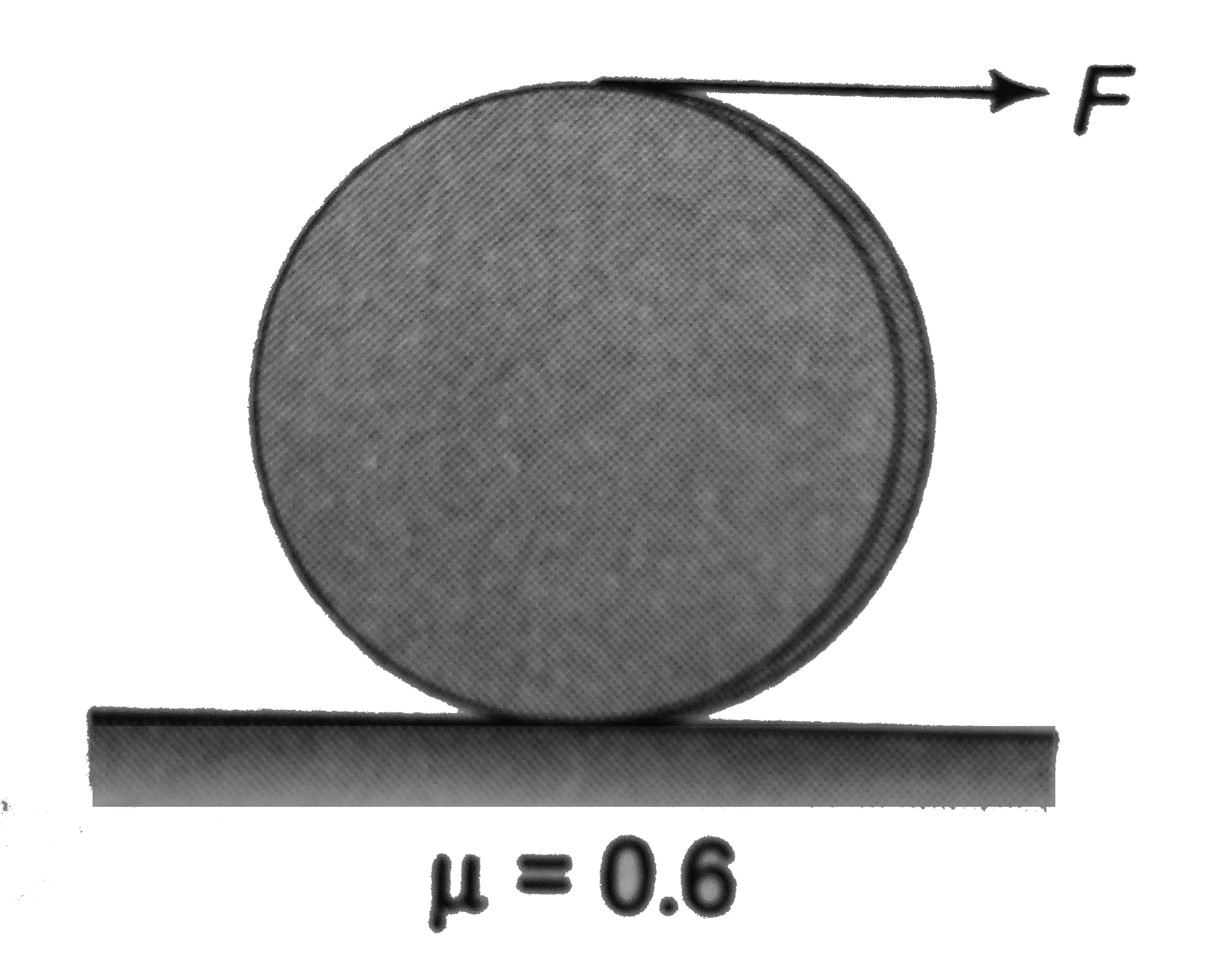
In the figure shown, a force F is applied at the top of a disc of mass 4 kg and radius 0.25 m. find maximum velue of F for no slipping.
View SolutionA force (F=Mg) is applied on the top of a circular ring of mass M and radius R kept on a rough horizontal surface. If the ring rolls without slipping, then minimum coefficient of friction required is
_PHY_E05_236_Q01.png)
View SolutionFind the minimum value of horizontal force (F) required on the block of mass m to keep it at rest on the wall. Given the coefficient of friction between the surfaces is μ.
View Solution