A: If length of a spring is halved, then its force constant becomes double.
R: The spring constant is inversely proportional to length of spring.
If both Assertion & Reason are true and the reason is the correct explanation of the assertion, then mark (1)
If both Assertion & Reason are true but the reason is not the correct explanation of the assertion, then mark (2)
If Assertion is true statement but Reason is false then mark (3)
If both Assertion and Reason are false statements, then mark (4)
The correct Answer is:A
To solve the assertion and reason question regarding the relationship between the length of a spring and its spring constant (force constant), we will follow these steps:
Step 1: Understand the Assertion
The assertion states: "If the length of a spring is halved, then its force constant becomes double."
Step 2: Understand the Reason
The reason states: "The spring constant is inversely proportional to the length of the spring."
Step 3: Recall the Relationship
The spring constant K is related to the length L of the spring by the formula:
K∝1L
This means that if the length of the spring decreases, the spring constant increases.
Step 4: Analyze the Changes
If the original length of the spring is L and the original spring constant is K, when the length is halved:
L′=L2
Step 5: Apply the Inverse Proportionality
From the relationship K⋅L=constant, we can write:
K⋅L=K′⋅L′
Substituting L′:
K⋅L=K′⋅L2
Step 6: Solve for the New Spring Constant
Rearranging the equation gives:
K′=2K
This indicates that the new spring constant K′ is indeed double the original spring constant K.
Step 7: Conclusion
Since both the assertion and the reason are true, and the reason correctly explains the assertion, we conclude that:
- The assertion is true.
- The reason is true.
- The reason is a correct explanation of the assertion.
Final Answer
Both assertion and reason are true, and the reason is the correct explanation of the assertion.
---
The assertion states: "If the length of a spring is halved, then its force constant becomes double."
The reason states: "The spring constant is inversely proportional to the length of the spring."
The spring constant K is related to the length L of the spring by the formula:
K∝1L
This means that if the length of the spring decreases, the spring constant increases.
If the original length of the spring is L and the original spring constant is K, when the length is halved:
L′=L2
From the relationship K⋅L=constant, we can write:
K⋅L=K′⋅L′
Substituting L′:
K⋅L=K′⋅L2
Rearranging the equation gives:
K′=2K
This indicates that the new spring constant K′ is indeed double the original spring constant K.
Since both the assertion and the reason are true, and the reason correctly explains the assertion, we conclude that:
- The assertion is true.
- The reason is true.
- The reason is a correct explanation of the assertion.
Both assertion and reason are true, and the reason is the correct explanation of the assertion.
Topper's Solved these Questions
- OSCILLATIONSBook:AAKASH INSTITUTE ENGLISHChapter:OSCILLATIONSExercise:Assignment (Section C) (PREVIOUS YEARS QUESTIONS)
Explore 43 Videos
- NUCLEIBook:AAKASH INSTITUTE ENGLISHChapter:NUCLEIExercise:ASSIGNMENT (SECTION-D)
Explore 10 Videos
- PHYSICAL WORLDBook:AAKASH INSTITUTE ENGLISHChapter:PHYSICAL WORLDExercise:ASSIGNMENT (Section-B)
Explore 4 Videos
Similar Questions
Knowledge Check
Hooke's law states that the force needed to keep a spring stretched x units beyond its natural length is directly proportional to x. if a spring has a natural length of 10 cm, and a force of 40 N (newtons) is required to keep the spring stretched to a length to 15 cm , what force , in newtons, will be needed to keep the spring stretched to a length of 14 cm ?
A8B32C37.3D50
A spring of spring constant k is cut in three equal pieces. The spring constant of each part will be
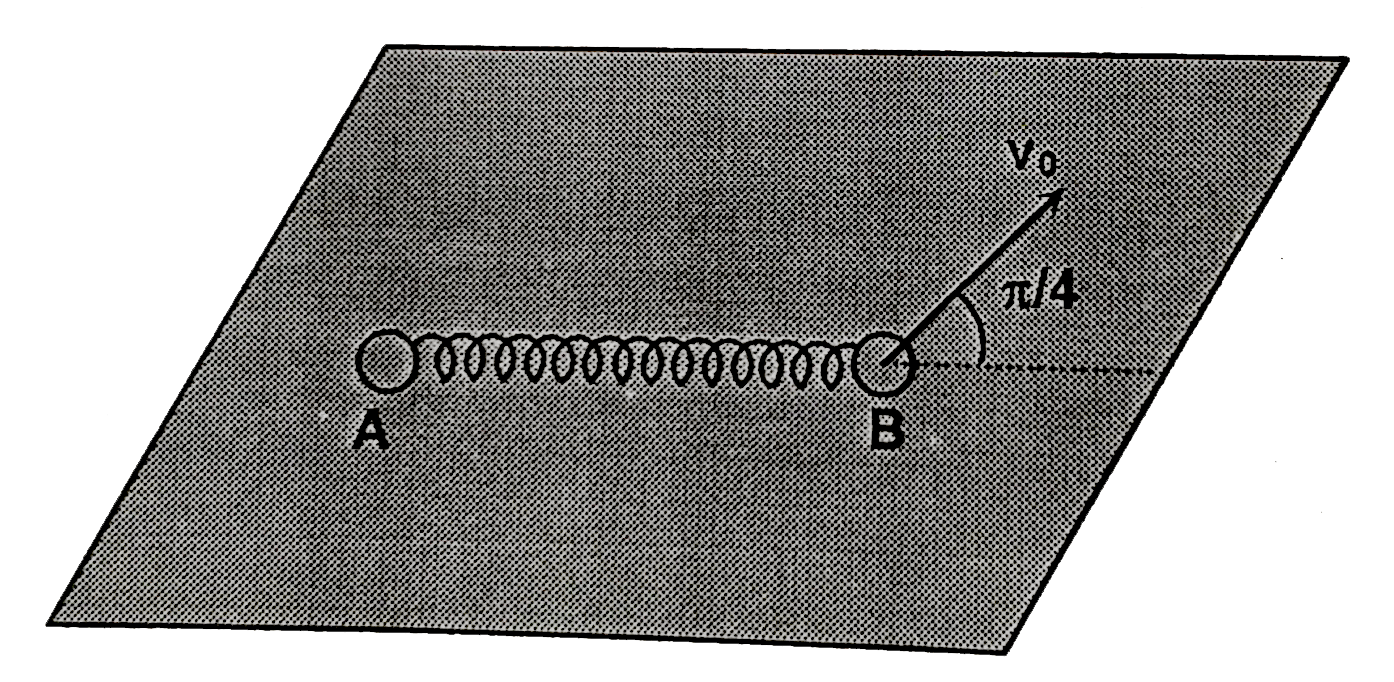
View SolutionA dumbbell consists of two balls A and B of mass m=1 kg and connected by a spring. The whole system is placed on a smooth horizontal surface as shown in the figure. Initially the spring is at its natural length, the ball B is imparted a velocity v0=8√7m/s in the direction shown. The spring constant of the spring is adjusted so that the length of the spring at maximum elongation is twice that of the natural, length of the spring. Find the maximum potential energy stored (in Joule) in the spring during the motion.
View SolutionTwo springs have their force constants in the ratio of 3:4. Both the springs are stretched by applying equal force F. If elongation in first spring is x then elogation is second spring is
View SolutionThe block of mass m is released when the spring was in its natrual length. Spring constant is k. Find the maximum elongation of the spring.

View SolutionA spring of certain length and having spring constant k is cut into two pieces of length in a ratio 1:2. The spring constants of the two pieces are in a ratio :
View SolutionWhich of the following graph depicts spring constant k versus length l of the spring correctly
View SolutionA ball of mass m is attached to the lower end of a light vertical spring of force constant K. The upper end of the spring is fixed. The ball is released from rest with the spring at its normal ( unstretched ) length, and comed to rest again after descending through a distance x.
View Solution