The length of a vertical pole at the surface of a lake of water (μ=43) is 24 cm. Then to an under-water fish just below the water surface the tip of the pole appeard to be
18 cm above the surface
24 cm above the surface
32 cm above the surface
36 cm above the surface
The correct Answer is:C
To solve the problem, we need to find the apparent height of the tip of a vertical pole when viewed by a fish just below the surface of the water. The given data includes:
- The actual length of the pole (real height) = 24 cm
- The refractive index of water (μ) = 4/3
1. Understand the Concept of Apparent Height:
The apparent height of an object when viewed from a different medium (in this case, from water to air) is affected by the refractive index of the medium. The formula to relate the real height (h) and the apparent height (h') is given by:
h′=hμ
where μ is the refractive index of the medium.
2. Identify the Given Values:
- Real height (h) = 24 cm
- Refractive index (μ) = 4/3
3. Calculate the Apparent Height:
Using the formula:
h′=hμ=24 cm4/3
To simplify this, we can multiply by the reciprocal of the refractive index:
h′=24 cm×34
4. Perform the Calculation:
h′=24×34=24×0.75=18 cm
5. Convert Apparent Height to Above Water Surface:
Since the fish is just below the water surface, the apparent height of the tip of the pole above the water surface will be:
Height above water surface=Real height+Apparent height
Therefore, the total height above the water surface is:
Total height=24 cm+18 cm=42 cm
Final Answer:
The tip of the pole appears to be 42 cm above the water surface to the fish just below the surface.
---
- The refractive index of water (μ) = 4/3
The apparent height of an object when viewed from a different medium (in this case, from water to air) is affected by the refractive index of the medium. The formula to relate the real height (h) and the apparent height (h') is given by:
h′=hμ
where μ is the refractive index of the medium.
- Real height (h) = 24 cm
- Refractive index (μ) = 4/3
Using the formula:
h′=hμ=24 cm4/3
To simplify this, we can multiply by the reciprocal of the refractive index:
h′=24 cm×34
h′=24×34=24×0.75=18 cm
Since the fish is just below the water surface, the apparent height of the tip of the pole above the water surface will be:
Height above water surface=Real height+Apparent height
Therefore, the total height above the water surface is:
Total height=24 cm+18 cm=42 cm
The tip of the pole appears to be 42 cm above the water surface to the fish just below the surface.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - B)
35 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - C)
80 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - EXERCISE
45 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
4 Videos
- SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )EXERCISE - Assignment SECTION - D (Assertion & reason type Question)
10 Videos
Similar Questions
_E01_107_Q01.png)
Two very wide parallel glass plates are held vertically at a small separation d, and dipped in water. Some water climbs up in the gap between the plate. Let S be the surface tension of water P0= atmospheric pressure, P= pressure of water just below the water surface in the region between the plates-
View SolutionWhen an air bubble rise from the bottom of a deep lake to a point just below the water surface, the pressure of air inside the bubble
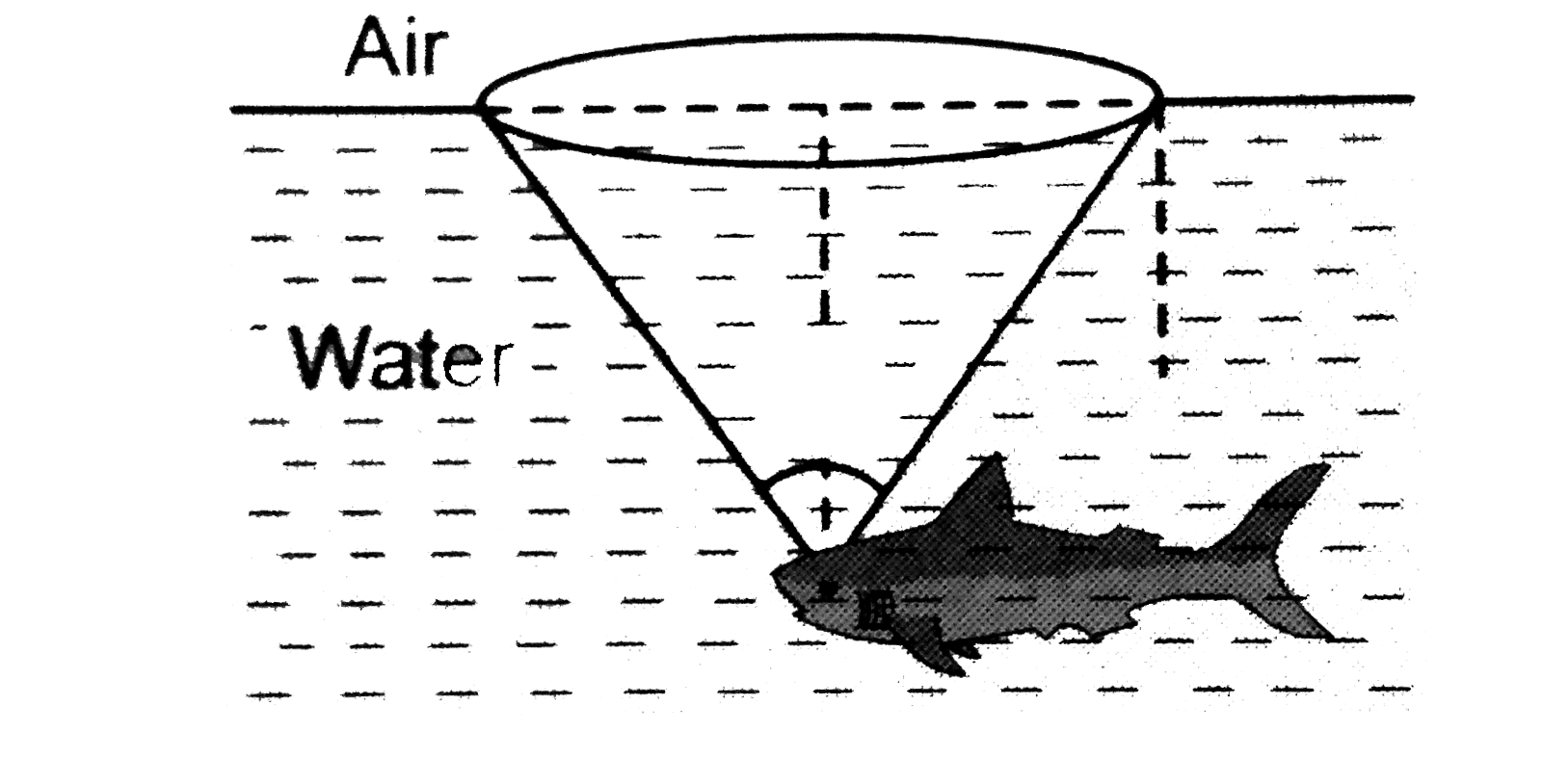
View SolutionA fish is a little away below the surface of a lake. If the critical angle is 49∘ , then the fish could see things above the water surface withing an angular range of θ∘ where
View SolutionThe length of needle foating on the surface of water is 1.5 cm the force in addition to its weight required to lift the needle from water surface will be (surface tension of water =7.5N/cm)
View SolutionIn a pond of water, a flame is held 2 m above the surface of the water. A fish is at depth of 4 m from the water surface. Refractive index of water is 43. The apparent height of the flame from the eyes of fish is -
View SolutionA ray of light travelling in glass (μg=3/2) is incident on a horizontal glass-air surface at the critical angle θC. If a thin layer of water (μw=4/3) is now poured on the glass-air surface. At what angle will the ray of light emerges into water at glass-water surface?
View SolutionThe temperature of water at the surface of a deep lake is 2∘C. The temperature expected at the bottom is
View SolutionA point source of light is placed at a distance hbelow the surface of a large deep lake.What is the percentage of light energy that escapes directly form the water surface if μof the water=4/3?(neglet partial reflection)
View SolutionA fish looking up through the water sees the outside world contained in a circular horizon. If the refractive index of water is 43 and the fish is 12 cm below the surface, the radius of this circle is cm is
View SolutionA point source of light is placed inside water and a thin converging lens of focal length f is placed just outside the surface of water. The image of source is formed at a distance of 50 cm from the surface of water. When the lens is placed just inside the water surface the image is formed at a distance of 40 cm from the surface of water. if focal length of the lens in air is f=100k8 cm, then find the value of k. (given refractive index of lens is 3/2 and that of water is 4/3 and in both cases image is formed inside water for the viewer in air).
View Solution
