A car of mass m has an engine which can deliver power P. The minimum time in which car can be accelerated from rest to a speed v is :-
The correct Answer is:A
To solve the problem of determining the minimum time in which a car of mass m can accelerate from rest to a speed v given that the engine can deliver power P, we can follow these steps:
1. Understand the relationship between work, power, and time:
- The work done by the car's engine is equal to the power delivered multiplied by the time taken. This can be expressed as:
W=P⋅t(Equation 1)
2. Determine the change in kinetic energy:
- Since the car starts from rest, its initial kinetic energy (KEi) is 0. The final kinetic energy (KEf) when the car reaches speed v is given by:
KEf=12mv2
- Therefore, the change in kinetic energy (ΔKE) is:
ΔKE=KEf−KEi=12mv2−0=12mv2(Equation 2)
3. Equate the work done to the change in kinetic energy:
- The work done on the car is also equal to the change in kinetic energy. Thus, we can set the two equations equal to each other:
P⋅t=12mv2
4. Solve for time t:
- Rearranging the equation to solve for t:
t=12mv2P
- This simplifies to:
t=mv22P
5. Final result:
- The minimum time in which the car can accelerate from rest to speed v is:
t=mv22P
Final Answer:
The minimum time t is given by:
t=mv22P
- The work done by the car's engine is equal to the power delivered multiplied by the time taken. This can be expressed as:
W=P⋅t(Equation 1)
- Since the car starts from rest, its initial kinetic energy (KEi) is 0. The final kinetic energy (KEf) when the car reaches speed v is given by:
KEf=12mv2
- Therefore, the change in kinetic energy (ΔKE) is:
ΔKE=KEf−KEi=12mv2−0=12mv2(Equation 2)
- The work done on the car is also equal to the change in kinetic energy. Thus, we can set the two equations equal to each other:
P⋅t=12mv2
- Rearranging the equation to solve for t:
t=12mv2P
- This simplifies to:
t=mv22P
- The minimum time in which the car can accelerate from rest to speed v is:
t=mv22P
The minimum time t is given by:
t=mv22P
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - B)
35 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - C)
80 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - EXERCISE
37 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
A car of mass m has an engine which can deliver constant power P.the maximum speed that the car can attain in t seconds in
View SolutionA car is moving up the plane. Angle of inclination is θ and coeffcient of friction is μ
(a)What is the condition in which car can be acceleration?
If this condition is satisfied then find (b) maximum acceleration of the car.
(c) minimum retardation of the car.
(d) maximum retardation of the car.
View Solution
Knowledge Check
A car can be stopped within a distance of s when its speed is v. What is the minimum distance within which the car can be stopped during the same time when its speed is nv ?
AnsBs/nCn2sDs/n2The maximum acceleration that can be produced by the engine of a vehicle is 5ms−2 and the maximum retardation is brake can produce is 10ms−2. Then the minimum time during which it can cover a distance of 60 m is
A6 sB5 sC36 sD24 s.
On a horizontal rough road , value of coefficient of friction μ=0.4.Find the minimum time in which a distance of 400m can be covered. The car start from rest and finally comes to rest.
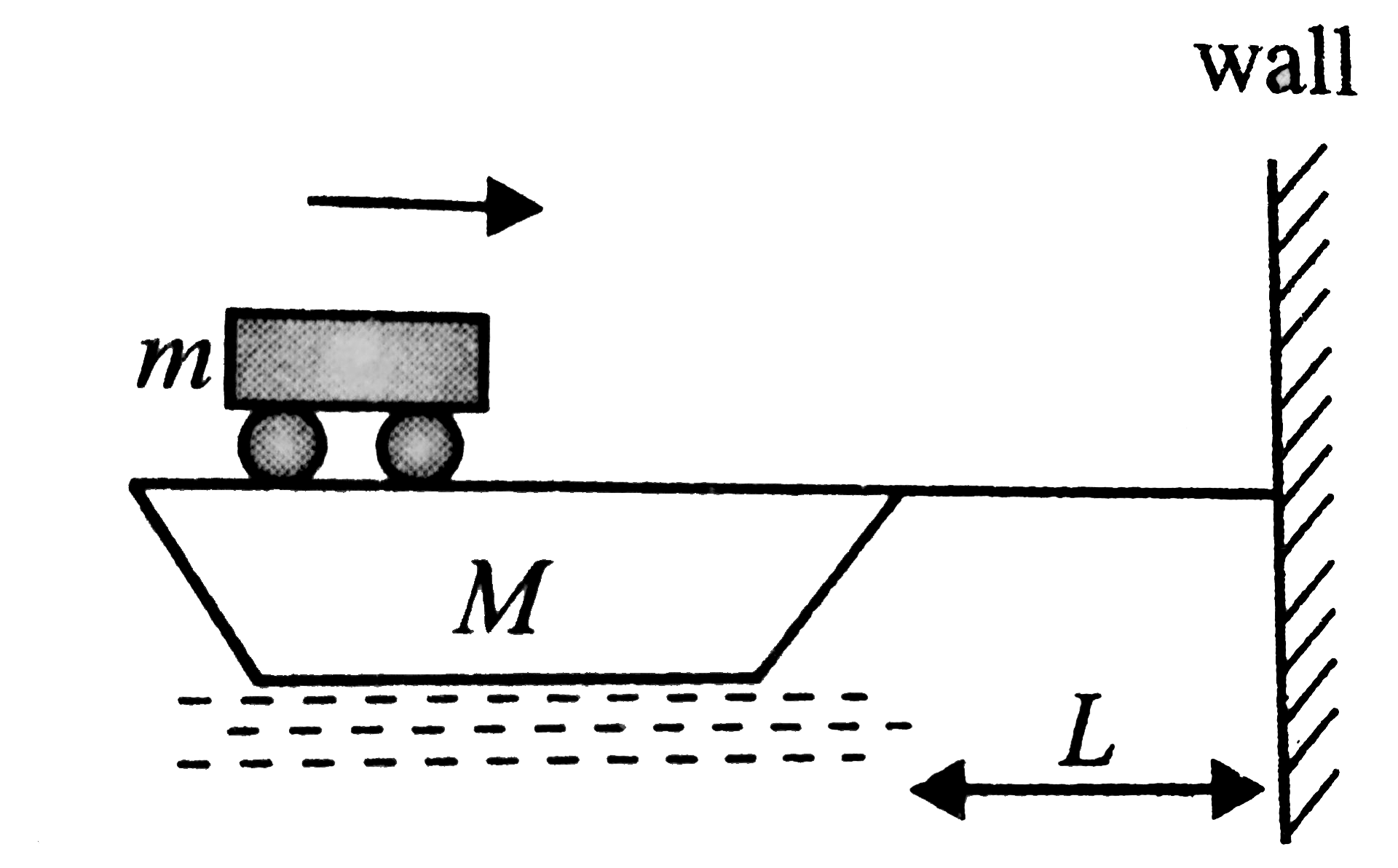
View SolutionA car of mass m is initially at rest on the boat of mass M tied to the wall of dock through a massless, inextensible string. The car accelerates from rest to velocity v0 in times to. At t=t0 the car applies brake and comes to rest relative to the boat in negligible time. Neglect friction between the boat and water: the time 't' at which boat will strike the wall is
View SolutionWhat is the power of an engine which can lift 600 kg of water per minute from 20 m deep well?
View SolutionA self-propelled vehicle of mass m, whose engine delivers a constant power P, has an acceleration a=(P/mv). (Assume that there is no friction). In order to increase its velocity from v1 to v2, the distan~e it has to travel will be:
View SolutionIn a car race, car A takes time t less than car B and passes the finishing point with a velocity of 12m/s more than the velocity with which car B passes the finishing point. Assume that the cars A and B start from rest and travel with constant acceleration of 9m/s2and 4m/s2 , respectively. If vA and vB be the velocities of cars A and B, respectively, then
View SolutionA body of mass 2 kg is driven by an engine delivering a constant power of 1 J /s. The body starts from rest and moves in a straight line. After 9 seconds, the body has moved a distance ( in m) ……….. .
View Solution
