A ball of mass M moving with speed v collides perfectly inelastically with another ball of mass m at rest. The magnitude of impulse imparted to the first ball is
Mv
mv
MmM+mv
M2M+mv
The correct Answer is:C
To solve the problem of finding the magnitude of impulse imparted to the first ball after a perfectly inelastic collision, we can follow these steps:
1. Identify the Initial Conditions:
- Mass of the first ball (moving) = M
- Speed of the first ball = v
- Mass of the second ball (at rest) = m
- Speed of the second ball = 0
2. Calculate Initial Momentum:
- The initial momentum of the system is given by the momentum of the first ball since the second ball is at rest.
Initial Momentum=M⋅v+m⋅0=Mv
3. Understand the Nature of Collision:
- Since the collision is perfectly inelastic, the two balls stick together after the collision.
4. Calculate Final Momentum:
- After the collision, the combined mass of the two balls is M+m.
- Let the final velocity of the combined mass be v′.
- By conservation of momentum:
Initial Momentum=Final Momentum
Mv=(M+m)v′
5. Solve for Final Velocity v′:
v′=MvM+m
6. Calculate Change in Momentum:
- The change in momentum for the first ball is given by:
Change in Momentum=Final Momentum−Initial Momentum
- The final momentum of the first ball after the collision is:
Final Momentum of first ball=M⋅v′
- Thus,
Change in Momentum=M⋅v′−M⋅v
- Substituting v′:
Change in Momentum=M(MvM+m)−Mv
7. Simplify the Change in Momentum:
=M2vM+m−Mv(M+m)M+m
=M2v−Mv(M+m)M+m
=M2v−M2v−MmvM+m
=−MmvM+m
8. Magnitude of Impulse:
- Impulse is equal to the change in momentum, hence:
Impulse=∣∣∣−MmvM+m∣∣∣=MmvM+m
Final Answer:
The magnitude of impulse imparted to the first ball is:
Impulse=M⋅m⋅vM+m
- Mass of the first ball (moving) = M
- Speed of the first ball = v
- Mass of the second ball (at rest) = m
- Speed of the second ball = 0
- The initial momentum of the system is given by the momentum of the first ball since the second ball is at rest.
Initial Momentum=M⋅v+m⋅0=Mv
- Since the collision is perfectly inelastic, the two balls stick together after the collision.
- After the collision, the combined mass of the two balls is M+m.
- Let the final velocity of the combined mass be v′.
- By conservation of momentum:
Initial Momentum=Final Momentum
Mv=(M+m)v′
v′=MvM+m
- The change in momentum for the first ball is given by:
Change in Momentum=Final Momentum−Initial Momentum
- The final momentum of the first ball after the collision is:
Final Momentum of first ball=M⋅v′
- Thus,
Change in Momentum=M⋅v′−M⋅v
- Substituting v′:
Change in Momentum=M(MvM+m)−Mv
=M2vM+m−Mv(M+m)M+m
=M2v−Mv(M+m)M+m
=M2v−M2v−MmvM+m
=−MmvM+m
- Impulse is equal to the change in momentum, hence:
Impulse=∣∣∣−MmvM+m∣∣∣=MmvM+m
The magnitude of impulse imparted to the first ball is:
Impulse=M⋅m⋅vM+m
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - B)
35 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - C)
80 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - EXERCISE
37 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
A particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 3 m at rest. Loss of KE of system in the collision is
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View SolutionA small ball of mass m moving with speed v collides elastically with a simple pendulum with bob of mass m at rest. The maximum height attained by the bob after collision is
View SolutionAn object of mass m1 moving with speed v collides with another object of mass m2 at rest and stick to it. Find the impulse imparted to the second object.
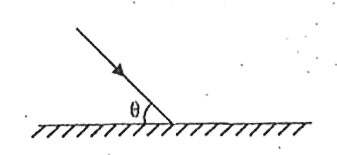
View SolutionA ball of mass m moving with speed u collides with a smooth horizontal surface at angle θ with it as shown in figure. The magnitude of impulse imparted to surface by ball is [Coefficient of restitution of collision is e]
View SolutionA ball of mass m moving at a speed v collides with another ball of mass 3m at rest. The lighter block comes to rest after collisoin. The coefficient of restitution is
View SolutionA ball of mass m moving with velocity v collides head-on which the second ball of mass m at rest. I the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then
View SolutionA ball of mass 4 kg moving on a smooth horizontal surface makes an elastic collision with another ball of mass m at rest in the line of motion of first ball. If after collision first ball moves in the same direction with one fourth of its velocity before collision, then mass of second ball is
View SolutionA spherical ball of mass M moving with initial velocity V collides elastically with another ball of mass M, which is fixed at one end of L shaped rigid massless frame as shown in Fig. (a). The L shaped frame contains another mass M connected at the other end.
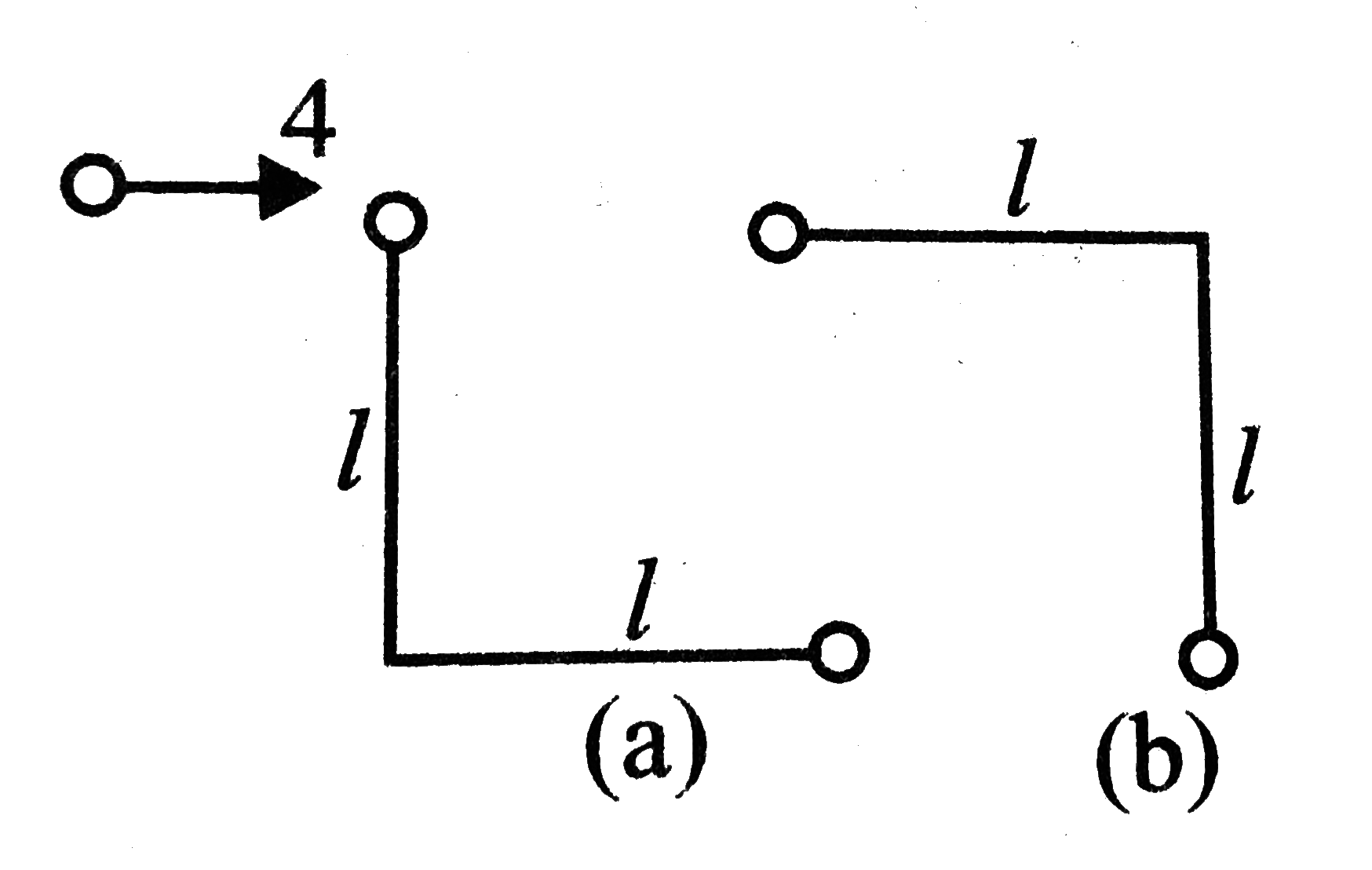
The speed of the striking mass after collision is
View Solution
