An infinite number of electric charges each equal to 5 nano coulombs are placed along x-axis at x = 1 cm, x-2 cm, x -4 cm, x = 8 cm,.. and so on. In this setup, if the consecutive charges have opposite sign, then the electric field in newton/coulomb at x =0 is
Infinity
zero
The correct Answer is:C
To solve the problem of finding the electric field at x=0 due to an infinite series of alternating charges placed along the x-axis, we can follow these steps:
1. Identify the Charge Configuration:
The charges are placed at positions x=1cm,2cm,4cm,8cm,… which are powers of 2. The charges alternate in sign: the charge at x=1cm is +5nC, at x=2cm is −5nC, at x=4cm is +5nC, and so on.
2. Electric Field Due to a Point Charge:
The electric field E due to a point charge Q at a distance r is given by:
E=14πϵ0Qr2
where ϵ0 is the permittivity of free space.
3. Calculate the Electric Field Contributions:
We will denote the electric field contributions from the charges at positions x=1,2,4,8,… respectively as E1,E2,E3,….
- For x=1cm:
E1=14πϵ05×10−9(0.01)2=5×10−94πϵ0×10−4
- For x=2cm:
E2=14πϵ05×10−9(0.02)2=5×10−94πϵ0×4×10−4=5×10−916πϵ0×10−4
- For x=4cm:
E3=14πϵ05×10−9(0.04)2=5×10−94πϵ0×16×10−4=5×10−964πϵ0×10−4
- For x=8cm:
E4=14πϵ05×10−9(0.08)2=5×10−94πϵ0×64×10−4=5×10−9256πϵ0×10−4
4. Sum the Electric Field Contributions:
The total electric field at x=0 is the sum of the contributions from all charges:
E=E1−E2+E3−E4+…
Factoring out 5×10−94πϵ0×10−4:
E=5×10−94πϵ0×10−4(1−14+116−164+…)
5. Recognize the Series:
The series inside the parentheses is a geometric series with first term a=1 and common ratio r=−14:
S=a1−r=11+14=45
6. Calculate the Total Electric Field:
Substituting back into the equation for E:
E=5×10−94πϵ0×10−4⋅45=1πϵ0×10−4
7. Final Calculation:
Using ϵ0=8.85×10−12C2/N m2:
E=1π×8.85×10−12×10−4≈36×104N/C
Final Answer:
The electric field at x=0 is approximately 36×104N/C.
The charges are placed at positions x=1cm,2cm,4cm,8cm,… which are powers of 2. The charges alternate in sign: the charge at x=1cm is +5nC, at x=2cm is −5nC, at x=4cm is +5nC, and so on.
The electric field E due to a point charge Q at a distance r is given by:
E=14πϵ0Qr2
where ϵ0 is the permittivity of free space.
We will denote the electric field contributions from the charges at positions x=1,2,4,8,… respectively as E1,E2,E3,….
E1=14πϵ05×10−9(0.01)2=5×10−94πϵ0×10−4
E2=14πϵ05×10−9(0.02)2=5×10−94πϵ0×4×10−4=5×10−916πϵ0×10−4
E3=14πϵ05×10−9(0.04)2=5×10−94πϵ0×16×10−4=5×10−964πϵ0×10−4
E4=14πϵ05×10−9(0.08)2=5×10−94πϵ0×64×10−4=5×10−9256πϵ0×10−4
The total electric field at x=0 is the sum of the contributions from all charges:
E=E1−E2+E3−E4+…
E=5×10−94πϵ0×10−4(1−14+116−164+…)
The series inside the parentheses is a geometric series with first term a=1 and common ratio r=−14:
S=a1−r=11+14=45
Substituting back into the equation for E:
E=5×10−94πϵ0×10−4⋅45=1πϵ0×10−4
Using ϵ0=8.85×10−12C2/N m2:
E=1π×8.85×10−12×10−4≈36×104N/C
The electric field at x=0 is approximately 36×104N/C.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT SECTION - C
53 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT SECTION - D
9 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT SECTION - A
44 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions
25 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
15 Videos
Similar Questions
An infinite number of charges, each equal to Q=10μC are placed along the x-axis at x=1,3,9…..m. Calculate the magnitude of electric field at x=0 if the consecutive charge have opposite signs.
View SolutionAn infinite number of charges each equal to q, are placed along the X-axis at x=1,x=2,x=4,x=8,…….. and so on.
(i) find the electric field at a point x=0 due to this set up of charges.
(ii) What will be the electric field if the above setup, the consecutive charges have opposite signs.
View SolutionAn infinite number of charges, each of coulomb, are placed along x-axis at x=1m,3m,9m and so on. Calculate the electric field at the point x = 0 due to these charges if all the charges are of the same sign.
View SolutionAn infinite number of charges, each of magnitude q, are placed along x-axis at x = 1m, 2m, 4m, 8m, 16m and so on but the consecutive charges are of opposite sign starting with +q at x = 1m. A point charge q0 , kept at the origin, experiences a force of magnitude :
View SolutionAt y = 1 cm, y = 3 cm y = 9 cm, y = 27 cm … and so on , an infinite number of charges equal to 5C are placed. At x = 1 cm, x = 2 cm, x = 4 cm, x = 8 cm …. And so on, an infinite number of charges equal to - 5C are placed. Find the electirc potential at origin is volts . (K=14πε0)
View SolutionThree charges are placed along x-axis at x = - a x = 0 and x = a as shown in the figure. The potential energy of the system is
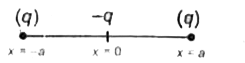
View SolutionThree charges are placed along x- axis at x=−a,x=0 and x=a as shown . Calculate the potential energy of the system.

View SolutionThe charges - q, Q, -q are placed along x-axis at x = 0, x = a and x = 2a. If the potential energy of the system is zero, then Q:q is
View SolutionAn infinite number of charges, each of charge 1μC are placed on the x-axis with co-ordinates x=1,2,4,8….∞ If a charge of 1C is kept at the origin, then what is the net force action on 1C charge
View SolutionAn infinite number of charges each q are placed in the x-axis at distances of 1, 2, 4, 8, ………meter from the origin. If the charges are alternately positive and negative find the intensity of electric field at origin.
View Solution
