An infinite number of charges each q are placed in the x-axis at distances of 1, 2, 4, 8, ………meter from the origin. If the charges are alternately positive and negative find the intensity of electric field at origin.
To find the intensity of the electric field at the origin due to an infinite number of charges placed alternately on the x-axis at distances of 1, 2, 4, 8, ..., we can follow these steps:
1. Identify the Charge Configuration:
- The charges are placed at distances of 1,2,4,8,… meters from the origin. This forms a geometric series where the distances are dn=2n−1 for n=1,2,3,….
- The charges are alternately positive and negative: +q,−q,+q,−q,….
2. Calculate the Electric Field Contribution from Each Charge:
- The electric field E due to a point charge q at a distance r is given by:
E=kqr2
- For the charge at distance 1 meter:
E1=kq12=kq
- For the charge at distance 2 meters:
E2=−kq22=−kq4
- For the charge at distance 4 meters:
E3=kq42=kq16
- For the charge at distance 8 meters:
E4=−kq82=−kq64
3. Sum the Electric Field Contributions:
- The total electric field at the origin E is the sum of the contributions from all the charges:
E=E1+E2+E3+E4+…
- This can be expressed as:
E=kq(1−14+116−164+…)
4. Recognize the Series:
- The series inside the parentheses is a geometric series with the first term a=1 and common ratio r=−14.
- The sum of an infinite geometric series is given by:
S=a1−r
- Applying this formula:
S=11−(−14)=11+14=154=45
5. Substitute Back to Find Total Electric Field:
- Thus, the total electric field at the origin becomes:
E=kq⋅45
6. Substitute the Value of k:
- The value of k is given by k=14πϵ0:
E=14πϵ0⋅q⋅45=q5πϵ0
Final Answer:
The intensity of the electric field at the origin is:
E=q5πϵ0
- The charges are placed at distances of 1,2,4,8,… meters from the origin. This forms a geometric series where the distances are dn=2n−1 for n=1,2,3,….
- The charges are alternately positive and negative: +q,−q,+q,−q,….
- The electric field E due to a point charge q at a distance r is given by:
E=kqr2
- For the charge at distance 1 meter:
E1=kq12=kq
- For the charge at distance 2 meters:
E2=−kq22=−kq4
- For the charge at distance 4 meters:
E3=kq42=kq16
- For the charge at distance 8 meters:
E4=−kq82=−kq64
- The total electric field at the origin E is the sum of the contributions from all the charges:
E=E1+E2+E3+E4+…
- This can be expressed as:
E=kq(1−14+116−164+…)
- The series inside the parentheses is a geometric series with the first term a=1 and common ratio r=−14.
- The sum of an infinite geometric series is given by:
S=a1−r
- Applying this formula:
S=11−(−14)=11+14=154=45
- Thus, the total electric field at the origin becomes:
E=kq⋅45
- The value of k is given by k=14πϵ0:
E=14πϵ0⋅q⋅45=q5πϵ0
The intensity of the electric field at the origin is:
E=q5πϵ0
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Assess Your Self13videos
ELECTRIC CHARGES AND FIELDS
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise-I80videos
ELECTRIC CHARGES AND FIELDS
- BOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX42videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)26videos
ELECTRIC FIELD AND POTENTIAL
Similar Questions
An infinite number of charges, each of charge 1μC are placed on the x-axis with co-ordinates x=1,2,4,8….∞ If a charge of 1C is kept at the origin, then what is the net force action on 1C charge
View SolutionInfinite number of bodies, each of mass 2kg, are situated on x-axis at distance 1m,2m,4m,8m......... respectively, from the origin. The resulting gravitational potential due to this system at the origin will be
View SolutionAn infinite number of particles each of mass m are placed on the positive X-axis of 1m,2m,4m,8m,... from the origin. Find the magnitude of the resultant gravitational force on mass m kept at the origin.
View SolutionInfinite number of bodies, each of mass 2kg, are situated on x-axis at distance 1m,2m,4m,8m......... respectively, from the origin. The resulting gravitational potential the to this system at the origing will be
View SolutionAn infinite number of charges, each of magnitude q, are placed along x-axis at x = 1m, 2m, 4m, 8m, 16m and so on but the consecutive charges are of opposite sign starting with +q at x = 1m. A point charge q0 , kept at the origin, experiences a force of magnitude :
View SolutionAn infinite number of charges each equal to q, are placed along the X-axis at x=1,x=2,x=4,x=8,…….. and so on.
(i) find the electric field at a point x=0 due to this set up of charges.
(ii) What will be the electric field if the above setup, the consecutive charges have opposite signs.View SolutionAn infinite number of charges, each equal to Q=10μC are placed along the x-axis at x=1,3,9…..m. Calculate the magnitude of electric field at x=0 if the consecutive charge have opposite signs.
View SolutionAn infinite number of charges, each of coulomb, are placed along x-axis at x=1m,3m,9m and so on. Calculate the electric field at the point x = 0 due to these charges if all the charges are of the same sign.
View Solution4 charges are placed each at a distance a from origin . The dipole moment of configuration is .
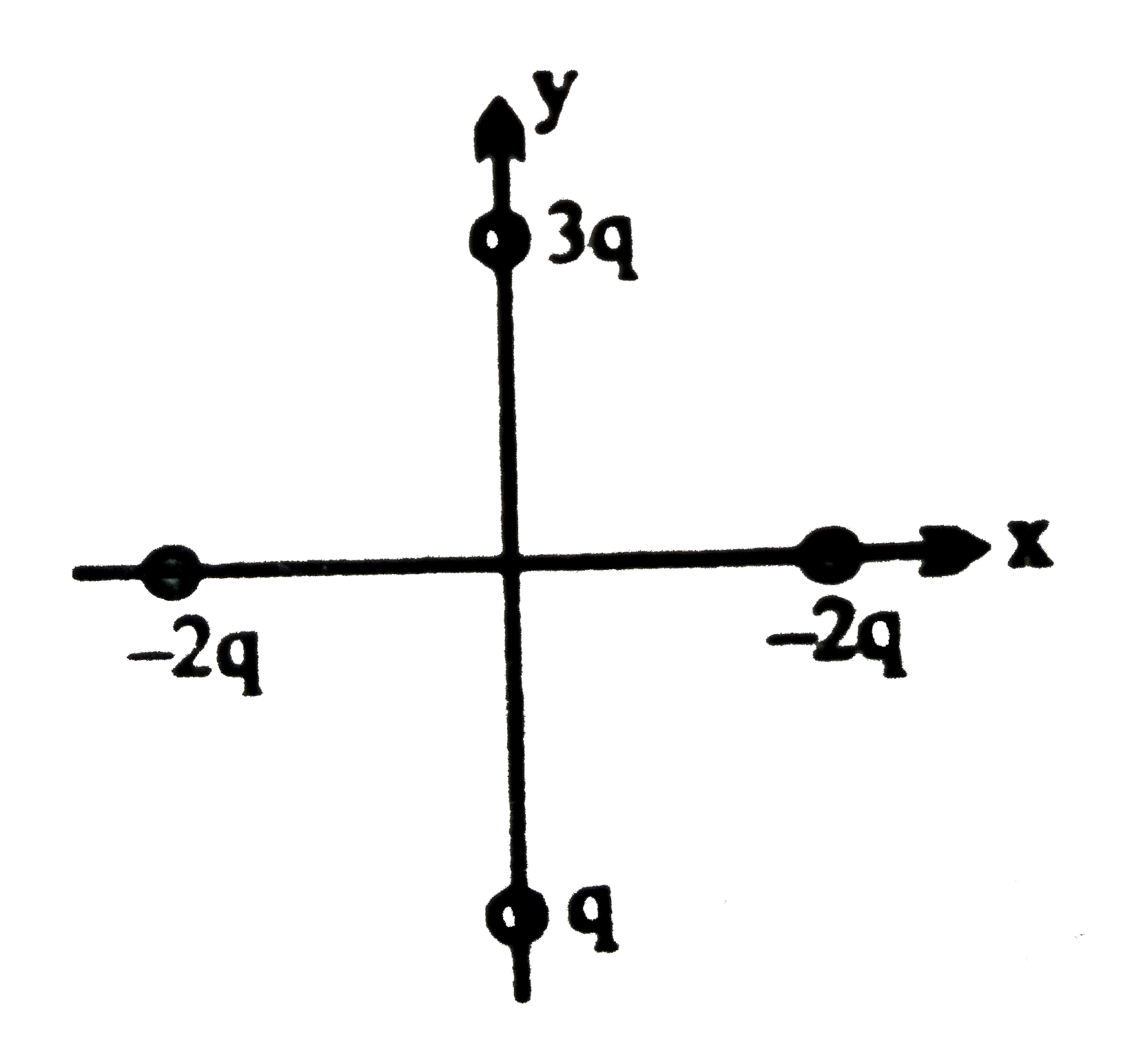 .View Solution
.View SolutionAn infinite number of electric charges each equal to 5 nano coulombs are placed along x-axis at x = 1 cm, x-2 cm, x -4 cm, x = 8 cm,.. and so on. In this setup, if the consecutive charges have opposite sign, then the electric field in newton/coulomb at x =0 is
View Solution

