A stone falls freely such that the distance covered by it in the last second of its motion is equal to the distance covered by it in the first 5 seconds. It remained in air for :-
16.5 s
17 s
15 s
13 s
The correct Answer is:D
To solve the problem, we need to find the time a stone remains in the air when the distance covered in the last second of its fall is equal to the distance covered in the first 5 seconds.
1. Understanding the Problem:
- Let the total time of fall be t seconds.
- The distance covered in the last second of motion (i.e., during the t-th second) is equal to the distance covered in the first 5 seconds.
2. Distance Covered in the Last Second:
- The distance covered in the t-th second can be calculated using the formula:
st=u+12g(2t−1)
- Since the stone is falling freely from rest, the initial velocity u=0:
st=12g(2t−1)
3. Distance Covered in the First 5 Seconds:
- The distance covered in the first 5 seconds can be calculated using the formula:
s5=u+12g(52)
- Again, since u=0:
s5=12g(25)=25g2
4. Setting the Distances Equal:
- According to the problem, the distance covered in the last second is equal to the distance covered in the first 5 seconds:
12g(2t−1)=25g2
5. Canceling Common Terms:
- We can cancel 12g from both sides (assuming g≠0):
2t−1=25
6. Solving for t:
- Rearranging the equation:
2t=25+1=26
t=262=13 seconds
Final Answer:
The stone remains in the air for 13 seconds.
- Let the total time of fall be t seconds.
- The distance covered in the last second of motion (i.e., during the t-th second) is equal to the distance covered in the first 5 seconds.
- The distance covered in the t-th second can be calculated using the formula:
st=u+12g(2t−1)
- Since the stone is falling freely from rest, the initial velocity u=0:
st=12g(2t−1)
- The distance covered in the first 5 seconds can be calculated using the formula:
s5=u+12g(52)
- Again, since u=0:
s5=12g(25)=25g2
- According to the problem, the distance covered in the last second is equal to the distance covered in the first 5 seconds:
12g(2t−1)=25g2
- We can cancel 12g from both sides (assuming g≠0):
2t−1=25
- Rearranging the equation:
2t=25+1=26
t=262=13 seconds
The stone remains in the air for 13 seconds.
Topper's Solved these Questions
Similar Questions
A stone falls freely from rest and the total distance covered by it in last second of its motion equals the distance covered by it in the first 3s of its motion. How long the stone will remain in the air?
View SolutionA stone falls freely rest. The distance covered by it in the last second is equal to the distance covered by it in the first 2 s. The time taken by the stone to reach the ground is
View Solution
Knowledge Check
A body falls freely form rest. It covers as much distance in the last second of its motion as covered in the first three seconds. The body has fallen for a time of
A3 sB5 sC7 sD9s
A ball is dropped from the roof of a tower height h. The total distance covered by it in the last second of its motion is equal to the distance covered by it in first three seconds. The value of h in metre is (g=10m/s2)
View SolutionA ball is dropped from the roof of a tower height h. The total distance covered by it in the last second of its motion is equal to the distance covered by it in first three seconds. The value of h in metre is (g=10m/s2)
View SolutionFor the velocity - time graph shown in figure below the distance covered by the body in last two seconds of its motion is what fraction of the total distance covered by it in all the total distance covered by it in all the seven seconds
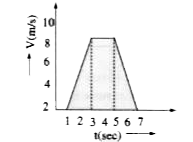
View SolutionIf the distance travelled by a freely falling body in the last second of its journey is equal to the distance travelled in the first 2s, the time of descent of the body is
View SolutionA ball dropped from the top of a tower covers a distance 7x in the last second of its journey, where x is the distance covered in the first second. How much time does it take to reach to ground?.
View SolutionA body dropped from the top of tower covers a distance 9 x in the last second of its journey, where x is the distance covered in the first second. How much time does it take to reach the ground.
View SolutionA bolldropped from the top of a tower covers a distance 7x in the last second of its journey, where x is the distance coverd int the first second. How much time does it take to reach to ground?.
View Solution
