There are two concentric conducting spherical shells of radius rA and rB respectively (rB=2rA). If the inner shell is given a charge Q and outer shell is given a charge 2Q, then the potential difference between the surfaces of the shells will be K=14πε0
kQ2rA
kQrA
kQ2rB
kQ4rB
To solve the problem of finding the potential difference between the surfaces of two concentric conducting spherical shells, we will follow these steps:
1. Identify the Given Information:
- Inner shell radius: rA
- Outer shell radius: rB=2rA
- Charge on the inner shell: Q
- Charge on the outer shell: 2Q
2. Calculate the Potential at the Inner Shell (VA):
The potential VA at the surface of the inner shell due to its own charge Q is given by:
VA=KQrA
where K=14πϵ0.
3. Calculate the Potential at the Outer Shell (VB):
The potential VB at the surface of the outer shell due to the charge 2Q on it is given by:
VB=K(2Q)rB
Substituting rB=2rA:
VB=K(2Q)2rA=KQrA
4. Calculate the Potential Difference (VAB):
The potential difference VAB between the inner and outer shells is given by:
VAB=VA−VB
Substituting the expressions for VA and VB:
VAB=KQrA−KQ2rA
Simplifying this expression:
VAB=KQrA−KQ2rA=KQrA(1−12)=KQrA⋅12=KQ2rA
5. Final Result:
The potential difference between the surfaces of the shells is:
VAB=KQ2rA
Conclusion:
The potential difference between the surfaces of the shells is KQ2rA.
- Inner shell radius: rA
- Outer shell radius: rB=2rA
- Charge on the inner shell: Q
- Charge on the outer shell: 2Q
The potential VA at the surface of the inner shell due to its own charge Q is given by:
VA=KQrA
where K=14πϵ0.
The potential VB at the surface of the outer shell due to the charge 2Q on it is given by:
VB=K(2Q)rB
Substituting rB=2rA:
VB=K(2Q)2rA=KQrA
The potential difference VAB between the inner and outer shells is given by:
VAB=VA−VB
Substituting the expressions for VA and VB:
VAB=KQrA−KQ2rA
Simplifying this expression:
VAB=KQrA−KQ2rA=KQrA(1−12)=KQrA⋅12=KQ2rA
The potential difference between the surfaces of the shells is:
VAB=KQ2rA
The potential difference between the surfaces of the shells is KQ2rA.
Topper's Solved these Questions
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Try Yourself
63 Videos
- TEST 2BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 2EXERCISE - Exercise
308 Videos
Similar Questions
There are two concentric conducting spherical shells of radii 10cm and 20cm. The outer shell is charged to a potential of 50esu while inner shell is earthed. The charge on outer shell is
View SolutionConsider two concentric spherical metal shells of radii r1 and r2(r2>r1). If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
View SolutionFour concentric conducting spherical shells A, B, C and D of radii a, 2a, 3a and 4a respectively. Shell B and D are given charges q and −q respectively. If shell C is grounded then potential difference (VA — VC) is
View SolutionThere are two concentric spherical shell of radii r and 2r. Initially, a charge Q is given to the inner shell and both the switches are open.
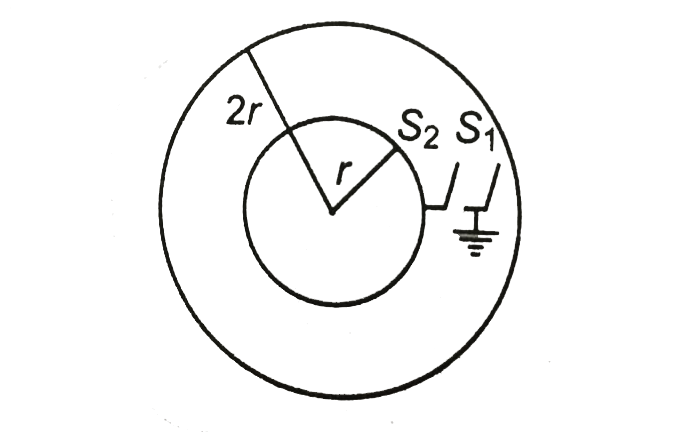
The two steps of the above two problems are repeated b times, the potential differences between the shells will be
View Solutionthere are two concentric metallic shells of radii R and 2R, if outer shell is given charge q1 , then charge on surface of inner shell will be
View SolutionThere are two concentric hollow conducting spherical shells of radii r and R(R>r). The charge on the outer shell is Q. What charge should be given to the inner shell, so that the potential at a point P , at a distance 2R from the common centre is zero ?
View SolutionTwo concentric conducting shells of radii R and 2R are having charges Q and -2Q respectively.In a region r
View SolutionA and C are concentric conducting spherical shells of radius a and c respectively.A is sueeounded by a concentric dielectric medium of inner radius a, outer radius b and dielectric constant k. If sphere A is given a charge Q, the potential at the outer surface of the dielectric is

View SolutionA conducting shell S1 having a charge Q is surrounded by an uncharged concentric conducting spherical shell S2. Let the potential difference between S1 and that S2 be V. If the shell S2 is now given a charge −3Q, the new potential difference between the same two shells is
View SolutionThere are two thin concentric conducting shells of radii a and b(a<b) . Equal charges Q given to each shell. Find the charge distribution on the inner and outer shell.
View Solution