A conductor having cubold shape has dimensions in ratio 1: 2:5, the ratio of maximum to minimum resistance across two opposite faces is
A. 0
B. 5:1
C. 25:1
D. 10:1
The correct Answer is:C
To solve the problem of finding the ratio of maximum to minimum resistance across two opposite faces of a cuboidal conductor with dimensions in the ratio 1:2:5, we can follow these steps:
Step 1: Define the Dimensions
Let the dimensions of the cuboid be:
- Length (L) = 1x
- Breadth (B) = 2x
- Height (H) = 5x
Step 2: Identify the Faces
The cuboid has three pairs of opposite faces:
1. Faces with dimensions (Length x Height) = (1x x 5x)
2. Faces with dimensions (Breadth x Height) = (2x x 5x)
3. Faces with dimensions (Length x Breadth) = (1x x 2x)
Step 3: Calculate Minimum Resistance
The minimum resistance will be across the faces with the smallest area. The area of the faces with dimensions (1x x 2x) is:
Amin=1x×2x=2x2
Using the formula for resistance:
R=ρLA
Where:
- ρ = resistivity of the material
- L = thickness of the conductor (which we take as the other dimension, here it will be 5x)
Thus, the minimum resistance Rmin is:
Rmin=ρ(5x)2x2=5ρ2x
Step 4: Calculate Maximum Resistance
The maximum resistance will be across the faces with the largest area. The area of the faces with dimensions (2x x 5x) is:
Amax=2x×5x=10x2
Thus, the maximum resistance Rmax is:
Rmax=ρ(1x)10x2=ρ10x
Step 5: Calculate the Ratio of Maximum to Minimum Resistance
Now, we can find the ratio of maximum resistance to minimum resistance:
RmaxRmin=ρ10x5ρ2x=ρ10x×2x5ρ
The ρ and x cancel out:
RmaxRmin=250=125
Thus, the ratio of maximum to minimum resistance is:
Rmax:Rmin=25:1
Final Answer
The ratio of maximum to minimum resistance across two opposite faces is 25:1.
---
Let the dimensions of the cuboid be:
- Length (L) = 1x
- Breadth (B) = 2x
- Height (H) = 5x
The cuboid has three pairs of opposite faces:
1. Faces with dimensions (Length x Height) = (1x x 5x)
2. Faces with dimensions (Breadth x Height) = (2x x 5x)
3. Faces with dimensions (Length x Breadth) = (1x x 2x)
The minimum resistance will be across the faces with the smallest area. The area of the faces with dimensions (1x x 2x) is:
Amin=1x×2x=2x2
R=ρLA
Where:
- ρ = resistivity of the material
- L = thickness of the conductor (which we take as the other dimension, here it will be 5x)
Rmin=ρ(5x)2x2=5ρ2x
The maximum resistance will be across the faces with the largest area. The area of the faces with dimensions (2x x 5x) is:
Amax=2x×5x=10x2
Rmax=ρ(1x)10x2=ρ10x
Now, we can find the ratio of maximum resistance to minimum resistance:
RmaxRmin=ρ10x5ρ2x=ρ10x×2x5ρ
RmaxRmin=250=125
Rmax:Rmin=25:1
The ratio of maximum to minimum resistance across two opposite faces is 25:1.
Topper's Solved these Questions
Similar Questions
In YDSE ratio of width of slit is 4:1, then ratio of maximum to minimum intensity
View SolutionIn YDSE ratio of width of slit is 4:1, then ratio of maximum to minimum intensity
View SolutionIf two light waves having same frequency have intensity ratio 4:1 and they interfere, the ratio of maximum to minimum intensity in the pattern will be
View SolutionAll the edges of a block with parallel faces are unequal. Its longest edge is twice its shortest edge. The ratio of the maximum to minimum resistance between parallel faces is.
View SolutionRatio of amplitude for two wave is 1:5.Find the ratio of maximum Intensity to minimum intensity.
View SolutionIf the ratio of amplitude of two waves is 4:3, then the ratio of maximum and minimum intensity is
View SolutionRatio of amplitude for two wave is 1:3 .Find the ratio of maximum intensity to minimum intensity.
View SolutionRatio of amplitude for two wave is 1:4 .Find the ratio of maximum intensity to minimum intensity.
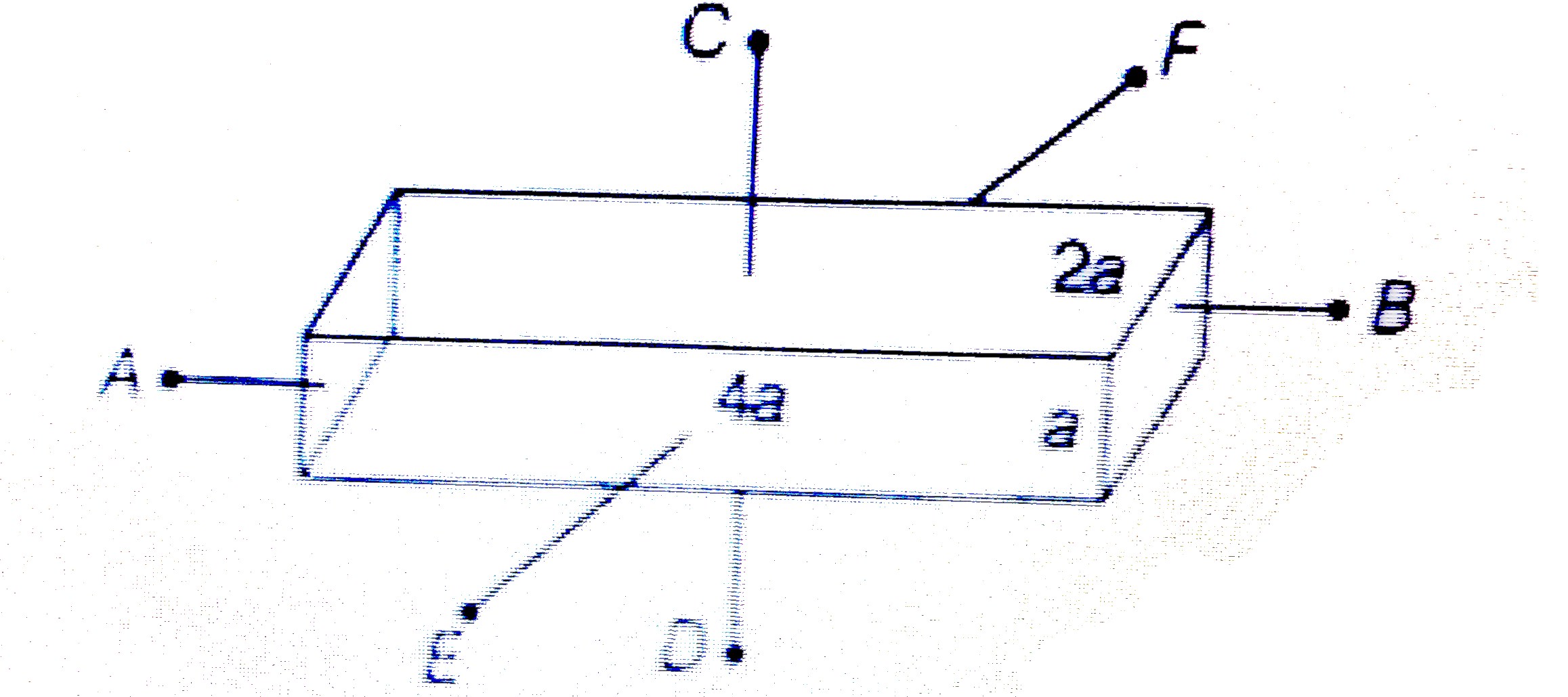
View SolutionA conductor with rectangular cross section has dimensions (a×2a×4a) as shown in figure. Resistance across AB is x, across CD is y and across EF is z. Then
View Solutionn equal resistors are first connected in series and then connected in parallel. What is the ratio of the maximum to the minimum resistance ?
View Solution