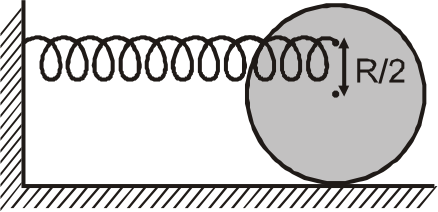
A spring of force constant k is connected with centre of a disc of mass m and radius R System is placed on honzontal surface and other end of spring is fixed with a vertical wall as shown in a figure. If there is sufficient friction for pure rolling then time period of oscillation of the disc will be
2π[√2m3k]
π[√2m3k]
2π[√3m2k]
π[√3m2k]
To find the time period of oscillation of the disc connected to a spring, we can follow these steps:
Step 1: Understand the System
We have a disc of mass m and radius R that is connected to a spring with force constant k. The other end of the spring is fixed to a wall. The system is on a horizontal surface, and there is sufficient friction for pure rolling.
Step 2: Identify Forces and Torques
When the disc is displaced by a distance x, the spring exerts a restoring force Fs=−kx. The frictional force Ff provides the necessary torque for rolling without slipping. The net force acting on the disc can be expressed as:
F=ma=kx−Ff
Step 3: Relate Torque and Angular Acceleration
The torque τ about the center of the disc due to the frictional force is given by:
τ=Ff⋅R
For a disc, the moment of inertia I about its center is 12mR2. The angular acceleration α is related to the linear acceleration a by:
α=aR
Thus, we can write:
τ=I⋅α=12mR2⋅aR=12mRa
Step 4: Set Up the Equations
From the torque equation, we can express the frictional force:
Ff=ma2R
Now substituting Ff back into the force equation:
ma=kx−ma2
Rearranging gives:
ma+ma2=kx
3ma2=kx
Step 5: Solve for Acceleration
From the above equation, we can solve for acceleration a:
a=2kx3m
Step 6: Relate Acceleration to Angular Frequency
The acceleration can also be expressed in terms of angular frequency ω2:
a=−ω2x
Equating the two expressions for a:
−ω2x=2kx3m
This implies:
ω2=2k3m
Step 7: Find the Time Period
The time period T of oscillation is related to the angular frequency ω by:
T=2πω
Substituting for ω:
T=2π√3m2k
Final Answer
Thus, the time period of oscillation of the disc is:
T=2π√3m2k
We have a disc of mass m and radius R that is connected to a spring with force constant k. The other end of the spring is fixed to a wall. The system is on a horizontal surface, and there is sufficient friction for pure rolling.
When the disc is displaced by a distance x, the spring exerts a restoring force Fs=−kx. The frictional force Ff provides the necessary torque for rolling without slipping. The net force acting on the disc can be expressed as:
F=ma=kx−Ff
The torque τ about the center of the disc due to the frictional force is given by:
τ=Ff⋅R
For a disc, the moment of inertia I about its center is 12mR2. The angular acceleration α is related to the linear acceleration a by:
α=aR
Thus, we can write:
τ=I⋅α=12mR2⋅aR=12mRa
From the torque equation, we can express the frictional force:
Ff=ma2R
Now substituting Ff back into the force equation:
ma=kx−ma2
Rearranging gives:
ma+ma2=kx
3ma2=kx
From the above equation, we can solve for acceleration a:
a=2kx3m
The acceleration can also be expressed in terms of angular frequency ω2:
a=−ω2x
Equating the two expressions for a:
−ω2x=2kx3m
This implies:
ω2=2k3m
The time period T of oscillation is related to the angular frequency ω by:
T=2πω
Substituting for ω:
T=2π√3m2k
Thus, the time period of oscillation of the disc is:
T=2π√3m2k
Topper's Solved these Questions
Similar Questions
A uniform disc of mass m is attached to a spring of spring constant k as shown in figure and there is sufficient friction to prevent slipping of disc. Time period of small oscillations of disc is:
View SolutionA disc of mass M and radius R rolls on a horizontal surface and then rolls up an inclined plane as shown in the figure. If the velocity of the disc is v, the height to which the disc will rise will be:

View Solution
Knowledge Check
A system of springs with their spring constants are as shown in figure. What is the frequency of oscillations of the mass m ?
 A12π√k1k2(k3+k4)[(k1+k2)+(k3+k4)+k1k4]mB12π√k1k2(k3+k4)[(k1+k2)+(k3+k4)+k1k2]mC12π√k1k2(k3+k4)[(k1+k2)+(k3+k4)+k1k2]mD12π√(k1+k2)(k3+k4)+k1k2k1k2(k3+k4)m
A12π√k1k2(k3+k4)[(k1+k2)+(k3+k4)+k1k4]mB12π√k1k2(k3+k4)[(k1+k2)+(k3+k4)+k1k2]mC12π√k1k2(k3+k4)[(k1+k2)+(k3+k4)+k1k2]mD12π√(k1+k2)(k3+k4)+k1k2k1k2(k3+k4)m
One end of spring of spring constant k is attached to the centre of a disc of mass m and radius R and the other end of the spring connected to a rigid wall. A string is wrapped on the disc and the end A of the string (as shown in the figure) is pulled through a distance a and then released.
The disc is placed on a horizontal rough surface and there is no slipping at any contact point. What is the amplitude of the oscillation of the centre of the disc?

View SolutionA point mass m collides with a disc of mass m and radius R resting on a rough horizontal surface as shown . Its collision is perfectly elastic. Find angular velocity of the disc after pure rolling starts

View SolutionA body of mass m is suspended from three springs as shown in figure. If mass m is displaced slightly then time period of oscillation is

View SolutionA uniform disc of mass m & radius R is pivoted at its centre O with its plane vertical as shown in figure A circular portion of disc of radius R2 is removed from it. The time period of small oscillations of remaining portion about O is -

View SolutionSpring mass system is shown in figure. find the time period of vertical oscillations. The system is in vertical plane.

View SolutionA force F is applied at the centre of a disc of mass M. The minimum value of coefficient of friction of the surface for rolling is
View SolutionA particle of mass m is attached with three springs A,B and C of equal force constancts k as shown in figure. The particle is pushed slightly against the spring C and released. Find the time period of oscillation.
 .
.
View Solution