Show by a diagram how two resistors R1andR2 are joined in parallel. Obtain an expression for the total resistance of the combination.
To solve the problem of showing how two resistors R1 and R2 are joined in parallel and obtaining an expression for the total resistance of the combination, we can follow these steps:
Step 1: Draw the Circuit Diagram
Draw a circuit diagram showing two resistors \( R_1 \) and \( R_2 \) connected in parallel. The arrangement should look like this:
±−−−−R1−−−−−+||∣||∣±−−−−R2−−−−−+
In the diagram, both resistors are connected to the same two points, indicating that they share the same voltage across them.
Step 2: Understand the Current Flow
Let:
- \( I \) be the total current flowing from the source.
- \( I_1 \) be the current flowing through resistor \( R_1 \).
- \( I_2 \) be the current flowing through resistor \( R_2 \).
Since the resistors are in parallel, the total current \( I \) is the sum of the currents through each resistor:
\(
I = I_1 + I_2
\)
Step 3: Apply Ohm's Law
According to Ohm's Law, the voltage across each resistor is the same and is equal to \( V \) (the voltage of the battery). Thus, we can write:
\(
V = I_1 R_1 \quad \text{(for resistor } R_1\text{)}
\)
\(
V = I_2 R_2 \quad \text{(for resistor } R_2\text{)}
\)
From these equations, we can express \( I_1 \) and \( I_2 \) in terms of \( V \):
\(
I_1 = \frac{V}{R_1}
\)
\(
I_2 = \frac{V}{R_2}
\)
Step 4: Substitute into the Total Current Equation
Substituting the expressions for \( I_1 \) and \( I_2 \) into the total current equation gives:
\(
I = \frac{V}{R_1} + \frac{V}{R_2}
\)
Step 5: Factor Out the Voltage
Factoring out \( V \) from the right side, we have:
\(
I = V \left( \frac{1}{R_1} + \frac{1}{R_2} \right)
\)
Step 6: Relate Total Current to Total Resistance
By Ohm's Law, we also know that:
\(
I = \frac{V}{R}
\)
where \( R \) is the equivalent resistance of the parallel combination. Setting the two expressions for \( I \) equal to each other gives:
\(
\frac{V}{R} = V \left( \frac{1}{R_1} + \frac{1}{R_2} \right)
\)
Step 7: Cancel Voltage and Solve for Total Resistance
Assuming \( V \neq 0 \), we can cancel \( V \) from both sides:
\(
\frac{1}{R} = \frac{1}{R_1} + \frac{1}{R_2}
\)
Step 8: Final Expression for Total Resistance
Taking the reciprocal of both sides gives us the final expression for the total resistance \( R \) of two resistors in parallel:
\(
R = \frac{1}{\frac{1}{R_1} + \frac{1}{R_2}}
\)
Summary
The total resistance \( R \) for two resistors \( R_1 \) and \( R_2 \) connected in parallel is given by:
\(
\frac{1}{R} = \frac{1}{R_1} + \frac{1}{R_2}
\)
Draw a circuit diagram showing two resistors \( R_1 \) and \( R_2 \) connected in parallel. The arrangement should look like this:
In the diagram, both resistors are connected to the same two points, indicating that they share the same voltage across them.
Let:
- \( I \) be the total current flowing from the source.
- \( I_1 \) be the current flowing through resistor \( R_1 \).
- \( I_2 \) be the current flowing through resistor \( R_2 \).
\(
I = I_1 + I_2
\)
According to Ohm's Law, the voltage across each resistor is the same and is equal to \( V \) (the voltage of the battery). Thus, we can write:
\(
V = I_1 R_1 \quad \text{(for resistor } R_1\text{)}
\)
\(
V = I_2 R_2 \quad \text{(for resistor } R_2\text{)}
\)
\(
I_1 = \frac{V}{R_1}
\)
\(
I_2 = \frac{V}{R_2}
\)
Substituting the expressions for \( I_1 \) and \( I_2 \) into the total current equation gives:
\(
I = \frac{V}{R_1} + \frac{V}{R_2}
\)
Factoring out \( V \) from the right side, we have:
\(
I = V \left( \frac{1}{R_1} + \frac{1}{R_2} \right)
\)
By Ohm's Law, we also know that:
\(
I = \frac{V}{R}
\)
where \( R \) is the equivalent resistance of the parallel combination. Setting the two expressions for \( I \) equal to each other gives:
\(
\frac{V}{R} = V \left( \frac{1}{R_1} + \frac{1}{R_2} \right)
\)
Assuming \( V \neq 0 \), we can cancel \( V \) from both sides:
\(
\frac{1}{R} = \frac{1}{R_1} + \frac{1}{R_2}
\)
Taking the reciprocal of both sides gives us the final expression for the total resistance \( R \) of two resistors in parallel:
\(
R = \frac{1}{\frac{1}{R_1} + \frac{1}{R_2}}
\)
The total resistance \( R \) for two resistors \( R_1 \) and \( R_2 \) connected in parallel is given by:
\(
\frac{1}{R} = \frac{1}{R_1} + \frac{1}{R_2}
\)
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(B) (MULTIPLE CHOICE TYPE)
3 Videos
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(B) (NUMERICALS )
54 Videos
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(A)(NUMERICALS )
16 Videos
- CALORIMETRYBOOK - ICSECHAPTER - CALORIMETRYEXERCISE - EXERCISE-11(B) (NUMERICALS)
15 Videos
- ELECTRO-MAGNETISM BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS
1 Videos
Similar Questions
Two cells of emfs ε1andε2 and internal resistances r1andr2 respectively are connected in parallel . Obtain expressions for the equivalent (i) resistance and , (ii) emf of the combination.
View Solution(a) Resistors given as R1,R2andR3 are connected in series to a battery V. Draw the circuit diagram showing the arrangement. Derive an expression for the equivalent resistance of the combination.
(b) If R1=10Ω,R2=20ΩandR3=30Ω, calculate the effective resistance when they are connected in series to a battery of 6 V. Also find the current flowing in the circuit.
View Solution(a) Three resistors 2Ω,4Ωand5Ω are combined in parallel. What is the total resistance of the combination ?
(b) If the combination is connected to a battery of emf 20 V and negligible internal resistance, determine the current through each resistor, and the total current drawn from the battery.
View SolutionTwo resistances R and 2R are connected in parallel in an electric circuit. The thermal energy developed in R and 2R are in the ratio
View SolutionFor two resistors R1 and R2, connected in parallel, find the relative error in their equivalent resistance. if R1=(50±2)Ω and R2=(100±3)Ω.
View Solution(a) Three resistors 1Ω,2Ω and 3Ω are combined in series. What is the total resistance of the combination ?
(b) If the combination is connected to a battery of emf 12 V and negligible internal resistance, obtain the potential drop across each resistor.
View Solution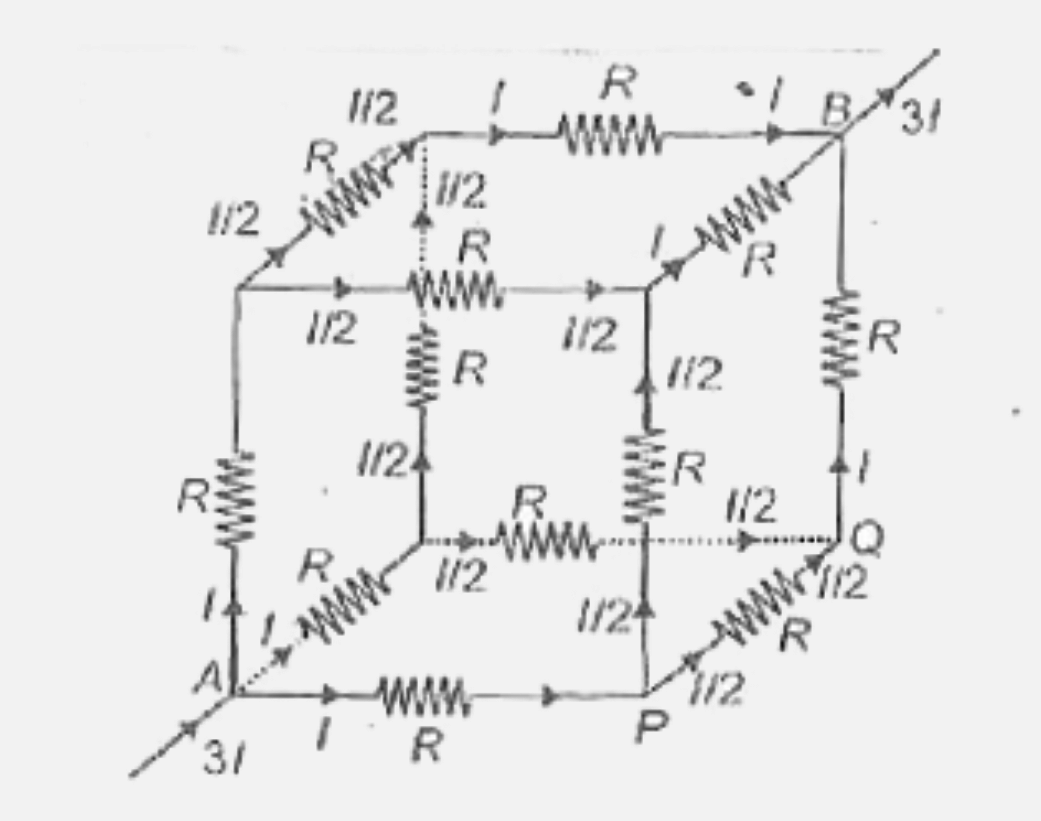
Twelve indentical resistance each of resistance R are joined to form a cube as shown in figure. Find resistance of the combination across AB.
View SolutionThe resistance of the series combination of two resistances is S. When they are joined in parallel the total resistance is P. If S= nP then the minimum possible value of n is
View SolutionThe resistance of the series combination of two resistances is S. When they are joined in parallel the total resistance is P. If S= nP then the minimum possible value of n is
View SolutionTwo cells of equal e.m.f. E b ut of difference internal resistance r1 and r2 are connected in parallel and the combination is connected with an external resistance R to obtain maximum power in R. find the value of R

View Solution
