ABC is a triangle right angled at C and M is mid-point of hypotenuse AB. Line drawn through M and parallel to BC intersects AC at D. Show that:
MD is mid-point of AC.
To solve the problem, we will follow these steps:
Step 1: Understand the Triangle and its Properties
We have a triangle ABC that is right-angled at C. The hypotenuse AB has a midpoint M. We need to draw a line through M that is parallel to BC, and this line intersects AC at point D.
Hint: Remember that in a right-angled triangle, the hypotenuse is opposite the right angle.
Step 2: Draw the Triangle
Draw triangle ABC with C being the right angle. Mark the points A, B, and C appropriately. Locate the midpoint M of the hypotenuse AB.
Hint: Use a ruler to ensure that M is exactly halfway between A and B.
Step 3: Draw the Parallel Line
From point M, draw a line parallel to side BC. This line will intersect side AC at point D.
Hint: Use a set square or a protractor to ensure that the line drawn through M is perfectly parallel to BC.
Step 4: Apply the Midpoint Theorem
According to the midpoint theorem, if a line is drawn through the midpoint of one side of a triangle and is parallel to another side, it will bisect the third side. Here, since M is the midpoint of AB and the line MD is parallel to BC, it follows that D is the midpoint of AC.
Hint: Recall the properties of similar triangles and parallel lines to justify why D must be the midpoint.
Step 5: Conclusion
Since we have established that D is the midpoint of AC, we can conclude that MD is indeed the midpoint of AC.
Hint: Summarize your findings clearly to reinforce the conclusion that you have proven D is the midpoint of AC.
Final Statement
Thus, we have shown that MD is the midpoint of AC, as required.
We have a triangle ABC that is right-angled at C. The hypotenuse AB has a midpoint M. We need to draw a line through M that is parallel to BC, and this line intersects AC at point D.
Draw triangle ABC with C being the right angle. Mark the points A, B, and C appropriately. Locate the midpoint M of the hypotenuse AB.
From point M, draw a line parallel to side BC. This line will intersect side AC at point D.
According to the midpoint theorem, if a line is drawn through the midpoint of one side of a triangle and is parallel to another side, it will bisect the third side. Here, since M is the midpoint of AB and the line MD is parallel to BC, it follows that D is the midpoint of AC.
Since we have established that D is the midpoint of AC, we can conclude that MD is indeed the midpoint of AC.
Thus, we have shown that MD is the midpoint of AC, as required.
Topper's Solved these Questions
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - pythagoras therorem
9 Videos
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - Rectillinear figures
24 Videos
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - Inequalities
5 Videos
- CHAPTER REVISION (STAGE 2) BOOK - ICSECHAPTER - CHAPTER REVISION (STAGE 2) EXERCISE - DISTANCE FORMULA
12 Videos
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - DISTANCE FORMULA
11 Videos
Similar Questions
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that (i) D is the mid-point of AC (ii) MD⊥AC (iii) CM=MA=12AB
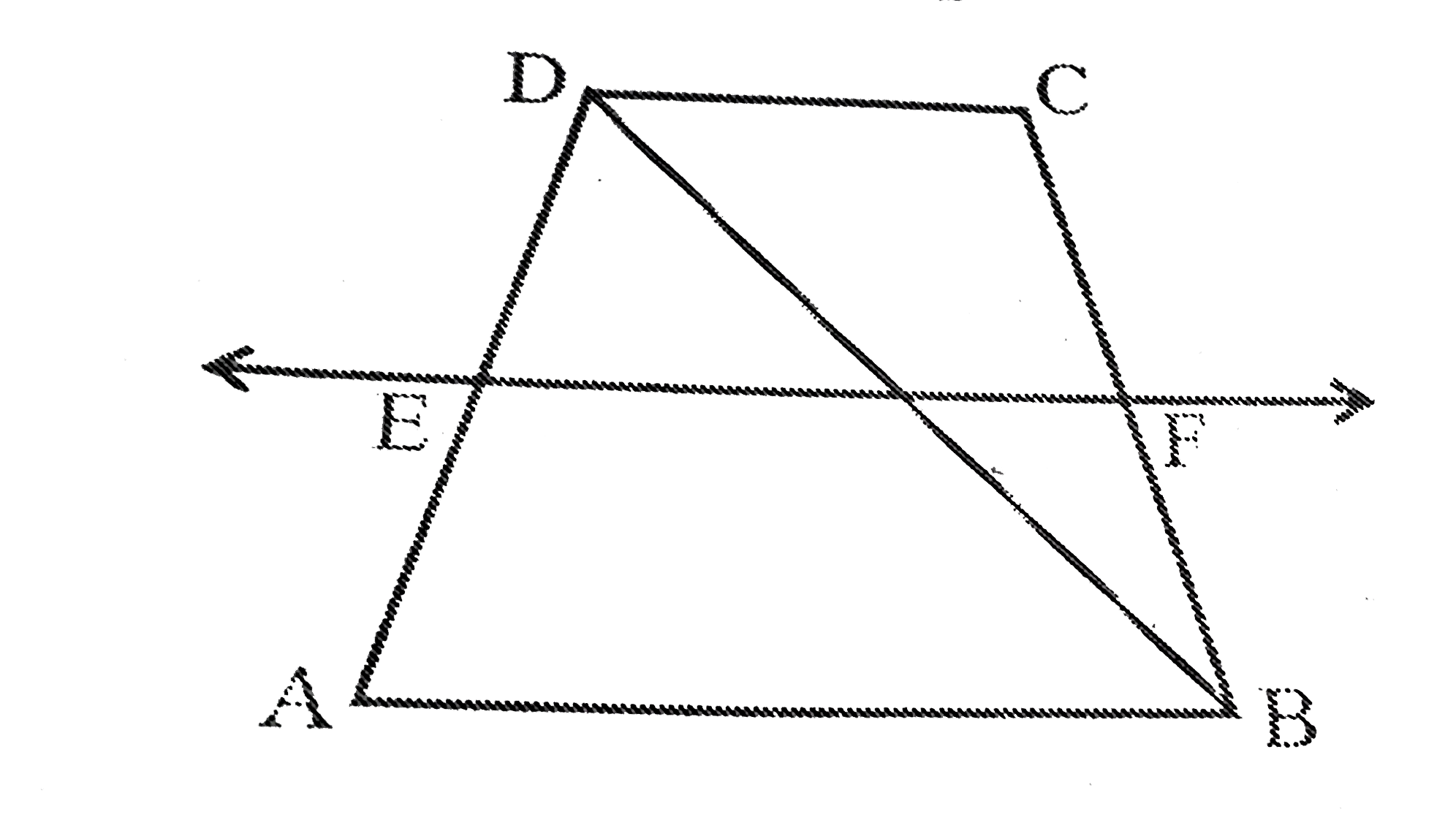
View SolutionABCD is a trapezium in which AB ∣∣ DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F (see Fig. 8.30). Show that F is the mid-point of BC.
View SolutionIn a right triangle ABC, right angled at C, P is the mid-point of hypotenuse AB. C is joined to P and produced to a point D, such that DP = CP. Point D is joined to point B. Show that:
CP=12AB
View SolutionIn a trapezium ABCD, AB/DC, E is mid-point of aD. A line through E and parallel to AB intersects BC at point F. Show that:
2EF=AB+DC
View SolutionIn a right triangle ABC, right angled at C, P is the mid-point of hypotenuse AB. C is joined to P and produced to a point D, such that DP = CP. Point D is joined to point B. Show that:
Angle DBC is a right angle.
View SolutionIn a right triangle ABC, right angled at C, P is the mid-point of hypotenuse AB. C is joined to P and produced to a point D, such that DP = CP. Point D is joined to point B. Show that:
ΔAPC≅ΔBPD
View SolutionIn a right triangle ABC, right angled at C, P is the mid-point of hypotenuse AB. C is joined to P and produced to a point D, such that DP = CP. Point D is joined to point B. Show that:
ΔDBC≅ΔACB
View SolutionE is the mid-point of the side AD of the tarapezium ABCD with AB∣∣DC. A line through E drawn parallel to AB intersects BC at F. Show that F is the mid-points of BC.
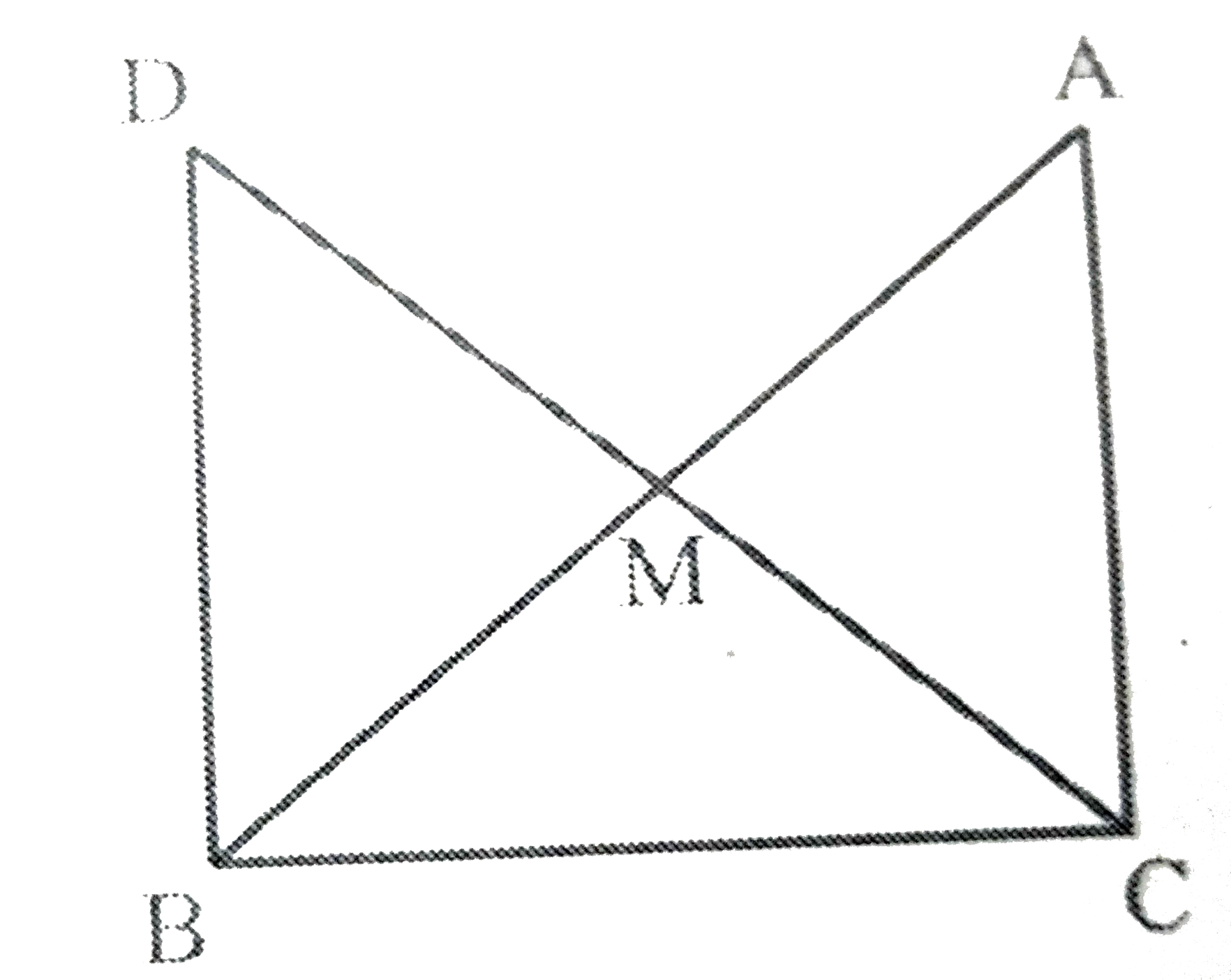
View SolutionIn right triangle ABC, right-angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see Fig. 7.23). Show that:
(i) ΔAMC≅ΔBMD
(ii) ∠DBC is a right angle
(iii) ΔDBC≅ΔACB
(iv) CM=12AB
View SolutionIn a triangle ABC, AD is a medium and E is mid-point of median AD. A line through B and E meets AC at point F.
View Solution
