P (4, 2) and Q (-1, 5) are the vertices of parallelogram PQRS and (-3, 2) are the co-ordinates of the point of intersection of its diagonals. Find co-ordinates of R and S.
To find the coordinates of points R and S in the parallelogram PQRS with given vertices P(4, 2) and Q(-1, 5) and the intersection of the diagonals at point O(-3, 2), we can use the midpoint formula.
1. Understanding the Midpoint Formula:
The midpoint M of a line segment connecting two points A(x1, y1) and B(x2, y2) is given by:
M=(x1+x22,y1+y22)
2. Finding Coordinates of R:
Let the coordinates of R be (x, y). The midpoint of diagonal PR is O(-3, 2). According to the midpoint formula:
O=(4+x2,2+y2)
Setting the x-coordinates equal:
−3=4+x2
Multiplying both sides by 2:
−6=4+x
Solving for x:
x=−6−4=−10
Now, setting the y-coordinates equal:
2=2+y2
Multiplying both sides by 2:
4=2+y
Solving for y:
y=4−2=2
Thus, the coordinates of R are (-10, 2).
3. Finding Coordinates of S:
Let the coordinates of S be (a, b). The midpoint of diagonal SQ is also O(-3, 2). Using the midpoint formula again:
O=(−1+a2,5+b2)
Setting the x-coordinates equal:
−3=−1+a2
Multiplying both sides by 2:
−6=−1+a
Solving for a:
a=−6+1=−5
Now, setting the y-coordinates equal:
2=5+b2
Multiplying both sides by 2:
4=5+b
Solving for b:
b=4−5=−1
Thus, the coordinates of S are (-5, -1).
Final Coordinates:
- R = (-10, 2)
- S = (-5, -1)
The midpoint M of a line segment connecting two points A(x1, y1) and B(x2, y2) is given by:
M=(x1+x22,y1+y22)
Let the coordinates of R be (x, y). The midpoint of diagonal PR is O(-3, 2). According to the midpoint formula:
O=(4+x2,2+y2)
Setting the x-coordinates equal:
−3=4+x2
Multiplying both sides by 2:
−6=4+x
Solving for x:
x=−6−4=−10
Now, setting the y-coordinates equal:
2=2+y2
Multiplying both sides by 2:
4=2+y
Solving for y:
y=4−2=2
Thus, the coordinates of R are (-10, 2).
Let the coordinates of S be (a, b). The midpoint of diagonal SQ is also O(-3, 2). Using the midpoint formula again:
O=(−1+a2,5+b2)
Setting the x-coordinates equal:
−3=−1+a2
Multiplying both sides by 2:
−6=−1+a
Solving for a:
a=−6+1=−5
Now, setting the y-coordinates equal:
2=5+b2
Multiplying both sides by 2:
4=5+b
Solving for b:
b=4−5=−1
Thus, the coordinates of S are (-5, -1).
- R = (-10, 2)
- S = (-5, -1)
Topper's Solved these Questions
- SECTION AND MID-POINT FORMULABOOK - ICSECHAPTER - SECTION AND MID-POINT FORMULAEXERCISE - EXERCISE 13 (C)
28 Videos
- SECTION AND MID-POINT FORMULABOOK - ICSECHAPTER - SECTION AND MID-POINT FORMULAEXERCISE - QUESTIONS
17 Videos
- SECTION AND MID-POINT FORMULABOOK - ICSECHAPTER - SECTION AND MID-POINT FORMULAEXERCISE - QUESTIONS
17 Videos
- SAMPLE PAPER 5 (MATHEMATICS)BOOK - ICSECHAPTER - SAMPLE PAPER 5 (MATHEMATICS)EXERCISE - SECTION C
8 Videos
- SHARES AND DIVIDENDSBOOK - ICSECHAPTER - SHARES AND DIVIDENDSEXERCISE - Exercise 3(C )
20 Videos
Similar Questions
A (-1, 0), B (1, 3) and D (3, 5) are the vertices of a parallelogram ABCD. Find the co-ordinates of vertex C.
View SolutionFind the co-ordinates of the point of intersection of the straight lines
3x−5y+5=0,2x+3y−22=0
View SolutionFind the co-ordinates of the points of trisection of the line segment joining the points A(2, -3, 5) and B(6, 0, -1).
View SolutionFind the co-ordinates of the point of intersection of the medians of triangle ABC, given A = (-2, 3), B = (6, 7) and C = (4,1).
View SolutionIf PQ is the focal chord of the parabola y2=−xandP is (−4,2), then the ordinate of the point of intersection of the tangents at P and Q is
View SolutionThe line through P(5, 3) intersects y-axis at Q.
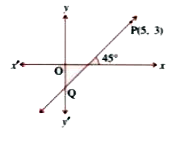
Find the co-ordinates of Q.
View SolutionThe area of a parallelogram is 12 square units. Two of its vertices are the points A(−1,3)andB(−2,4). Find the other two vertices of the parallelogram, if the point of intersection of diagonals lies on x-axis on its positive side.
View SolutionThe three vertices of a parallelogram ABCD are A(-1,3,4), B(2,-1,3) and C(5,1,2). Find the co-ordinates of its 4th vertex D.
View SolutionA(-2,4),C(4,10) and D(-2,10) are the vertices of a square ABCD. Use the graphical method to find the co-ordinates of the fouth vertex B. Find :
(i) the co-ordinates of the mid point of BC
(ii) the co-ordinates of the mix point of CD and
(iii) the co-ordinates of the point of intersection of the diagonals of the square ABCD.
View SolutionIn what ratio is the join of (4, 3) and (2, -6) divided by the x-axis ? Also, find the co-ordinates of the point of intersection.
View Solution
