In ΔABC,∠B=90∘ and BD⊥AC.
If AC = 9 cm and AB = 7 cm, find AD.
The correct Answer is:549cm
To solve the problem, we will use the properties of right triangles and the Pythagorean theorem. Here are the steps to find the length of AD in triangle ABC where ∠B = 90° and BD ⊥ AC.
1. Understand the Triangle Setup:
- We have triangle ABC with ∠B = 90°.
- BD is perpendicular to AC, which means BD is the height from point B to side AC.
- We know AC = 9 cm and AB = 7 cm.
2. Define the Segments:
- Let AD = x cm.
- Therefore, CD = AC - AD = 9 - x cm.
3. Apply the Pythagorean Theorem in Triangle ABD:
- According to the Pythagorean theorem:
AB2=AD2+BD2
- Substituting the known values:
72=x2+BD2
- This simplifies to:
49=x2+BD2(Equation 1)
4. Apply the Pythagorean Theorem in Triangle BCD:
- Again using the Pythagorean theorem:
BC2=BD2+CD2
- Substituting CD = 9 - x:
BC2=BD2+(9−x)2
- Expanding this:
BC2=BD2+(81−18x+x2)(Equation 2)
5. Use the Pythagorean Theorem in Triangle ABC:
- Since ∠B = 90°, we can apply the Pythagorean theorem:
AC2=AB2+BC2
- Substituting the known values:
92=72+BC2
- This simplifies to:
81=49+BC2
- Therefore:
BC2=81−49=32(Equation 3)
6. Substitute Equation 3 into Equation 2:
- From Equation 2, we have:
32=BD2+(81−18x+x2)
- Rearranging gives:
BD2=32−81+18x−x2
- Thus:
BD2=18x−x2−49(Equation 4)
7. Equate Equation 1 and Equation 4:
- From Equation 1:
BD2=49−x2
- Set this equal to Equation 4:
49−x2=18x−x2−49
- Simplifying gives:
49+49=18x
- Therefore:
98=18x
- Solving for x:
x=9818=499≈5.44 cm
Final Answer:
AD = 499 cm or approximately 5.44 cm.
- We have triangle ABC with ∠B = 90°.
- BD is perpendicular to AC, which means BD is the height from point B to side AC.
- We know AC = 9 cm and AB = 7 cm.
- Let AD = x cm.
- Therefore, CD = AC - AD = 9 - x cm.
- According to the Pythagorean theorem:
AB2=AD2+BD2
- Substituting the known values:
72=x2+BD2
- This simplifies to:
49=x2+BD2(Equation 1)
- Again using the Pythagorean theorem:
BC2=BD2+CD2
- Substituting CD = 9 - x:
BC2=BD2+(9−x)2
- Expanding this:
BC2=BD2+(81−18x+x2)(Equation 2)
- Since ∠B = 90°, we can apply the Pythagorean theorem:
AC2=AB2+BC2
- Substituting the known values:
92=72+BC2
- This simplifies to:
81=49+BC2
- Therefore:
BC2=81−49=32(Equation 3)
- From Equation 2, we have:
32=BD2+(81−18x+x2)
- Rearranging gives:
BD2=32−81+18x−x2
- Thus:
BD2=18x−x2−49(Equation 4)
- From Equation 1:
BD2=49−x2
- Set this equal to Equation 4:
49−x2=18x−x2−49
- Simplifying gives:
49+49=18x
- Therefore:
98=18x
- Solving for x:
x=9818=499≈5.44 cm
AD = 499 cm or approximately 5.44 cm.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(B)17videos
SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)
- BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(C)24videos
SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)
- BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(E)61videos
SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)
- BOOK - ICSECHAPTER - SIMILARITY EXERCISE - MULTIPLE CHOICE QUESTIONS (COMPETENCY BASED QUESTIONS)10videos
SIMILARITY
- BOOK - ICSECHAPTER - SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)EXERCISE - Exercise 6(e )18videos
SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)
Similar Questions
In ΔABC,∠B=90∘ and BD⊥AC.
If AC = 18 cm and AD = 6 cm, find BD.View SolutionIn ΔABC,∠B=90∘ and BD⊥AC.
If CD = 10 cm and BD = 8 cm, find AD.View SolutionIn ΔABC,∠ABC=∠DAC,AB=8cm,AC=4cmandAD=5cm.

Find BC and CD.View SolutionIn ΔABC,∠ABC=∠DAC,AB=8cm,AC=4cmandAD=5cm.

Find area of ΔACD: area of ΔABC.View SolutionIn ΔABC,∠B=90∘,AB=12cmandAC=15cm. D and E are points on AB and AC respectively such that ∠AED=90∘ and DE = 3 cm. Calculate the area of ΔABC and then the area of ΔADE.
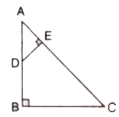 View Solution
View SolutionΔABC is right-angled at C. If AC=5cmandBC=12cm find the length of AB.
View SolutionIn the given figure, ABC is a right angled triangle with ∠BAC=90∘.

If BD = 18 cm and CD = 8 cm, find AD.View SolutionIn ΔABC,∠ABC=∠DAC,AB=8cm,AC=4cmandAD=5cm.

Prove that ΔACD is similar to ΔBCA.View SolutionIn triangle ABC, AB = AC = 15 cm and BC = 18 cm. Find :
cos BView Solutionin the given figure ∠CAB=90∘andAD⊥BC. if AC= 75 cm , AB= 1m and BD= 1.25 m . Find AD.
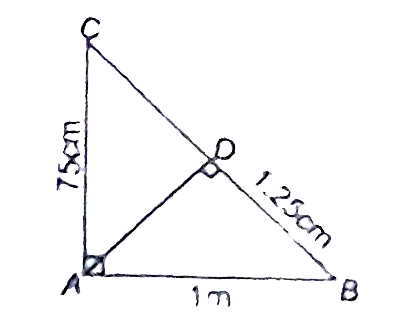 View Solution
View Solution

