In the right-angled triangle QPR, PM altitude.
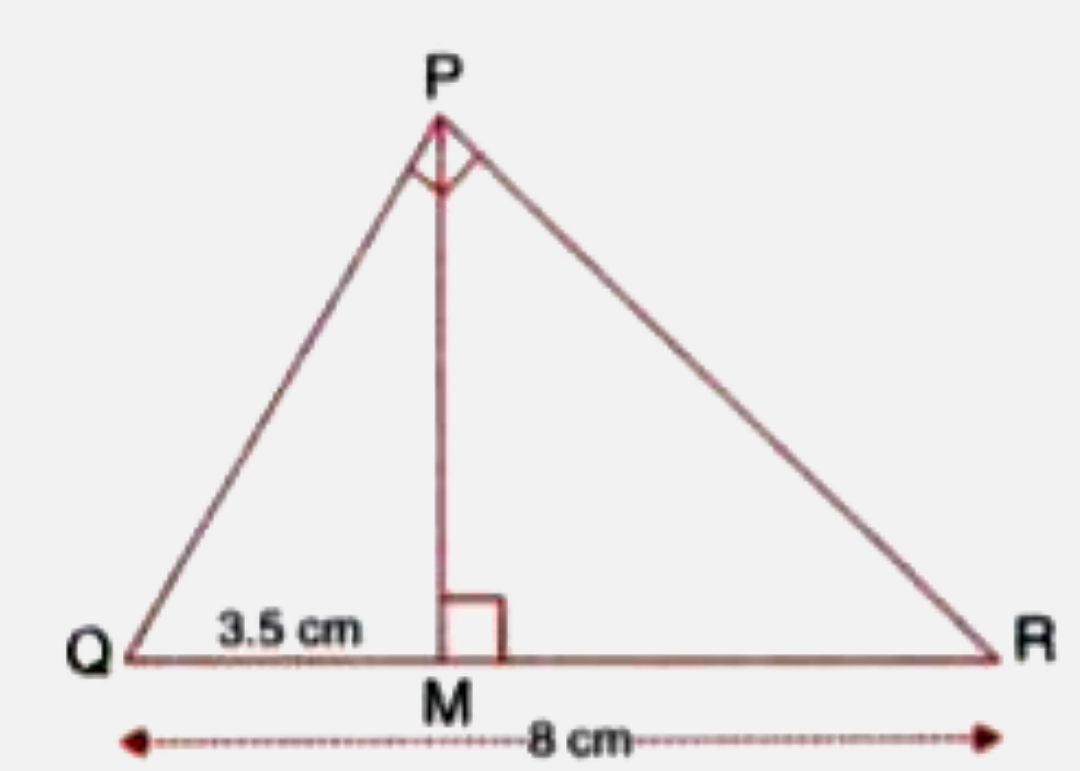
Given that QR = 8 cm and MQ = 3-5 cm, calculate the value of PR.
The correct Answer is:6 cm
Step by step video & image solution for In the right-angled triangle QPR, PM altitude. Given that QR = 8 cm and MQ = 3-5 cm, calculate the value of PR. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(B)
17 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(C)
24 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(E)
61 Videos
- SIMILARITY BOOK - ICSECHAPTER - SIMILARITY EXERCISE - MULTIPLE CHOICE QUESTIONS (COMPETENCY BASED QUESTIONS)
10 Videos
- SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)BOOK - ICSECHAPTER - SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)EXERCISE - Exercise 6(e )
18 Videos
Similar Questions
In triangle ABC, given below, AB = 8 cm, BC = 6 cm and AC = 3 cm. Calculate the length of OC

View SolutionThe sides of right-angled triangle containing the right angle are 5x cm and (3x- 1) cm. Calculate the lengths of the hypotenuse of the triangle. If its area is 60 cm2
View SolutionIn triangle ABC is right-angled at B. Given that AB=9cm, AC=15cmcalculate B C.
View SolutionABC is a right-angled triangle with the right angle at vertex B. BD is the altitude through B. Given BD = 12 cm and AD = 9 cm.
Calculate AB .
View SolutionIn the given triangle PQR, LM is parallel to QR and PM : MR = 3: 4.
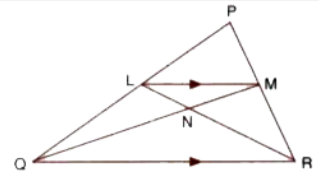
Calculate the value of ratio :
PLPQ and then LMQR
View SolutionAn equilateral triangle if its altitude is 3.2 cm.
View SolutionIn the adjoining figure, ABC is a triangle right-angled at vertex A and AD is altitude.
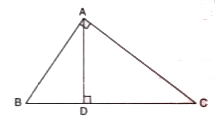
If BD = 3.6 cm and CD = 6.4 cm, find the length of AD.
View SolutionIn the given triangle PQR, LM is parallel to QR and PM : MR = 3: 4.
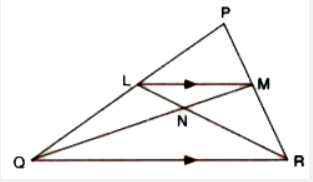
Calculate the value of ratio :
Area of ΔLMNArea of ΔMNR
View SolutionIn the given triangle PQR, LM is parallel to QR and PM : MR = 3: 4.
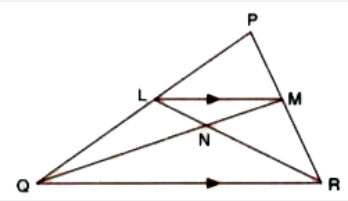
Calculate the value of ratio :
Area of ΔLQMArea ofΔLQN
View SolutionIn the figure, given below, PQR is a right angled triangle right angled at Q. XY is parallel to QR, PQ = 6 cm, PY = 4 cm and PX : XQ = 1:2. Calculate the lengths of PR and QR.

View Solution
