In the figure, given below, the medians BD and CE of a triangle ABC meet at G. Prove that:
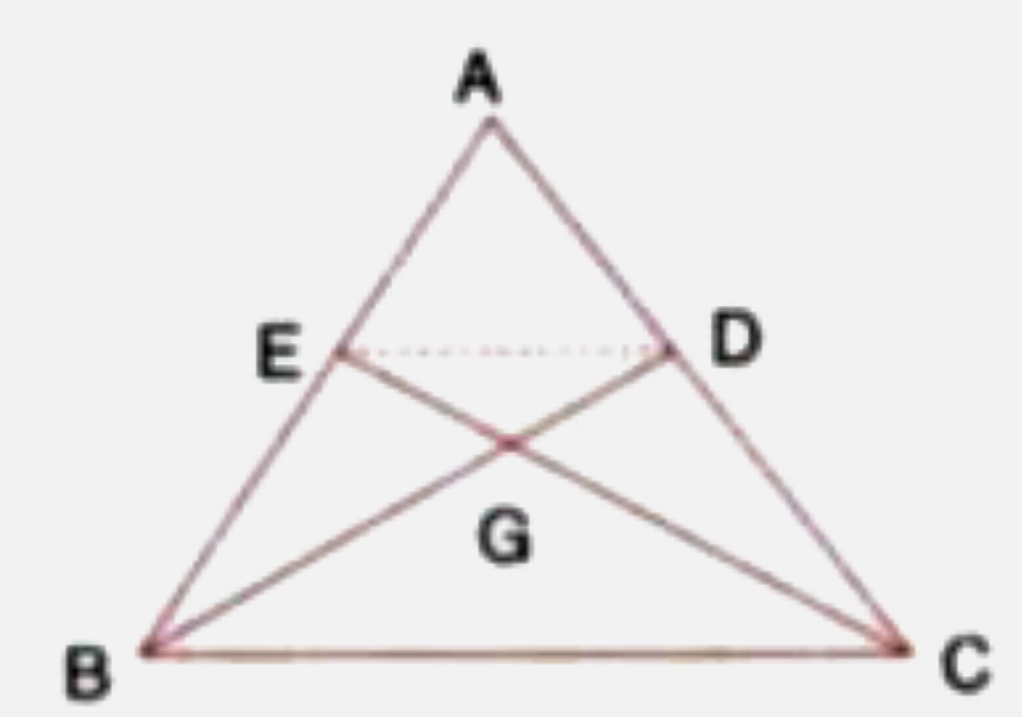
ΔEGD−ΔCGBand (ii) BG=2GD from (i) above.
Step by step video & image solution for In the figure, given below, the medians BD and CE of a triangle ABC meet at G. Prove that: DeltaEGD - DeltaCGB and (ii) BG = 2 GD from (i) above. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(B)
17 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(C)
24 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(E)
61 Videos
- SIMILARITY BOOK - ICSECHAPTER - SIMILARITY EXERCISE - MULTIPLE CHOICE QUESTIONS (COMPETENCY BASED QUESTIONS)
10 Videos
- SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)BOOK - ICSECHAPTER - SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)EXERCISE - Exercise 6(e )
18 Videos
Similar Questions
In the figure, given below, straight lines AB and CD intersect at P, and AC//BD. Prove that :
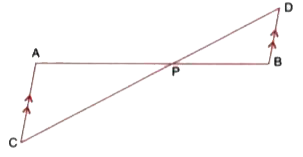
ΔAPCandΔBPD are similar.
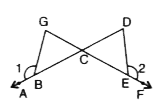
View SolutionIn the given figure , BC = CE and ∠1=∠2.
Prove that :
ΔGCB=ΔDCB .
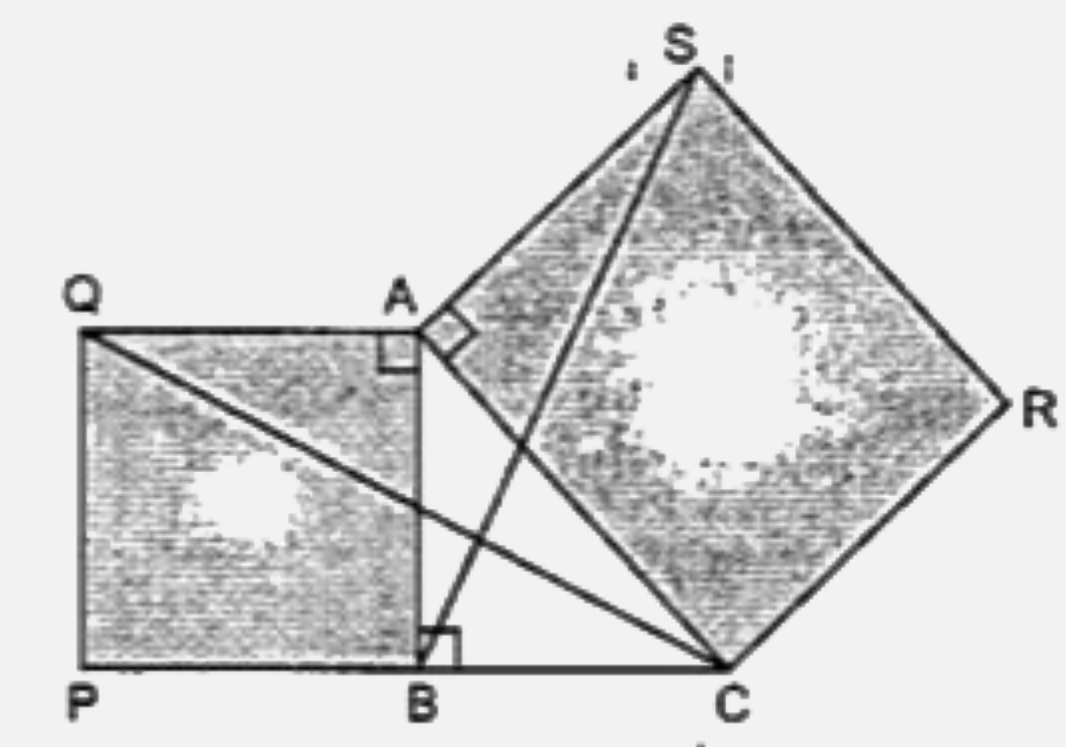
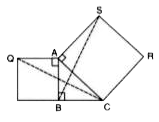
View SolutionIn the figure, given below, triangle ABC is right-angled at B. ABPQ and ACRS are squares.
Prove that :
CQ=BS
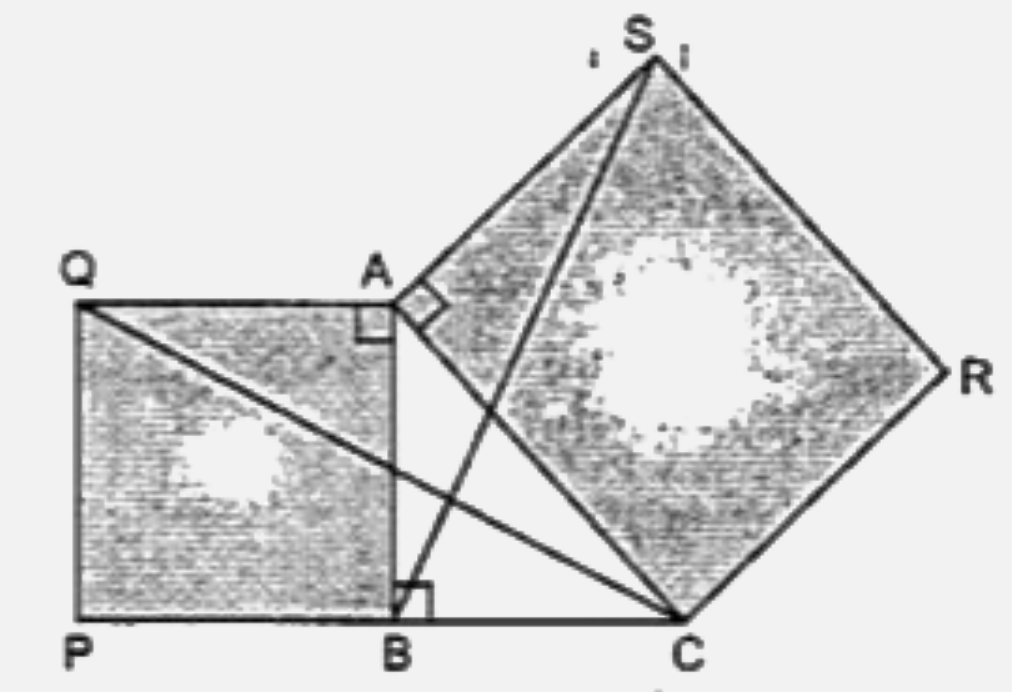
View SolutionIn the figure, given below, triangle ABC is right-angled at B. ABPQ and ACRS are squares. Prove that :
(i) ΔACQandΔASB are congruent.
(ii) CQ = BS
View SolutionIn the figure, given below, triangle ABC is right-angled at B. ABPQ and ACRS are squares.
Prove that :
ΔACQ and ΔASB are congruent
View SolutionIn the following figure, AD and CE are medians of ΔABC. DF is drawn parallel to CE. Prove that :
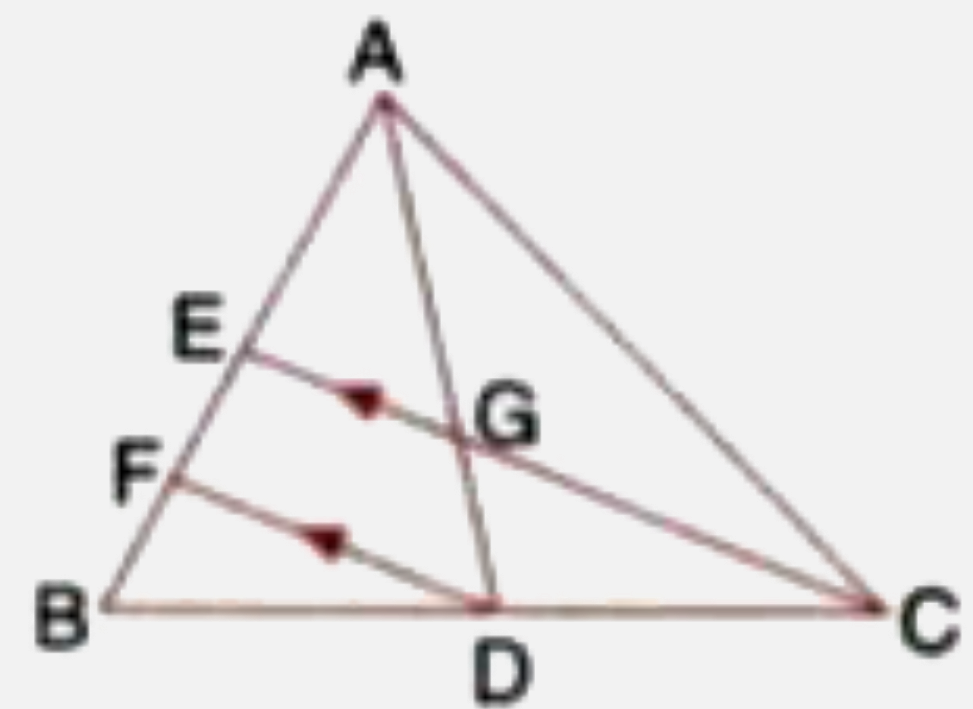
AG:GD=2:1.
View SolutionIn the figure, given alongside, AD bisects angle BAC. Prove that :
(i) AB>BD
(ii) AC>CD
(iii) AB+AC>BC
View SolutionIn the figure, given alongside, AD bisects angle BAC. Prove that :
(i) AB>BD
(ii) AC>CD
(iii) AB+AC>BC

View SolutionThe given figure shows an equilateral triangles ABC and D is point in AC.
Prove that :
(i) AD<BD
(ii)BC>BD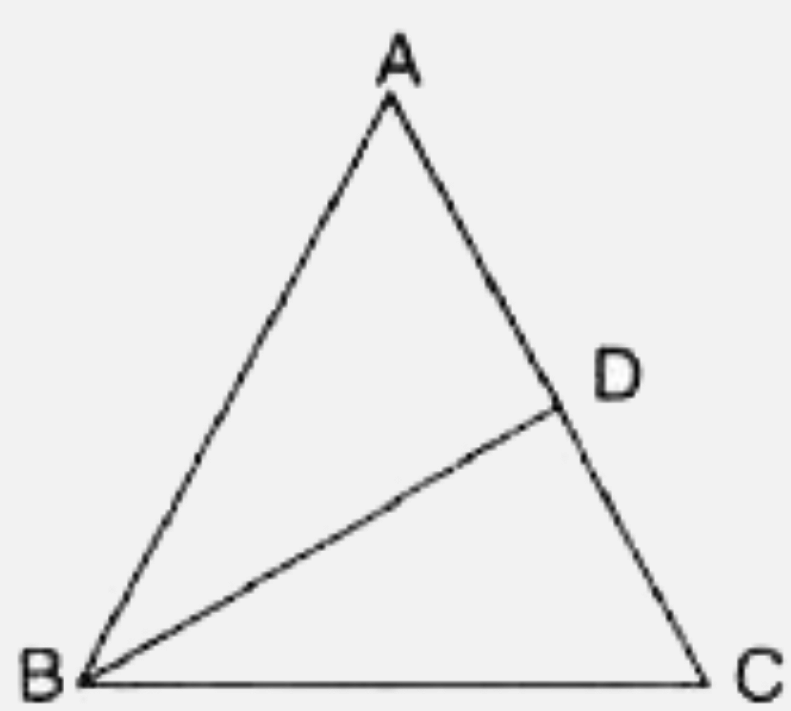
View SolutionIn the given figure , CM and RN are respectively the median of triangles ABC and PQR

IF ΔABC is similar to ΔPQR ,prove that
(i) ΔAMC~ΔPNR
(ii) CMRN=ABPQand
(iii)ΔCMB~ΔRNQ
View Solution
