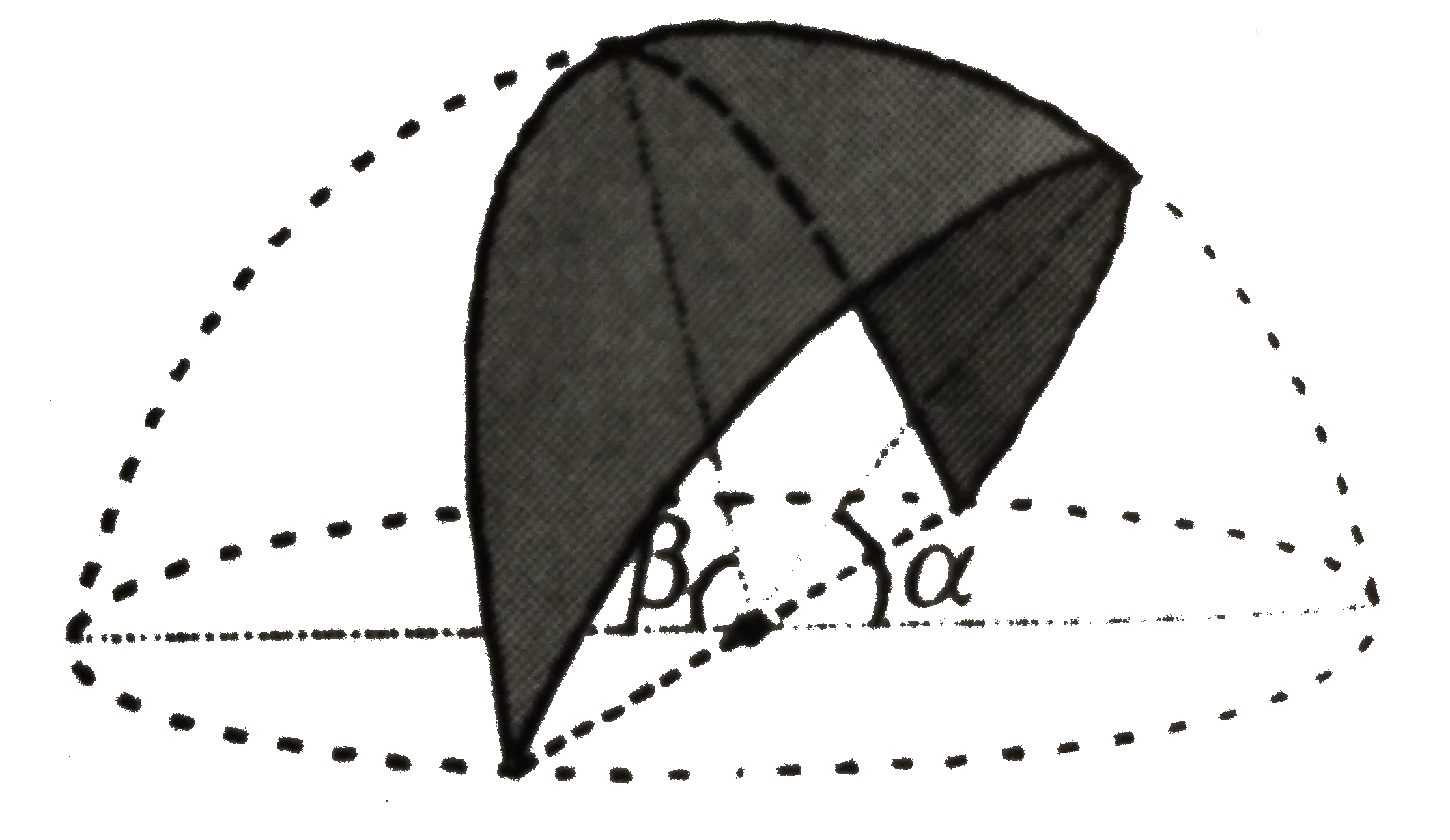
The electric field intensity at the center of a uniformly charged hemispherical shell is E0. Now two portions of the hemisphere are cut from either side, and the remaining portion is shown in fig. If α=β=π/3, then the electric field intensity at the center due to the remaining portion is
E03
E06
E02
E0
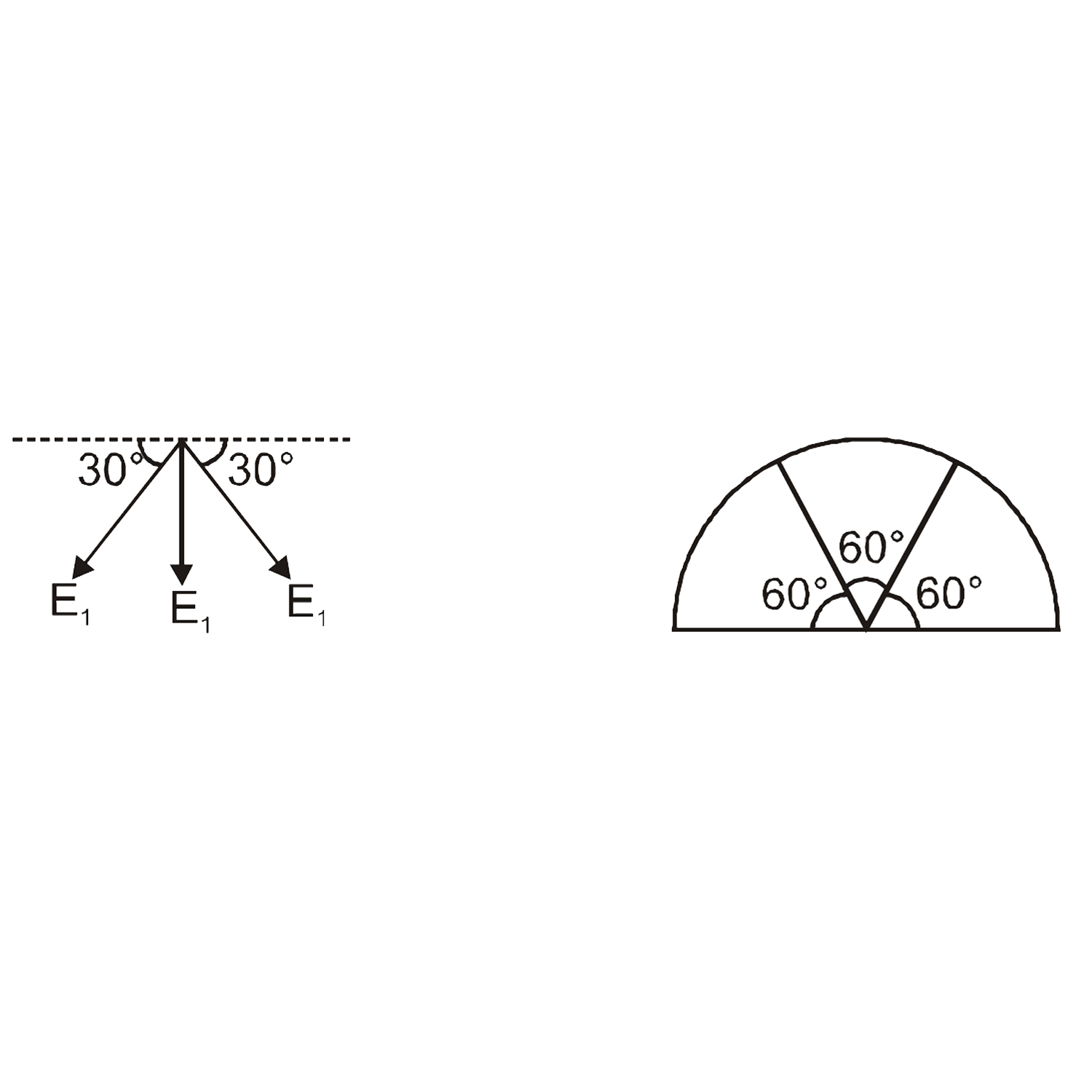
consider the whole hemispere as three portion if electric field due to one portion is E1 then 2E1sin30+E1=E0
2E1=E0
⇒E1=E02
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Electric field intensity (E) due to an electric dipole varies with distance (r ) from the point of the center of dipole as :
A1rand1r2B1r2and1rC1r3and1r3D1r3and1r2
Due to an electric dipole shown in fig., the electric field intensity is parallel to dipole axis :
 View Solution
View SolutionThe electric field at a distance 3R/2 from the centre of a charge conducting spherical shell of radius R is E. The electric field at a distance R/2 from the centre of the sphere is
View SolutionThe electric field at a distance 3R/2 from the centre of a charge conducting spherical shell of radius R is E. The electric field at a distance R/2 from the centre of the sphere is
View SolutionTwo charges e and 3e are placed at a distance r. The distance of the point where the electric field intensity will be zero is
View Solution
A small part of dl length is removed from a ring having charge per unit length λ. Find electric field at centre due to remaining ring.View SolutionFind the electric field potential and strength at the center of a hemisphere of radius R charged uniformly with the the surface density σ.
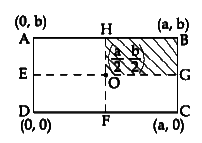
View SolutionA uniform rectangular thin sheet ABCD of mass M has length a and breadth b, as shown in the figure. If the shaded portion HBGO is cut-off, the coordinates of the center of mass of the remaining portion will be:
 View Solution
View Solution