Question

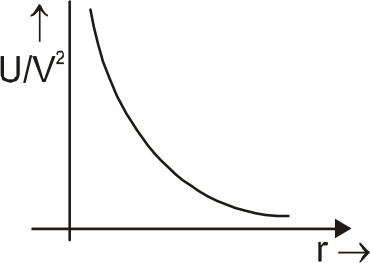
At distance 'r' from a point charge, the ratio $\frac{U}{V^2}$ (where 'U' is energy density and 'V' is potential) is best respresented by :

Options

A.
B.
C.
D.

Explanation

To solve the problem, we need to find the ratio $$\frac{U}{V^2}$$ where $$U$$ is the energy density and $$V$$ is the electric potential at a distance $$r$$ from a point charge $$Q$$.

1. Understand the Definitions:
- The electric potential \( V \) at a distance \( r \) from a point charge \( Q \) is given by:
\( V = \frac{kQ}{r} \)
where \( k \) is Coulomb's constant.

2. Calculate the Electric Field \( E \):
- The electric field \( E \) at a distance \( r \) from a point charge \( Q \) is given by:
\( E = \frac{kQ}{r^2} \)

3. Calculate the Energy Density \( U \):
- The energy density \( U \) in an electric field is given by:
\( U = \frac{1}{2} \epsilon_0 E^2 \)
- Substituting the expression for \( E \):
\( U = \frac{1}{2} \epsilon_0 \left(\frac{kQ}{r^2}\right)^2 \)
- Simplifying this gives:
\( U = \frac{1}{2} \epsilon_0 \frac{k^2 Q^2}{r^4} \)

4. Calculate \( V^2 \):
- Now, we find \( V^2 \):
\( V^2 = \left(\frac{kQ}{r}\right)^2 = \frac{k^2 Q^2}{r^2} \)

5. Find the Ratio \( \frac{U}{V^2} \):
- Now we can find the ratio:
\( \frac{U}{V^2} = \frac{\frac{1}{2} \epsilon_0 \frac{k^2 Q^2}{r^4}}{\frac{k^2 Q^2}{r^2}} \)
- Simplifying this ratio:
\( \frac{U}{V^2} = \frac{1}{2} \epsilon_0 \frac{k^2 Q^2}{r^4} \cdot \frac{r^2}{k^2 Q^2} = \frac{1}{2} \epsilon_0 \frac{1}{r^2} \)

6. Conclusion:
- The final expression for the ratio \( \frac{U}{V^2} \) is:
\( \frac{U}{V^2} = \frac{1}{2} \frac{\epsilon_0}{r^2} \)
- This shows that \( \frac{U}{V^2} \) is inversely proportional to \( r^2 \).

Answer:
The ratio \( \frac{U}{V^2} \) is best represented by a hyperbolic relationship as \( r \) varies, confirming that it is inversely proportional to \( r^2 \).