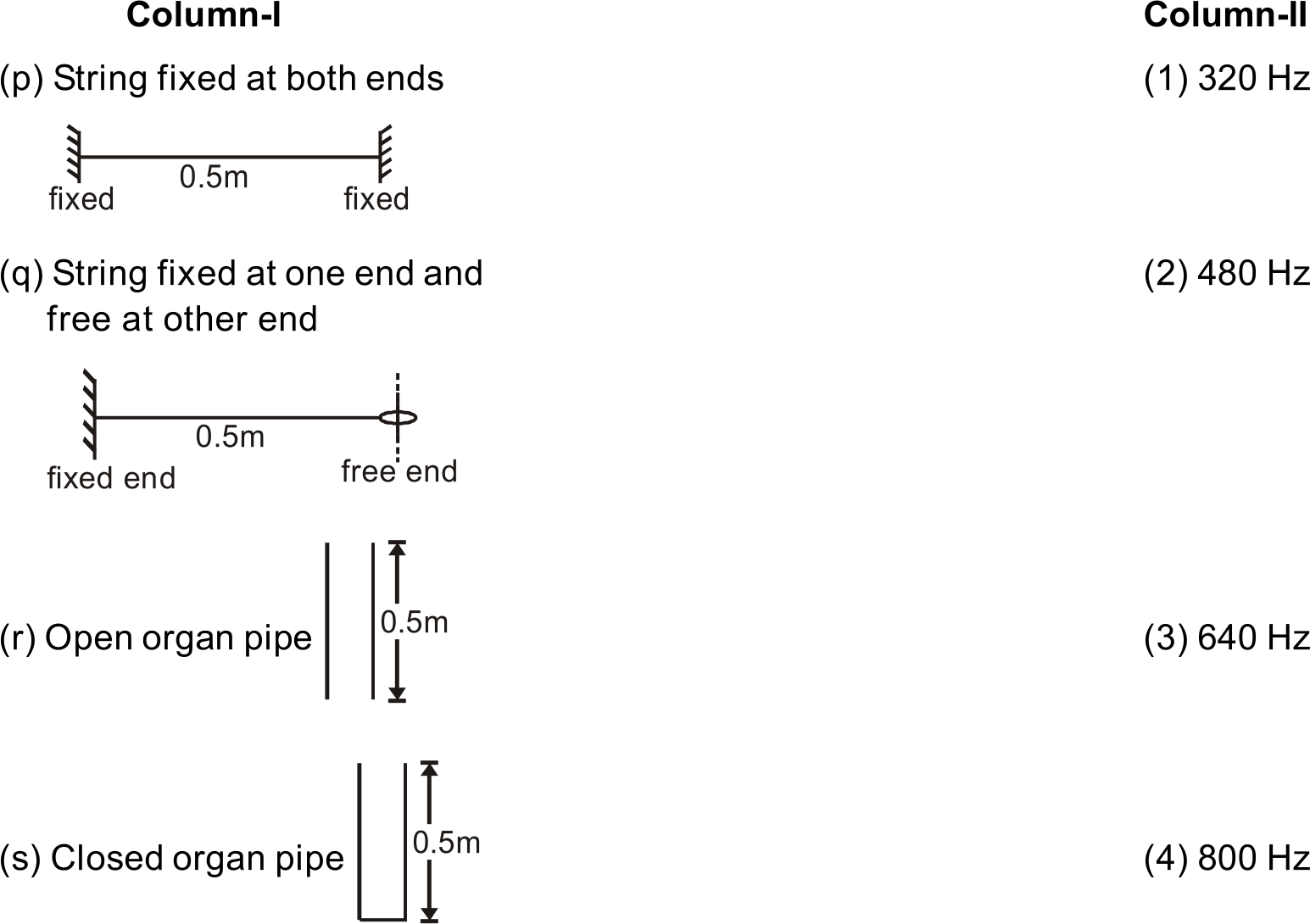
In each of the four situation of table -I a stretched string or an organ pipe is given with the required date. In case of string the tension in string is T = 102.4 N and the mass per unit length of string is 1 g/m . Speed of sound in air is 320 m/s. Neglect end corrections. Match each situation in table -I with the possible resonance frequencies given in Table -II. 
pqrs(A)1324
pqrs(B)1432
pqrs(C)3241
pqrs(D)2413
(A) The fundamental frequency in the string.
f0=√T/μ2l=√102.41×10−3×12×0.5Hz=320Hz.
Other possible resonance frequencies are fAandf0=320Hz,640Hz,960Hz
(B)The fundamental frequency in the string.
f0=√T/μ4l=3204×0.5=160Hz.
Other possible resonance frequencies are
fB=160 Hz, 480 Hz, 800 Hz.
(C )The fundamental frequency in both ends open organ pipe is
f0=v2l=3202×0.5=320Hz
Other possible resonance frequencies are
fc=320Hz,640Hz,960Hz
(D)The fundamental frequency in one end open organ pipe is
f0=v4l=3204×0.5=160Hz
Other possible resonance frequencies are fD=160Hz,480Hz,800Hz
Topper's Solved these Questions
Similar Questions
In each of the four situations of column-I , a stretched string or an organ pipe is given along with the required data. In case of strings the tension in string is T=102.4 N and the mass per unit length of string is 1g/m. Speed of sound in air is 320 m/s. Neglect end corrections.The frequencies of resonance are given in column-II.Match each situation in column-I with the possible resonance frequencies given in Column-II.
View SolutionVelocity of secnod in air is 320 m/s. Neglecting end correctionss, the air column in the pipe can resonate for sound of frequency
View SolutionA narrow pipe of length 2m is closed at one end. The velocity of sound in air is 320 m/s. Neglecting end corrections , the air column in the pipe will resonate for sound of frequencies :
View SolutionThe frequency v of the stretched string may depend on (i) the length of the vibrating segment 1 (ii) the tension in the string and (iii) the mass per unit length m. Show that v∝1l√Fm.
View SolutionDerive an expression for the velocity of pulse in a in stretched in string under a tension T and μ is the mass per unit length of the sting.
View SolutionThe frequency (f) of a stretched string depends upen the tension F (dimensions of form ) of the string and the mass per unit length mu of string .Derive the formula for frequency
View SolutionVelocity of sound in air is 320 m/s. For an organ pipe closed at one end of length 2 m the resonating frequency may be
View SolutionHow is the frequency of a stretched string related to:
Its tension?
View SolutionTension in the strings is 100 N mass per unit length of strings is 0.01 kg/m, velocity of sound in air is 320 m/s.
Column I : gives situation.
Column II : gives frequency of resonance.
Column III: gives wavelength for corresponding resonating frequency.
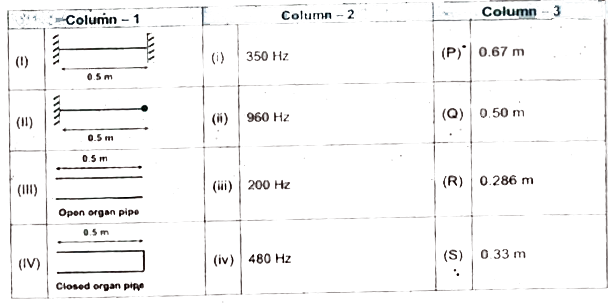
Pick correct combination.
View SolutionA string of 7m length has a mass of 0.035kg. If tension in the string is 60.N, then speed of a wave on the string is
View Solution
