In the situation as shown in figure time period of small vertical oscillation of block will be - (String, springs and pulley are ideal)
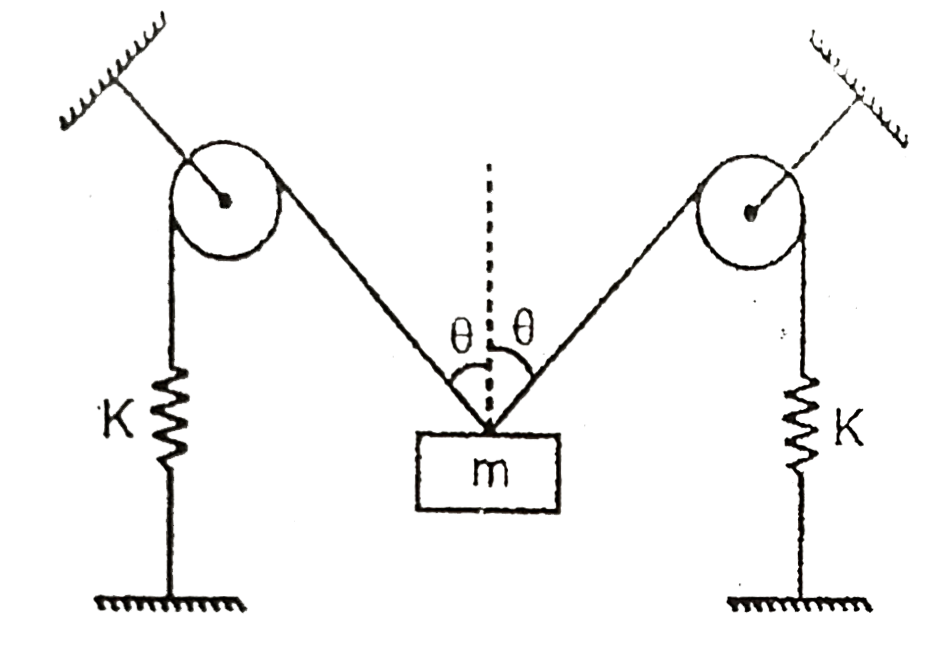
2πcosθ√m2k
2πsecθ√m2k
2πsinθ√m2k
2πcosecθ√m2k
The correct Answer is:B
In the situtation ………….
Let block is displaced through a small displacement x in downward direction and elogation in spring =x1 then
xcosθ=x1 ….(1)
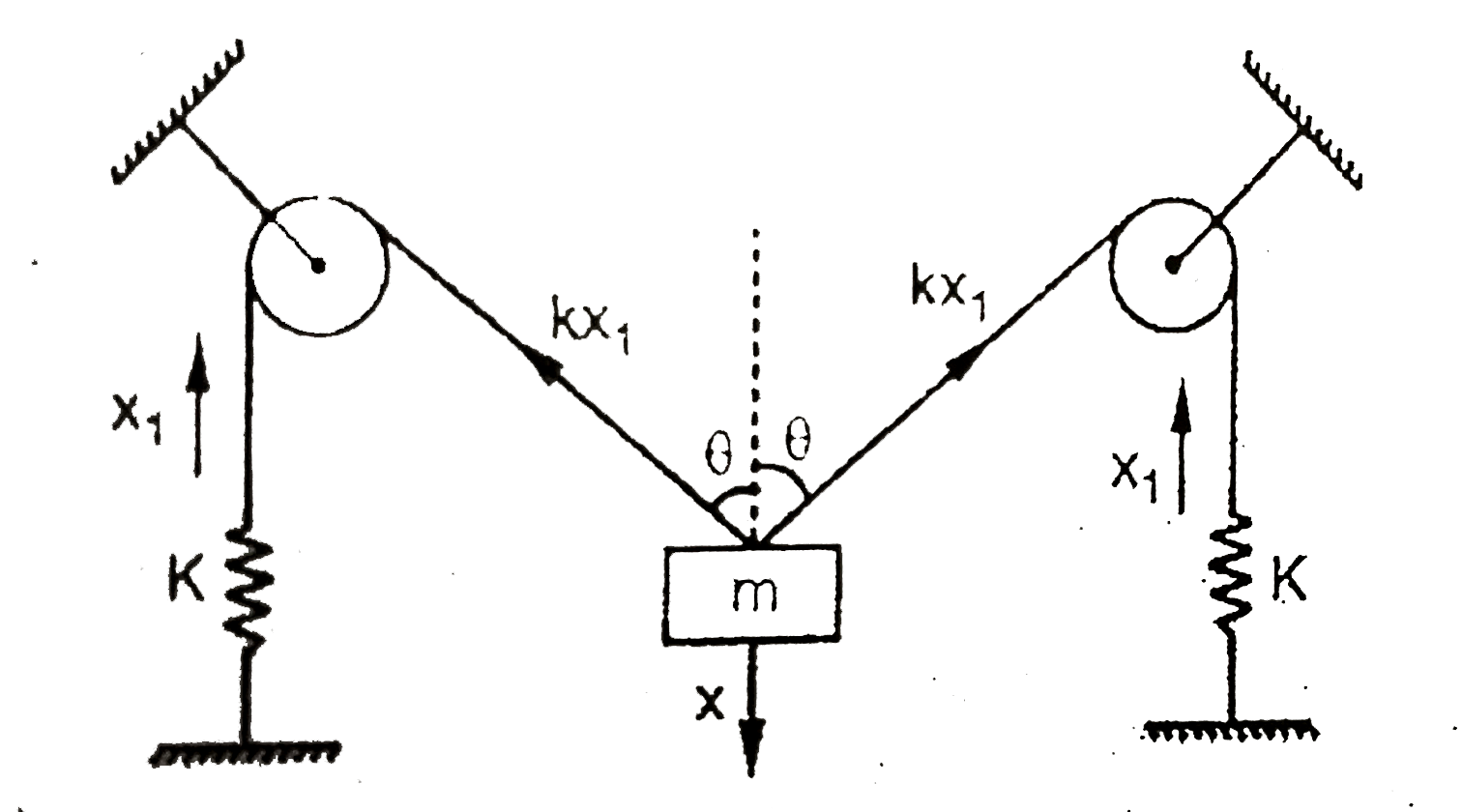
Restoring force F=2kx1cosθ
F=2kcos2θx
Hence T=2π√m2kcos2θ=2πsecθ√m2k
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
106 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
Find the time period of oscillation of block of mass m. Spring, and pulley are ideal. Spring constant is k.

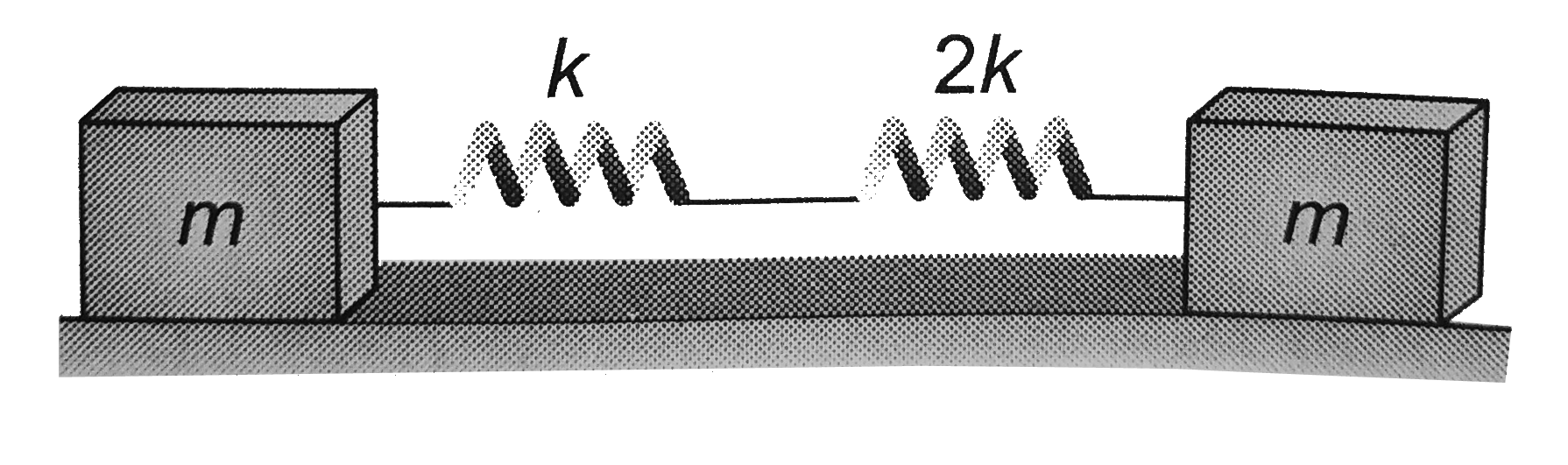
View SolutionA system is shown in the figure. The force The time period for small oscillations of the two blocks will be
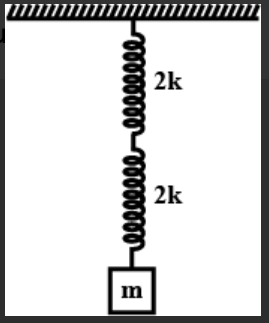
View SolutionThe time period of oscillation of the block as shown in figure is
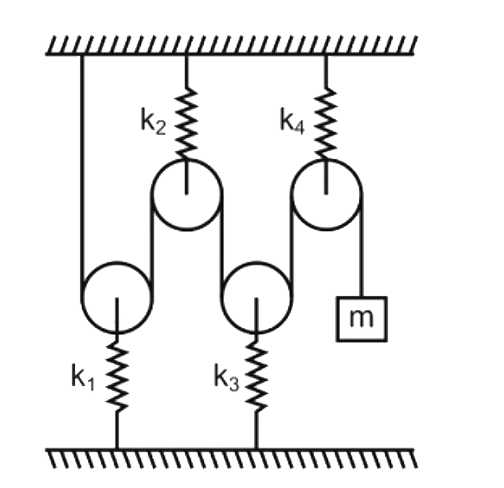
View SolutionIn the arrangement shown in the diagram, pulleys are small and springs are ideal. k1=k2=k3=k4=10Nm−1 are force constants of the springs and mass m=10kg. If the time period of small vertical oscillations of the block of mass m is given by 2πx seconds, then find the value of x.
View SolutionSpring mass system is shown in figure. find the time period of vertical oscillations. The system is in vertical plane.

View SolutionConsider the situation shown in the figure. A mass is hanging from a inextensible string which is passing over a pulley. The pulley itself is attached to a massless spring of stiffness k as shown in the figure. Find the time period of vertical oscillations of mass m if pulley is slightly displaced from its mean position. Assume that string does not slip over pulley.
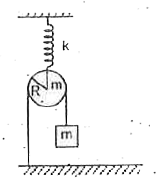
View SolutionThe pulley shown in figure has a moment of inertias I about its axis and mass m. find the time period of vertical oscillation of its centre of mass. The spring has spring constant k and the string does not slip over the pulley.
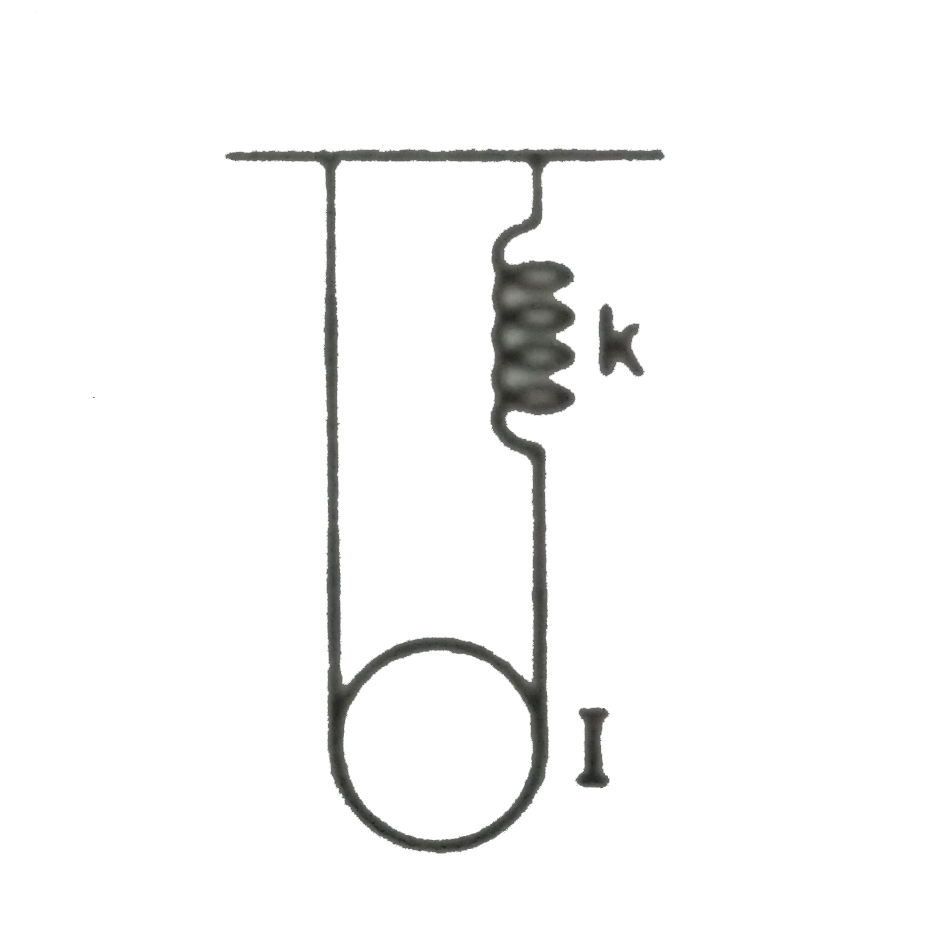
View SolutionThe pulley shown in figure has a moment of inertias I about its xis and mss m. find the tikme period of vertical oscillastion of its centre of mass. The spring has spring constant k and the string does not slip over the pulley.

View SolutionA uniform cubical block of mass M is attached to a pulley. One end of a string, passing over the pulley is fixed to a light pole while is fixed on the bigger block and its another end is attached to a block of mass m as shown in figure. The maximum value of the mass m so that the block does not topple is : (string and pulley are ideal)

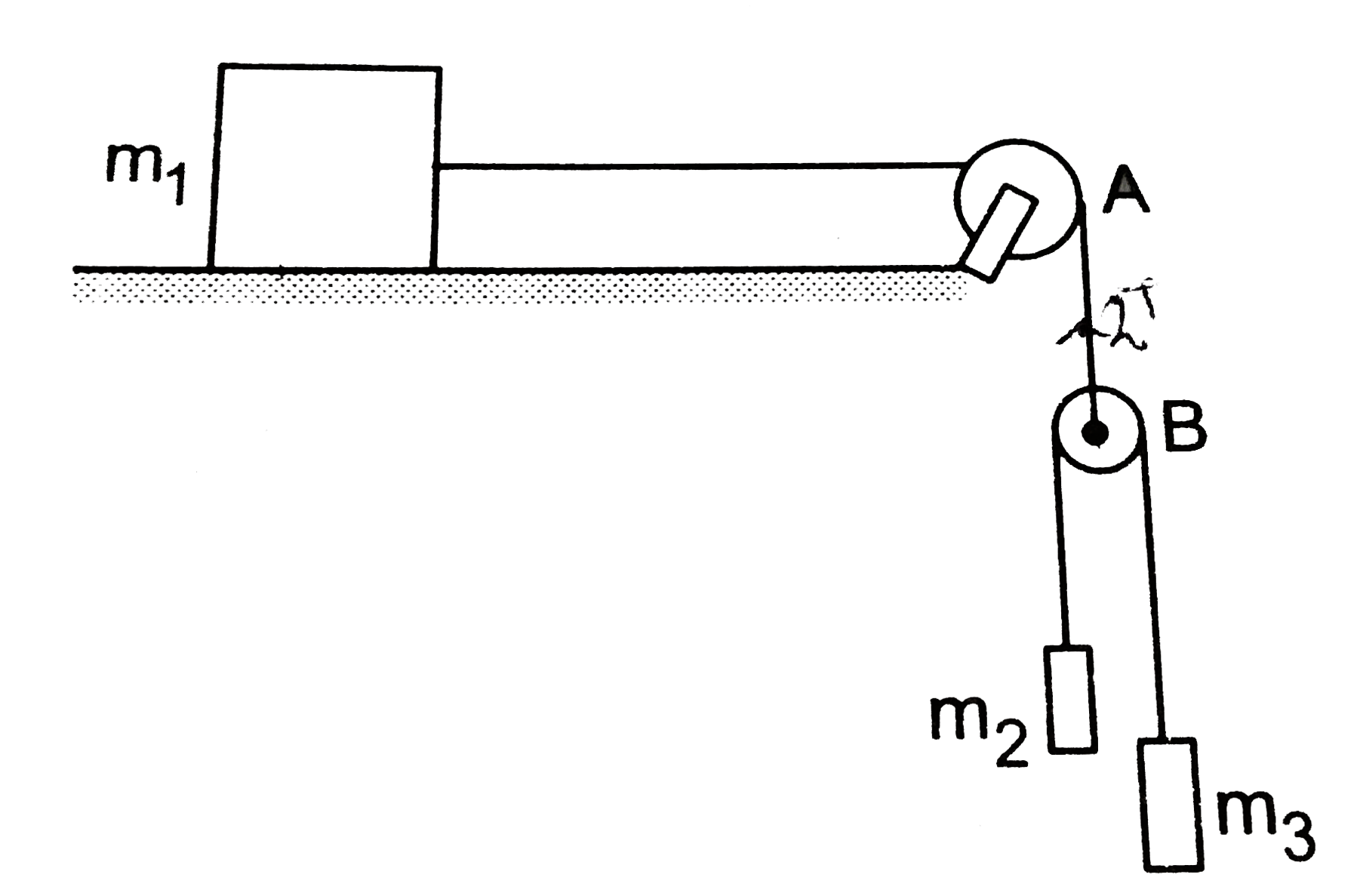
View SolutionThree blocks of masses m1,m2andm3 are connected as shown in the figure. All the surfaces are frictionless and the string and the pulleys are light. Find the acceleration of m1
View Solution