A stone projected at angle θ with horizontal from the roof of a tall building falls on the ground after three seconds. Two second after the projection it was again at the level of projection. Then the height of the building
5m
25 m
20m
15m
The correct Answer is:D
To solve the problem step by step, we need to analyze the motion of the stone projected from the roof of a tall building.
Step 1: Understand the Problem
We know that a stone is projected at an angle θ with the horizontal from the roof of a tall building. It falls to the ground after 3 seconds. It reaches the level of projection again after 2 seconds. We need to find the height of the building (h).
Step 2: Analyze the Motion
The stone is projected with an initial velocity u. We can break this velocity into two components:
- Horizontal component: ucosθ
- Vertical component: usinθ
Step 3: Time of Flight
The total time of flight until the stone hits the ground is given as 3 seconds. The stone returns to the level of projection after 2 seconds. Therefore, the time taken to fall from the level of projection to the ground is:
tfall=3 seconds−2 seconds=1 second
Step 4: Vertical Motion Equations
Using the vertical motion equation, we can express the height of the building (h) in terms of the vertical motion:
h=usinθ⋅ttotal−12gt2fall
Where:
- ttotal=3 seconds (total time of flight)
- tfall=1 second (time taken to fall to the ground)
- g=10m/s2 (acceleration due to gravity)
Step 5: Calculate the Vertical Component
From the information given, we know that the stone reaches the level of projection after 2 seconds. The time taken to reach this level can be expressed as:
tup=2usinθg
Setting this equal to 2 seconds gives:
2=2usinθg⟹usinθ=10m/s
Step 6: Substitute Back to Find Height
Now substituting usinθ=10 into the height equation:
h=10⋅3−12⋅10⋅(12)
Calculating this gives:
h=30−5=25meters
Conclusion
The height of the building is h=25meters.
We know that a stone is projected at an angle θ with the horizontal from the roof of a tall building. It falls to the ground after 3 seconds. It reaches the level of projection again after 2 seconds. We need to find the height of the building (h).
The stone is projected with an initial velocity u. We can break this velocity into two components:
- Horizontal component: ucosθ
- Vertical component: usinθ
The total time of flight until the stone hits the ground is given as 3 seconds. The stone returns to the level of projection after 2 seconds. Therefore, the time taken to fall from the level of projection to the ground is:
tfall=3 seconds−2 seconds=1 second
Using the vertical motion equation, we can express the height of the building (h) in terms of the vertical motion:
h=usinθ⋅ttotal−12gt2fall
Where:
- ttotal=3 seconds (total time of flight)
- tfall=1 second (time taken to fall to the ground)
- g=10m/s2 (acceleration due to gravity)
From the information given, we know that the stone reaches the level of projection after 2 seconds. The time taken to reach this level can be expressed as:
tup=2usinθg
Setting this equal to 2 seconds gives:
2=2usinθg⟹usinθ=10m/s
Now substituting usinθ=10 into the height equation:
h=10⋅3−12⋅10⋅(12)
Calculating this gives:
h=30−5=25meters
The height of the building is h=25meters.
Topper's Solved these Questions
Similar Questions
A body is thrown horizontally from the top of a tower and strikes the ground after three seconds at an angle of 45∘ ,with the horizontal . Then find ,
(i) The height of the tower
View SolutionA body is thrown horizontally from the top of a tower. It reaches the ground after 4s at an angle 45° to the ground. The velocity of projection is
View Solution
Knowledge Check
If a body is projected with an angle θ to the horizontal, then
Aits velocity is always perpendicular to its acceleration thenBits velocity becomes zero at its maximum height.Cits velocity makes zero angle with the horizontal at its maximum height.Dthe body just before hitting the ground, the direction of velocity coincides with the acceleration.
A particle is projected at angle θ with horizontal from ground. The slop (m) of the trajectory of the particle varies with time (t) as
View SolutionA glass marble projected horizontally from the top of a table falls at a distance x from the edge of the table. If h is the height of the table, then the velocity of projection is
View Solution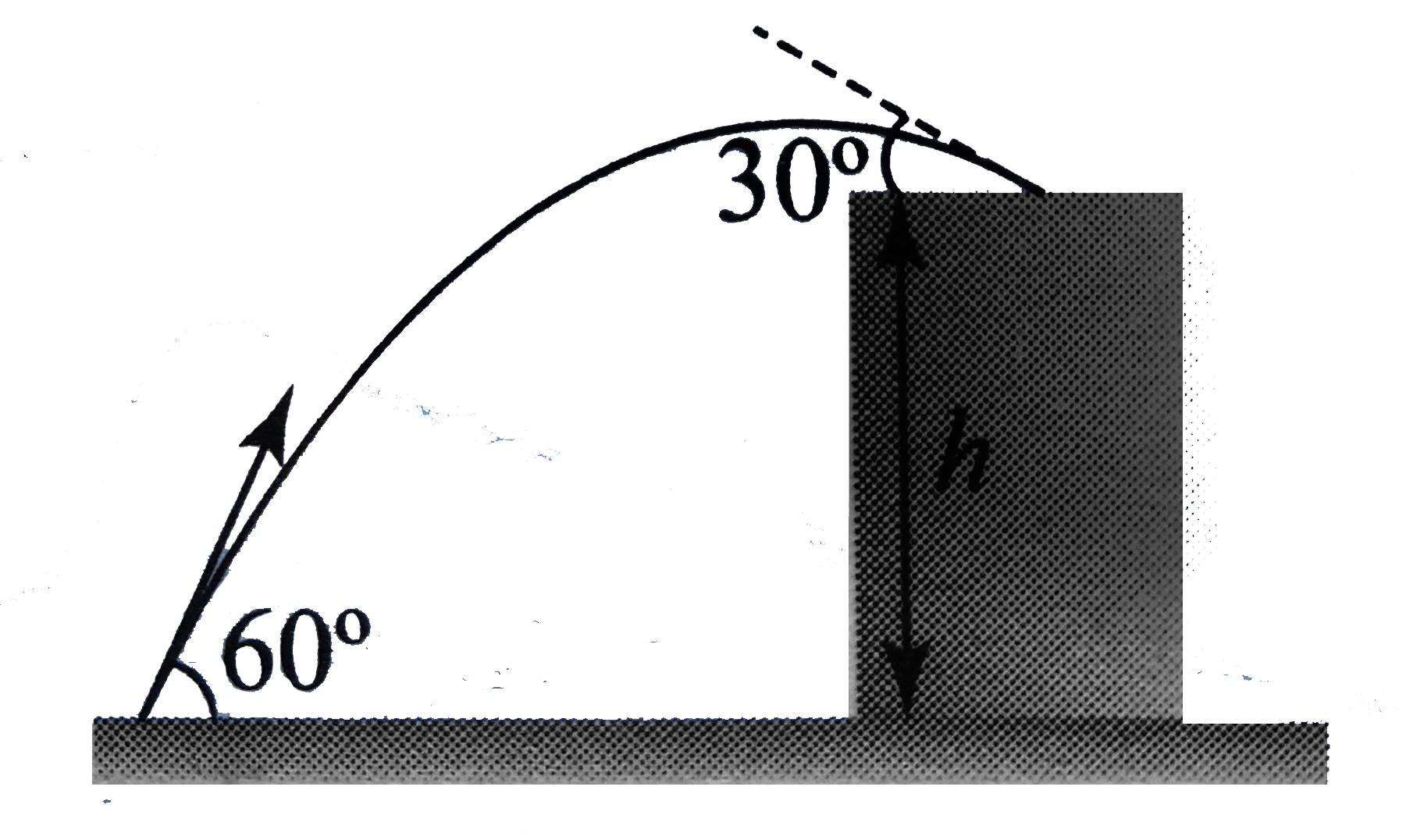
A stone projected at an angle of 60∘ from the ground level strikes at an angle of 30∘ on the roof of a building of height h. Then the speed of projection of the stone is:
View Solution
A stone projected at an angle of 60∘ from the ground level strikes at an angle of 30∘ on the roof of a building of height h. Then the speed of projection of the stone is:
View SolutionA body is thrown horizontally from the top of a tower and strikes the ground after three seconds at an angle of 45∘ ,with the horizontal . Then find ,
(i) The height of the lower
(ii) The speed of projection of the body
View SolutionA particle projected from ground moves at angle 45∘ with horizontal one second after projection and speed is minimum two seconds after the projection The angle of projection of particle is [ Neglect the effect of air resistance )
View SolutionA stone is thrown from the top of a tower at an angle of 30∘ above the horizontal level with a velocity of 40 m/s. it strikes the ground after 5 second from the time of projection then the height of the tower is
View Solution
