Which graph(s) show(s) the correct distribution of potential (at the points between them) due to two like point charges. [X= distance from the charge on left]
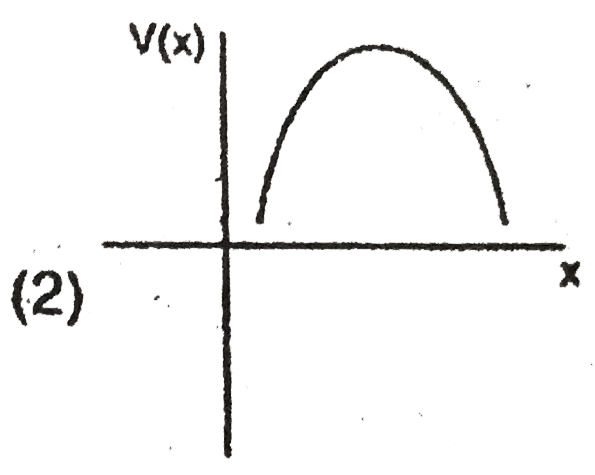


The correct Answer is:C
To determine the correct distribution of electric potential due to two like point charges, we can follow these steps:
Step 1: Understand the Concept of Electric Potential
Electric potential (V) due to a point charge is given by the formula:
V=kQr
where k is Coulomb's constant, Q is the charge, and r is the distance from the charge. For two like charges, both charges will contribute to the total potential at any point in space.
Step 2: Analyze the Configuration
Let's consider two positive point charges, Q1 and Q2, separated by a distance d. We will analyze the potential at a point X which is at a distance x from Q1 and (d−x) from Q2.
Step 3: Write the Expression for Total Potential
The total electric potential Vtotal at point X is the sum of the potentials due to both charges:
Vtotal=V1+V2=kQ1x+kQ2d−x
Since both charges are equal, we can simplify this to:
Vtotal=kQx+kQd−x
Step 4: Analyze the Behavior of the Potential
1. As x approaches 0 (i.e., very close to Q1), Vtotal approaches infinity because 1x becomes very large.
2. As x approaches d (i.e., very close to Q2), Vtotal also approaches infinity because 1d−x becomes very large.
3. At the midpoint x=d2, the potential will be at a local maximum because the contributions from both charges are equal and additive.
Step 5: Sketch the Graph
- The graph of potential V versus distance x will show:
- A steep increase as x approaches 0 from the right.
- A steep increase as x approaches d from the left.
- A maximum at the midpoint x=d2.
Conclusion
The correct graph will show a curve that rises steeply towards infinity as you approach either charge and has a maximum at the midpoint between the two charges.
Electric potential (V) due to a point charge is given by the formula:
V=kQr
where k is Coulomb's constant, Q is the charge, and r is the distance from the charge. For two like charges, both charges will contribute to the total potential at any point in space.
Let's consider two positive point charges, Q1 and Q2, separated by a distance d. We will analyze the potential at a point X which is at a distance x from Q1 and (d−x) from Q2.
The total electric potential Vtotal at point X is the sum of the potentials due to both charges:
Vtotal=V1+V2=kQ1x+kQ2d−x
Since both charges are equal, we can simplify this to:
Vtotal=kQx+kQd−x
1. As x approaches 0 (i.e., very close to Q1), Vtotal approaches infinity because 1x becomes very large.
2. As x approaches d (i.e., very close to Q2), Vtotal also approaches infinity because 1d−x becomes very large.
3. At the midpoint x=d2, the potential will be at a local maximum because the contributions from both charges are equal and additive.
- The graph of potential V versus distance x will show:
- A steep increase as x approaches 0 from the right.
- A steep increase as x approaches d from the left.
- A maximum at the midpoint x=d2.
The correct graph will show a curve that rises steeply towards infinity as you approach either charge and has a maximum at the midpoint between the two charges.
Topper's Solved these Questions
Similar Questions
find the electric field strength due to a point charge of 5μC at a distance of 80 cm from the charge.
View SolutionThe potential at a point 0.1 m from an isolated point charge is + 100 volt. The nature of the point charge is
View SolutionWhich of the following graphs represents the relationship between the force F between two point charges and the distance r between them :
View SolutionPlot a graph comparing the variation of potential ‘V’ and electric field ‘E’ due to a point charge ‘Q’ as a function of distance r from point charge
(ii) Find the ratio of the potential differences that must be applied across the parallel and the series combination of two identical capacitors so that the energy stored, in the two cases, becomes the same.
View SolutionObtain an expression for electric potential .V due to a point charge .Q at a distance r.
View SolutionDetermine the potential at a point 0.50 m (i) from a+20μC potential charge (ii) from a−20μC point charge.
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .

View SolutionSuppose that electric potential due to a small charge configuration varies inversely with with square of distance from the charge distribution . Electric field intensity will vary inversely with what power of distance from the charge configuration in this case ?
View SolutionElectric field intensity at a point in between two parallel sheets with like charges of same surface charge densities (σ) is
View SolutionA point charge q is placed at the origin . How does the electric field due to the charge very with distance r from the origin ?
View Solution