Plane surface of a thin plano-convex lens is silvered. If a point object is placed on principal axis at a distance 60cm from the lens and fial image is formed at a distance 30cm from the lens on same side. What will be the distance of final imagge from the lens if the plane surface is not silvered.
120cm
180cm
30cm
90cm
The correct Answer is:A
To solve the problem step-by-step, let's break it down into manageable parts.
Step 1: Understand the Setup
We have a plano-convex lens with its plane surface silvered. This effectively turns the lens into a mirror for light reflecting off the silvered surface. The object is placed 60 cm from the lens, and the final image is formed 30 cm from the lens on the same side as the object.
Step 2: Use the Mirror Formula
The mirror formula is given by:
1f=1v+1u
where:
- f = focal length of the mirror (which we need to find)
- v = image distance (30 cm, but since it's on the same side as the object, we take it as -30 cm)
- u = object distance (60 cm, taken as -60 cm)
Step 3: Substitute Values into the Mirror Formula
Substituting the values into the mirror formula:
1f=1−30+1−60
Step 4: Calculate the Focal Length
Finding a common denominator (which is 60):
1f=−260−160=−360=−120
Thus, the focal length f is:
f=−20 cm
Step 5: Relate Focal Length of the Lens
For a plano-convex lens, the focal length f is related to the focal length of the silvered lens as:
f′=−f2=−−202=10 cm
This means the effective focal length of the silvered lens is 10 cm.
Step 6: Find the Focal Length of the Unsilvered Lens
The focal length of the unsilvered plano-convex lens is:
f=20 cm
Step 7: Use the Lens Formula for the Unsilvered Lens
Now we will use the lens formula:
1f=1v−1u
Substituting f=20 cm, u=−60 cm:
120=1v−1−60
This simplifies to:
120=1v+160
Step 8: Solve for Image Distance v
Finding a common denominator (which is 60v):
3v60v=360+v60v
This leads to:
3v+2060v=1
Cross-multiplying gives:
3v+20=60v
Rearranging gives:
60v−3v=20⟹57v=20⟹v=2057≈0.35 cm
Final Answer
The distance of the final image from the lens if the plane surface is not silvered is approximately 0.35 cm.
We have a plano-convex lens with its plane surface silvered. This effectively turns the lens into a mirror for light reflecting off the silvered surface. The object is placed 60 cm from the lens, and the final image is formed 30 cm from the lens on the same side as the object.
The mirror formula is given by:
1f=1v+1u
where:
- f = focal length of the mirror (which we need to find)
- v = image distance (30 cm, but since it's on the same side as the object, we take it as -30 cm)
- u = object distance (60 cm, taken as -60 cm)
Substituting the values into the mirror formula:
1f=1−30+1−60
Finding a common denominator (which is 60):
1f=−260−160=−360=−120
Thus, the focal length f is:
f=−20 cm
For a plano-convex lens, the focal length f is related to the focal length of the silvered lens as:
f′=−f2=−−202=10 cm
This means the effective focal length of the silvered lens is 10 cm.
The focal length of the unsilvered plano-convex lens is:
f=20 cm
Now we will use the lens formula:
1f=1v−1u
Substituting f=20 cm, u=−60 cm:
120=1v−1−60
This simplifies to:
120=1v+160
Finding a common denominator (which is 60v):
3v60v=360+v60v
This leads to:
3v+2060v=1
Cross-multiplying gives:
3v+20=60v
Rearranging gives:
60v−3v=20⟹57v=20⟹v=2057≈0.35 cm
The distance of the final image from the lens if the plane surface is not silvered is approximately 0.35 cm.
Topper's Solved these Questions
Similar Questions
The plane surface of a plano-convex lens of focal length 60 cm is silvered. A point object is placed at a distance 20 cm from the lens. Find the position and nature of the final image formed.
View SolutionThe plane surface of a plano-convex lens of focal length f is silvered. It will behave as
View SolutionA convex lens produces a real image m times the size of the object. What will be the distance of the object from the lens ?
View SolutionA plano-convex lens P and a concavo-convex lens Q are in contact as shown in figure. The refractive index o fthe material of the lens P and Q is 1.8and1.2 respectively. The radius of curvature of the concave surface of the lens Q is double the radius of curvature of the convex surface. The convex surface of Q is silvered.

An object is placed on the placed on the principal axis at a distance 10cm form the plane surface. The image is formed at a distance 40cm from the plane surfaces on the same side. The focal length of the system isView SolutionAn object is put at a distance of 5cm from the first focus of a convex lens of focal length 10cm. If a real image is formed, its distance from the lens will be
View SolutionAn object is placed at a distance 24 cm in front of a convex lens of focal length 8 cm.
Calculate the distance of the image from the lens.View SolutionAn object is placed at a distance of f/2 from a convex lens. The image will be
View SolutionAn object is kept at a distance of 4 cm form the first focus of a convex lens. A real image is formed at a distance of 9 cm from its second focus. What is the focal length of that lens is ___________(in cm)
View SolutionFind distanace of image from a convex lens of focal length
20cm if object is placed at a distance of 30cm from the lens. Also find its
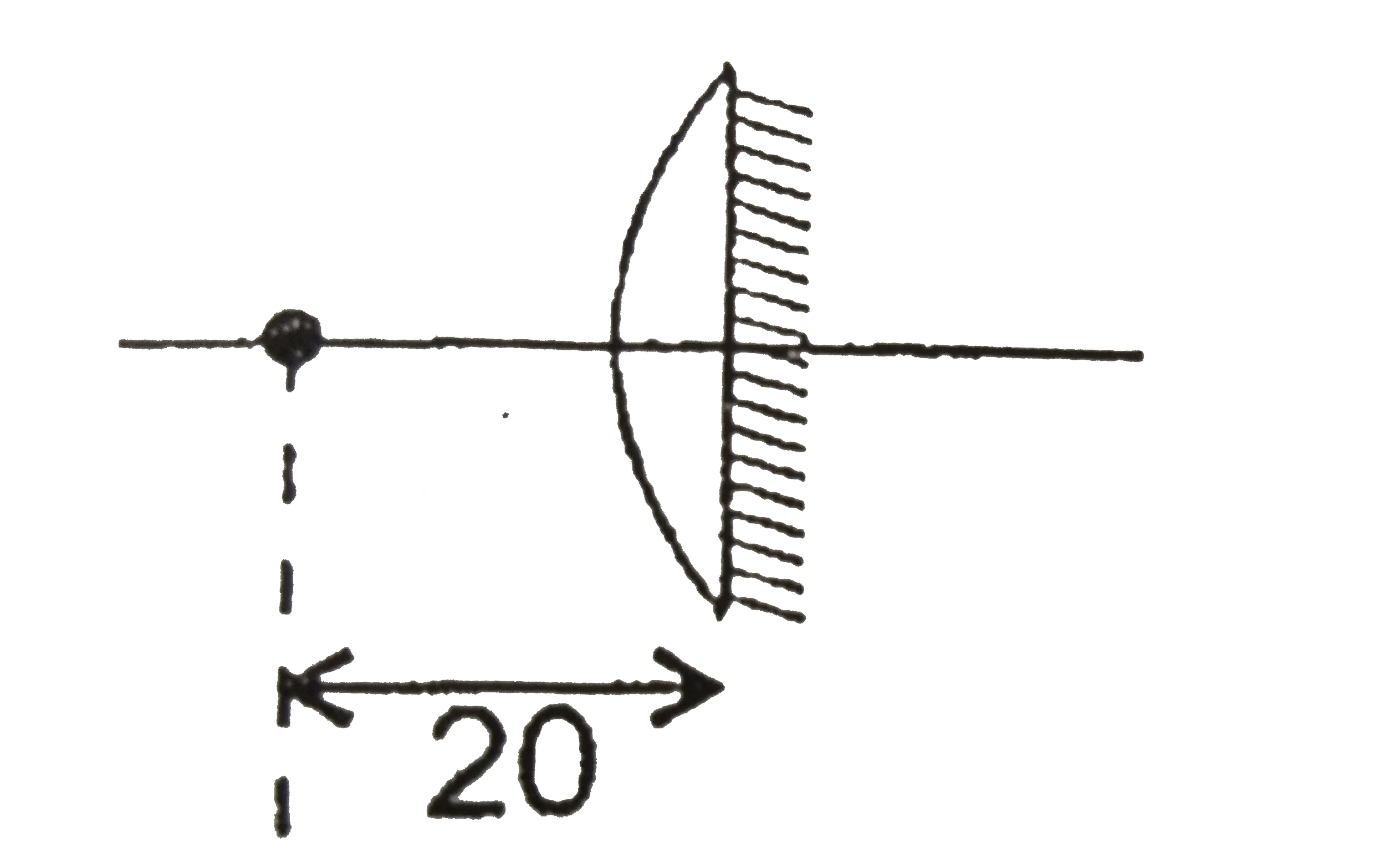
magnification.View SolutionA point object is placed at a distance of 20cm from a thin plano-concex lens of focal length 15cm. The plane surface of the lens is now silvered. The image created by the is at :
 View Solution
View Solution

