Electric field at a point of distance r from a uniformly charged wire of infinite length having linear charge density λ is directly proportional to
r−1
r
r2
r−2
The correct Answer is:A
To solve the problem of finding the relationship between the electric field E at a distance r from a uniformly charged wire of infinite length with linear charge density λ, we can follow these steps:
Step 1: Understand the Problem
We need to determine how the electric field E behaves with respect to the distance r from an infinitely long charged wire.
Hint: Recall that the electric field due to a charged object can be derived using Gauss's Law.
Step 2: Set Up the Gaussian Surface
To apply Gauss's Law, we consider a cylindrical Gaussian surface that surrounds the charged wire. Let the cylinder have:
- Length L
- Radius r
Hint: The symmetry of the problem suggests that the electric field will be uniform and directed radially outward from the wire.
Step 3: Apply Gauss's Law
Gauss's Law states:
ΦE=Qencϵ0
Where ΦE is the electric flux through the Gaussian surface and Qenc is the charge enclosed by the surface.
Hint: The electric flux ΦE can be expressed as ∫E⋅dA.
Step 4: Calculate the Electric Flux
The electric flux through the curved surface of the cylinder is given by:
ΦE=E⋅A
Where A is the surface area of the cylindrical side, which is 2πrL. Therefore:
ΦE=E⋅(2πrL)
Hint: Remember that the electric field E is constant over the curved surface of the cylinder.
Step 5: Determine the Charge Enclosed
The total charge enclosed by the Gaussian surface is:
Qenc=λ⋅L
Where λ is the linear charge density.
Hint: Linear charge density λ is defined as charge per unit length.
Step 6: Substitute into Gauss's Law
Substituting the expressions for electric flux and charge into Gauss's Law gives:
E⋅(2πrL)=λLϵ0
Step 7: Solve for Electric Field E
Now, we can solve for E:
E=λ2πϵ0r
Hint: Notice how E depends on r.
Step 8: Identify the Relationship
From the equation E=λ2πϵ0r, we see that:
E∝1r
This means that the electric field E is inversely proportional to the distance r.
Conclusion
Thus, the electric field at a point of distance r from a uniformly charged wire of infinite length having linear charge density λ is directly proportional to r−1.
Final Answer: The electric field is proportional to r−1.
We need to determine how the electric field E behaves with respect to the distance r from an infinitely long charged wire.
To apply Gauss's Law, we consider a cylindrical Gaussian surface that surrounds the charged wire. Let the cylinder have:
- Length L
- Radius r
Gauss's Law states:
ΦE=Qencϵ0
Where ΦE is the electric flux through the Gaussian surface and Qenc is the charge enclosed by the surface.
The electric flux through the curved surface of the cylinder is given by:
ΦE=E⋅A
Where A is the surface area of the cylindrical side, which is 2πrL. Therefore:
ΦE=E⋅(2πrL)
The total charge enclosed by the Gaussian surface is:
Qenc=λ⋅L
Where λ is the linear charge density.
Substituting the expressions for electric flux and charge into Gauss's Law gives:
E⋅(2πrL)=λLϵ0
Now, we can solve for E:
E=λ2πϵ0r
From the equation E=λ2πϵ0r, we see that:
E∝1r
This means that the electric field E is inversely proportional to the distance r.
Thus, the electric field at a point of distance r from a uniformly charged wire of infinite length having linear charge density λ is directly proportional to r−1.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Match the columns
5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
Let E1(r), E2(r) and E3(r) be the respectively electric field at a distance r from a point charge Q, an infinitely long wire with constant linear charge density λ, and an infinite plane with uniform surface charge density σ. If E1(r0)=E2(r0)=E3(r0) at a given distance r0, then
View SolutionIntensity of electric field at a point at a perpendicular distance .r. from an infinite line charge, having linear charge density .λ. is given by :
View SolutionIntensity of electric field at a point at a perpendicular distance .r. from an infinite line charge, having linear charge density .λ. is given by :
View SolutionFind the electric field at the centre of a uniformly charged semicircular ring of radius R. Linear charge density is λ
View SolutionA charge particle q is released at a distance R∘ from the infinite long wire of linear charge density λ. Then velocity will be proportional to (At distance R from the wire)
_2019_CBT2_E01_009_Q01.png)
View SolutionWhat is the electric field intensity at any point on the axis of a charged rod of length 'L' and linear charge density λ? The point is separated from the nearer end by a.
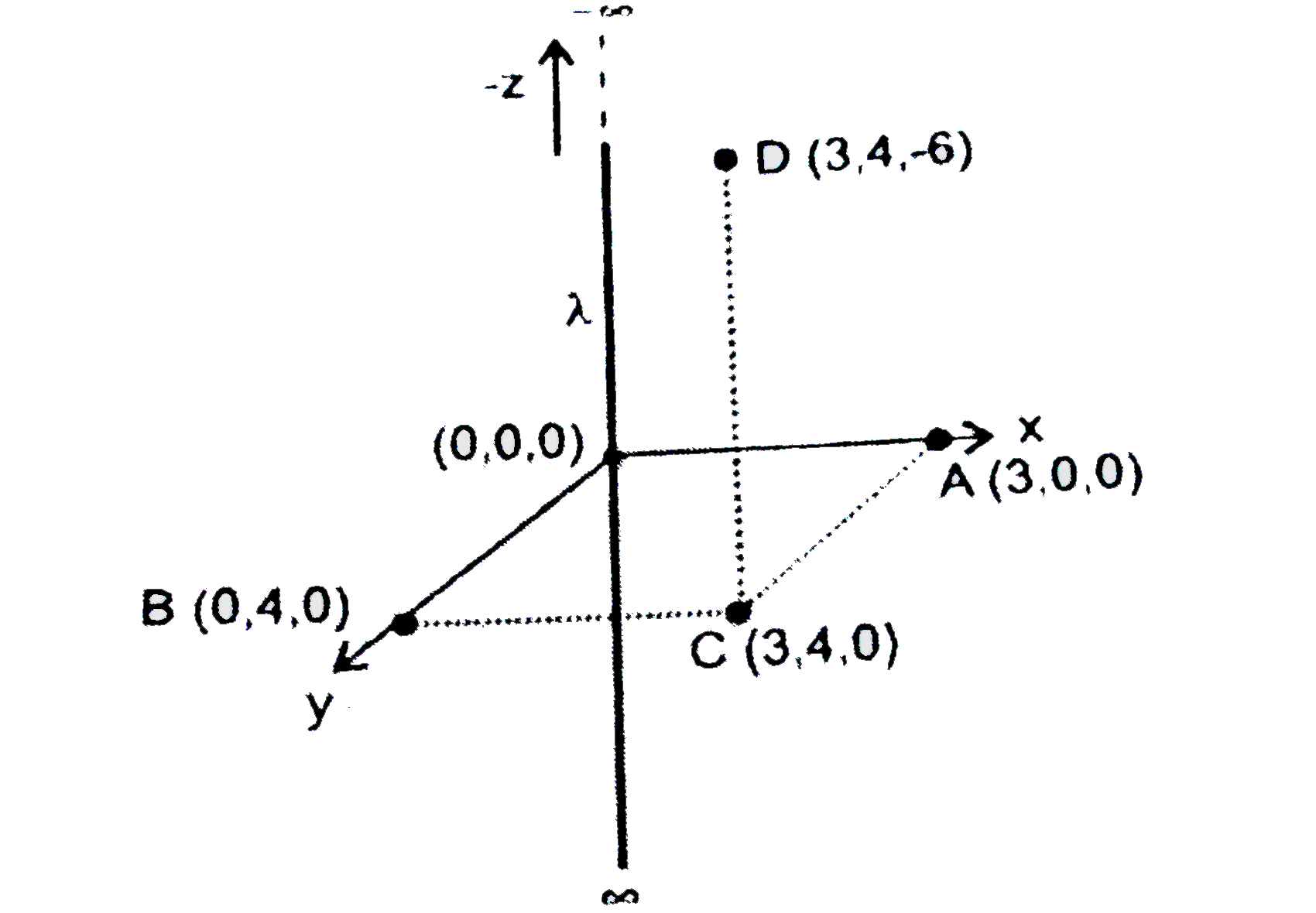
View SolutionFind electric field at point A, B, C, D due to infinitely long uniformly charged wire with linear charge density λ and kept along z-axis (as shown in figure). Assume that all the parameters are in S.l. units.
View SolutionIntensity of electric field at a perpendicular distance of 0.5 m from an infinitely long line charge having linear charge density (λ) is 3.6×103Vm−1 . Find the value of λ
View SolutionFind the electric field due to an infinitely long cylindrical charge distribution of radius R and having linear charge density λ at a distance half of the radius from its axis.
View SolutionFind the electric field at a point P on the perpendicular bisector of a uniformly charged rod. The length of the rod is L, the charge on it is Q and the distance of P from the centre of the rod is a.
View Solution
