A particle of mass and charge is released from rest in a uniform electric field. If there is no other force on the particle, the dependence of its kinetic energy on the distance travelled by it is correctly given by (graphs are schematic and not drawn to scale)
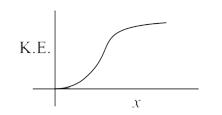
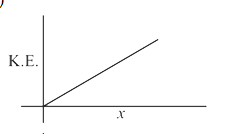
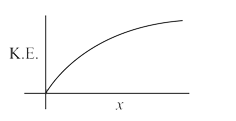
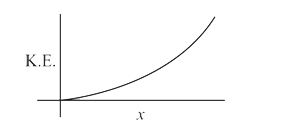
The correct Answer is:B
To solve the problem, we need to analyze the motion of a charged particle in a uniform electric field and determine how its kinetic energy depends on the distance traveled.
1. Identify the Forces Acting on the Particle:
- The particle has a mass m and charge q.
- It is placed in a uniform electric field E.
- The force acting on the particle due to the electric field is given by:
F=qE
2. Apply Newton's Second Law:
- According to Newton's second law, the net force acting on the particle is equal to the mass of the particle multiplied by its acceleration a:
F=ma
- Therefore, we can equate the two expressions for force:
ma=qE
- From this, we can express the acceleration a:
a=qEm
3. Use Kinematic Equations to Find Velocity:
- Since the particle starts from rest, its initial velocity u=0.
- We can use the kinematic equation that relates velocity, initial velocity, acceleration, and displacement:
v2=u2+2as
- Substituting u=0 and a=qEm, we have:
v2=0+2(qEm)x
- This simplifies to:
v2=2qEmx
4. Calculate the Kinetic Energy:
- The kinetic energy K of the particle is given by:
K=12mv2
- Substituting the expression for v2:
K=12m(2qEmx)
- Simplifying this, we find:
K=qEx
5. Determine the Relationship Between Kinetic Energy and Distance:
- The expression K=qEx shows that the kinetic energy K is directly proportional to the distance x traveled by the particle.
- This indicates a linear relationship, meaning that as the distance increases, the kinetic energy increases linearly.
Conclusion:
The dependence of the kinetic energy on the distance traveled by the particle is linear, and the correct option is that kinetic energy K is proportional to x.
- The particle has a mass m and charge q.
- It is placed in a uniform electric field E.
- The force acting on the particle due to the electric field is given by:
F=qE
- According to Newton's second law, the net force acting on the particle is equal to the mass of the particle multiplied by its acceleration a:
F=ma
- Therefore, we can equate the two expressions for force:
ma=qE
- From this, we can express the acceleration a:
a=qEm
- Since the particle starts from rest, its initial velocity u=0.
- We can use the kinematic equation that relates velocity, initial velocity, acceleration, and displacement:
v2=u2+2as
- Substituting u=0 and a=qEm, we have:
v2=0+2(qEm)x
- This simplifies to:
v2=2qEmx
- The kinetic energy K of the particle is given by:
K=12mv2
- Substituting the expression for v2:
K=12m(2qEmx)
- Simplifying this, we find:
K=qEx
- The expression K=qEx shows that the kinetic energy K is directly proportional to the distance x traveled by the particle.
- This indicates a linear relationship, meaning that as the distance increases, the kinetic energy increases linearly.
The dependence of the kinetic energy on the distance traveled by the particle is linear, and the correct option is that kinetic energy K is proportional to x.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 27 - JEE -2020EXERCISE - PHYSICS (SECTION 2)5videos
JEE MAIN REVISION TEST - 27 - JEE -2020
- BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 26 (2020)EXERCISE - PHYSICS (SECTION 2)5videos
JEE MAIN REVISION TEST - 26 (2020)
- BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 28EXERCISE - PHYSICS (SECTION-2)5videos
JEE MAIN REVISION TEST - 28
Similar Questions
A particle of mass m and charge −q is released from rest in a uniform electric field. If there is no other force on the particle, the dependence of its potential energy on the distance x travelled by it is correctly given by (assume initial potential energy to be zero ,graphs are schematic and not drawn to scale)
View SolutionA particle of mass m and charge −q is released from rest in a uniform electric field. If there is no other force on the particle, the dependence of its potential energy on the distance x travelled by it is correctly given by (assume initial potential energy to be zero ,graphs are schematic and not drawn to scale)
View Solution
Knowledge Check
- Question 1 - Select One
A positively charged particle is released from rest in a uniform electric field. The electric potential energy of the charge.
Aremains a constant because the electric field is uniform.Bincreases because the charge moves along the electric field.Cdecreases because the charge moves along the electric field.Ddecreases because the charge moves opposite to the electric field.
A particle of mass m and charge q is released from rest in uniform electric field of intensity E. Calculate the kinetic energy it attains after moving a distance y between the plates.
View SolutionA charge particle of mass m and charge q is released from rest in uniform electric field. Its graph between velocity (v) and distance travelled (x) will be :
View SolutionA charge particle of mass m and charge q is released from rest in uniform electric field. Its graph between velocity (v) and distance travelled (x) will be :
View SolutionA charged particle of mass m and charge q is released from rest in an electric field of constant magnitude E. The kinetic energy of the particle after time t is
View SolutionA positively charged particle is released from rest in a uniform electric field. The electric potential energy of the charge.
View SolutionA positively charged particle is released from rest in a uniform electric field. The electric potential energy of the charge.
View SolutionA particle of mass .m. and charge q is placed at rest in a uniform electric field E and then released. The K.E. attained by the particle after moving a distance y is
View Solution

