If the lengths of the sides of a triangle are 8, 15 and 17 units, the R the circum-radius is :
9.0
8.5
8.0
7.5
The correct Answer is:B
Step by step video & image solution for If the lengths of the sides of a triangle are 8, 15 and 17 units, the R the circum-radius is : by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES OF TRIANGLEBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF TRIANGLEEXERCISE - Level - 2
55 Videos
- PROPERTIES OF TRIANGLEBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF TRIANGLEEXERCISE - JEE Main (Archive)
35 Videos
- PROBABILITYBOOK - VMC MODULES ENGLISHCHAPTER - PROBABILITYEXERCISE - JEE ADVANCED (ARCHIVE)
102 Videos
- QUADRATIC EQUATIONS & INEQUATIONS BOOK - VMC MODULES ENGLISHCHAPTER - QUADRATIC EQUATIONS & INEQUATIONS EXERCISE - JEE Advance ( Archive )
20 Videos
Similar Questions
If the lengths of the side of a triangle are 3,4 and 5 units, then find the circum radius R.
View SolutionFind the length of the third side of the triangle.
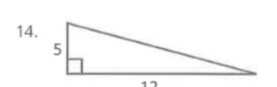
View SolutionFind the length of the third side of the triangle.
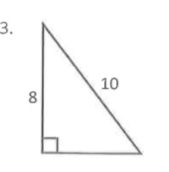
View SolutionIf the lengths of the sides of a triangle are in AP and the greatest angle is double the smallest, then a ratio of lengths of the sides of this triangle is
View SolutionThe sides of triangle are in A.P. and the greatest angle exceeds the least by 90∘. The sides are in the ratio:
View SolutionIf area of a triangle is 2 sq. units, then find the value of the product of the arithmetic mean of the lengths of the sides of a triangle and harmonic mean of the lengths of the altitudes of the triangle.
View SolutionIf area of a triangle is 2 sq. units, then find the value of the product of the arithmetic mean of the lengths of the sides of a triangle and harmonic mean of the lengths of the altitudes of the triangle.
View SolutionThe sides of a triangle are 13,14,15, then the radius of its in-circle is
View SolutionThe lengths of two sides of a triangle are 12 cm and 15 cm. Between what two measures should the length of the third side fall?
View SolutionIf the sides of a triangle are in the ratio 3:7:8, then find R:r
View Solution
