l1andl2 are the side lengths of two variable squares S1,andS2, respectively. If l1=l2+l32+6 then rate of change of the area of S2, with respect to rate of change of the area of S1 when l2=1 is equal to
34
43
32
132
The correct Answer is:C
To solve the problem, we need to find the rate of change of the area of square S2 with respect to the area of square S1 when l2=1.
1. Define the areas of the squares:
- The area of square S1 is given by:
A1=l21
- The area of square S2 is given by:
A2=l22
2. Express l1 in terms of l2:
- From the problem statement, we have:
l1=l2+l32+6
3. Differentiate the areas with respect to time t:
- Differentiate A1:
dA1dt=2l1dl1dt
- Differentiate A2:
dA2dt=2l2dl2dt
4. Differentiate l1:
- Differentiate l1 with respect to t:
dl1dt=ddt(l2+l32+6)=dl2dt+3l22dl2dt
- This can be simplified to:
dl1dt=(1+3l22)dl2dt
5. Substitute l1 and l2 into the derivatives:
- Substitute l2=1:
l1=1+13+6=8
- Now substitute l2=1 into the derivatives:
dA1dt=2⋅8⋅(1+3⋅12)dl2dt=2⋅8⋅4dl2dt=64dl2dt
dA2dt=2⋅1⋅dl2dt=2dl2dt
6. Find the ratio of the rates of change of areas:
- The ratio dA2dA1 is given by:
dA2dA1=2dl2dt64dl2dt=264=132
Final Answer:
The rate of change of the area of S2 with respect to the area of S1 when l2=1 is:
132
- The area of square S1 is given by:
A1=l21
- The area of square S2 is given by:
A2=l22
- From the problem statement, we have:
l1=l2+l32+6
- Differentiate A1:
dA1dt=2l1dl1dt
- Differentiate A2:
dA2dt=2l2dl2dt
- Differentiate l1 with respect to t:
dl1dt=ddt(l2+l32+6)=dl2dt+3l22dl2dt
- This can be simplified to:
dl1dt=(1+3l22)dl2dt
- Substitute l2=1:
l1=1+13+6=8
- Now substitute l2=1 into the derivatives:
dA1dt=2⋅8⋅(1+3⋅12)dl2dt=2⋅8⋅4dl2dt=64dl2dt
dA2dt=2⋅1⋅dl2dt=2dl2dt
- The ratio dA2dA1 is given by:
dA2dA1=2dl2dt64dl2dt=264=132
The rate of change of the area of S2 with respect to the area of S1 when l2=1 is:
132
Topper's Solved these Questions
- DIFFERENTIAL CALCULUS 2BOOK - VMC MODULES ENGLISHCHAPTER - DIFFERENTIAL CALCULUS 2EXERCISE - Numerical ValueType for JEE Main
14 Videos
- DIFFERENTIAL CALCULUS 2BOOK - VMC MODULES ENGLISHCHAPTER - DIFFERENTIAL CALCULUS 2EXERCISE - JEE Main (Archive)
36 Videos
- DIFFERENTIAL CALCULUS 2BOOK - VMC MODULES ENGLISHCHAPTER - DIFFERENTIAL CALCULUS 2EXERCISE - Level -1
102 Videos
- DIFFERENTIAL CALCULUSBOOK - VMC MODULES ENGLISHCHAPTER - DIFFERENTIAL CALCULUSEXERCISE - JEE Advanced (Archive)
75 Videos
- DIFFERENTIAL EQUATIONSBOOK - VMC MODULES ENGLISHCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - JEE ADVANCE (ARCHIVE )
32 Videos
Similar Questions
θ1andθ2 are the inclination of lines L1andL2 with the x-axis. If L1andL2 pass through P(x1,y1), then the equation of one of the angle bisector of these lines is
View SolutionWe have two (narrow) capillary tubes T1 and T2 . Their lengths are l1 and l2 and radii of cross-section are r1 and r2 respectively. The rate of flow of water under a pressure difference P through tube T is 8cm3/sec. If l1=2l2 and r1=r2 what will be the rate of flow when the two tubes are connected in series and pressure difference across the combinatin is same as before (=P)
View SolutionThe length of a metal wire is l1 when the tension in it is T1 and is l2 when the tension is T2. The natural length of the wire is
View SolutionThe length of a metal wire is l1 when the tension in it is T1 and is l2 when the tension is T2. The natural length of the wire is
View SolutionThe length of a metal wire is l1 when the tensionin it is T1andisl2 when the tension is T2. The natural length of the wire is
View SolutionLet l1andl2 be the two skew lines. If P, Q are two distinct points on l1 and R, S are two distinct points on l2, then prove that PR cannot be parallel to QS.
View SolutionTwo plates eachb of area A, thickness L1andL2 thermal conductivities K1andK2 respectively are joined to form a single plate of thickness (L1+L2). If the temperatures of the free surfaces are T1andT2. Calculate.
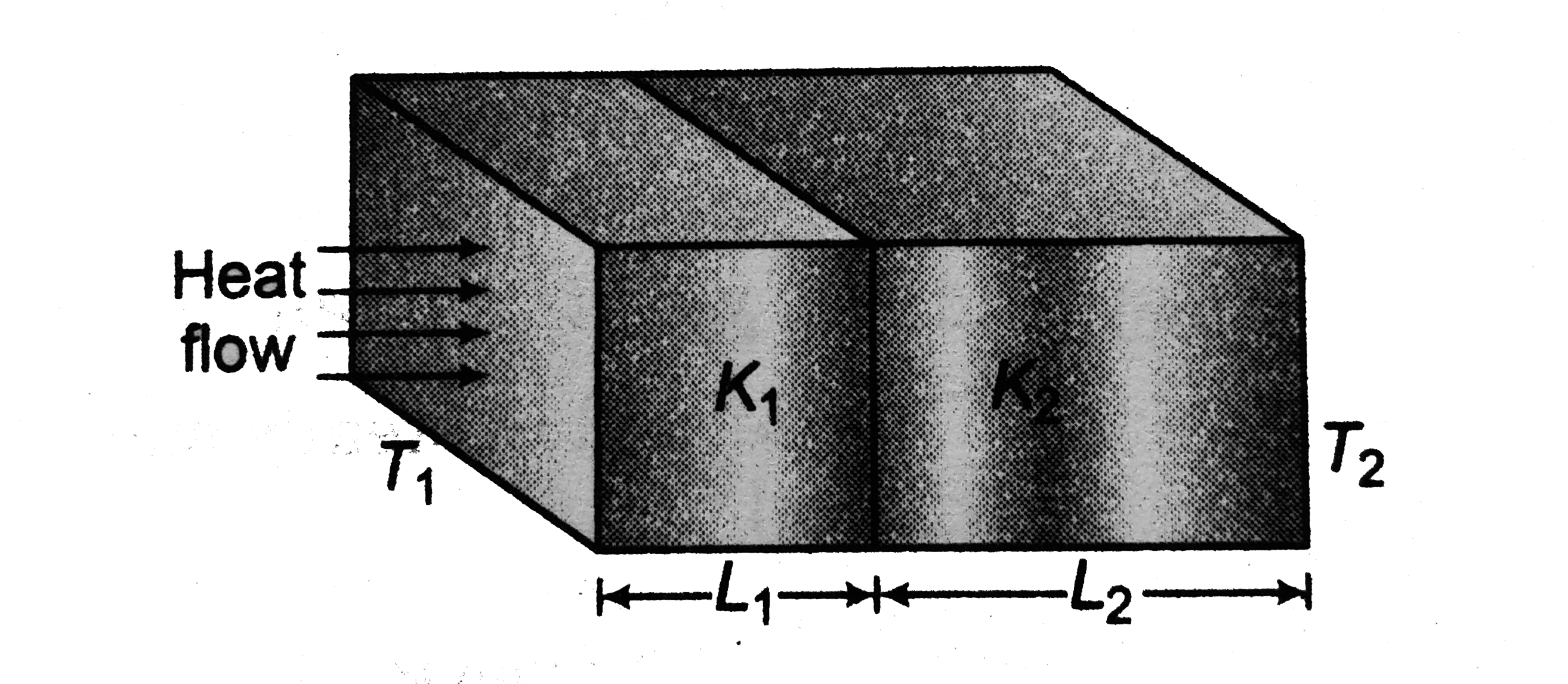
(a) rate of flow of heat
(b) temperature of interface and
(c) equivalent thermal conductivity.
View SolutionLet l1 and l2 be the two lines which are normal to y2=4x and tangent to x2=−12y respectively (where, l1andl2 are not the x - axis). Then, the product of the slopes of l1andl2 is
View SolutionTwo rods, one of aluminium and the other made of steel, having initial length l1andl2 are connected together to from a single rod of length l1+l2. The coefficients of linear expansion for aluminium and steel are αaandαs and respectively. If the length of each rod increases by the same amount when their temperature are raised by t0C, then find the ratio l1/(l1+l2)
View SolutionTwo rods, one of aluminium and the other made of steel, having initial length l1andl2 are connected together to from a single rod of length l1+l2. The coefficients of linear expansion for aluminium and steel are αaandαs and respectively. If the length of each rod increases by the same amount when their temperature are raised by t0C, then find the ratio l1/(l1+l2)
View Solution
