Two uniform soild spheres of equal radii R but mass M and 4M have a centre to centre separation 6R, as shows in Fig. (a) The two spheres are held fixed. A projectile of massm is projected from the surface of the sphere of mass M directly towards the centre of teh second. Obtain an expression for the minimum speed υ of the projectile so that it reaches the surface of second sphere.
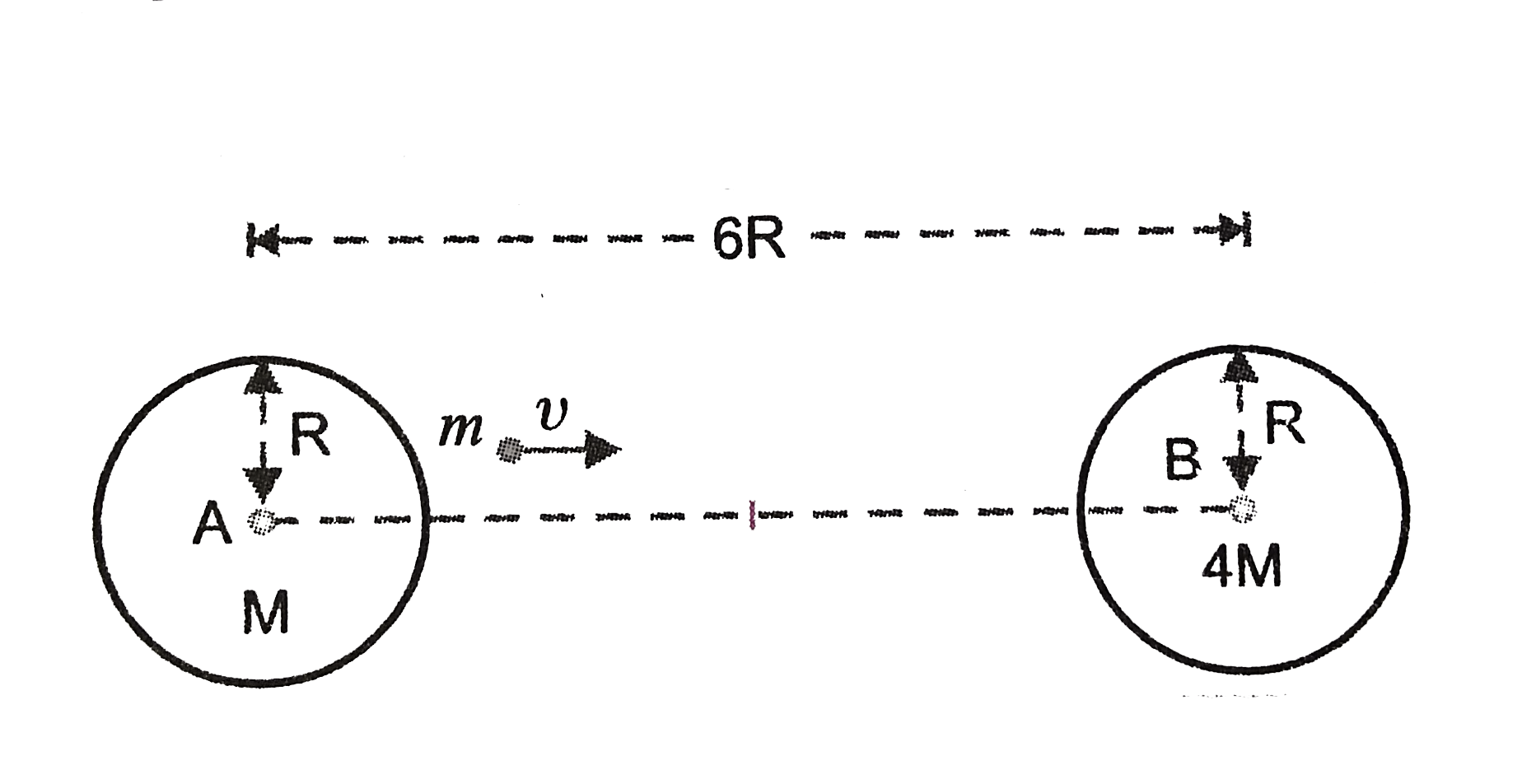
The two spheres exert gravitational forces on the projectile in mutually opposite directions. At the neutral point N, there two forces cancel each other. If ON = r, then
GMmr2=G(4M)m(6R−r)2 or (6R−r)2=4r2⇒6R−r=±2r or r=2R or -6R
The neutral point r = – 6R is inadmissible.
∴ ON=r=2 R
It will be sufficient to project the particle m with a minimum speed v which enables it to reach the point N.
Therefore, the particle m gets attracted by the gravitational pull of 4 M.
The total mechanical energy of m at surface of left sphere is Ei=KE of m+ PE due to left sphere + PE due to right sphere
=12mv2−GMmR−4GMm5R
At the neutral point, speed of the particle becomes zero. The energy is purely potential.
∴EN=PE due to left sphere + PE due to right sphere =−GMm2R−4GMm4R
By conservation of mechanical energy, Ei=EN
Or 12mv2−GMmR−4GMm5R=−GMm2R−4GMm4R or v2=2GMR(45−12)=3GM5R∴v=√3GM5R
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level-1 MCQs60videos
GRAVITATION
- BOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level-230videos
GRAVITATION
- BOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - LEVEL -0 Short Answer Type – II13videos
GRAVITATION
- BOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )111videos
GASEOUS STATE & THERMODYNAMICS
- BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Advanced ( ARCHIVE LEVEL-2)12videos
INTRODUCTION TO VECTORS & FORCES
Similar Questions
Two solid spherical planets of equal radii R having masses 4M and 9M their centre are separated by a distance 6R. A projectile of mass m is sent from theplanet of mass 4 M towards the heavier planet. What is the distance r of the point from the lighter planet where the gravitational force on the projectille is zero ?
View SolutionA particle of mass m is lying at the centre of a solid sphere of mass M and radius R. There is a turnel of negligible thickness, so that particle may escape. Find the minimum velocity required to escape the particle from the gravitational field of the sphere.
View Solution
Knowledge Check
- Question 1 - Select One
Two spheres each of mass M and radius R are separated by a distance of r. The gravitational potential at the midpoint of the line joining the centres of the spheres is
A−GMrB−2GMrC−GM2rD−4GMr
The gravitational field due to an uniform solid sphere of mass M and radius a at the centre of the sphere is
View SolutionA body of mass m is placed at the centre of the spherical shell of radius R and mass M. The gravitation potential on the surface of the shell is
View SolutionTwo planets have masses M and 16 M and their radii are a and 2a, respectively. The separation between the centres of the planets is 10a. A body of mass m is fired trom the surface of the larger planet towards the samller planet along the line joining their centres. For the body to be able to reach at the surface of smaller planet, the minimum firing speed needed is :
View SolutionThree solid spheres each of mass m and radius R are released from the position shown in Fig. What is the speed of any one sphere at the time of collision?
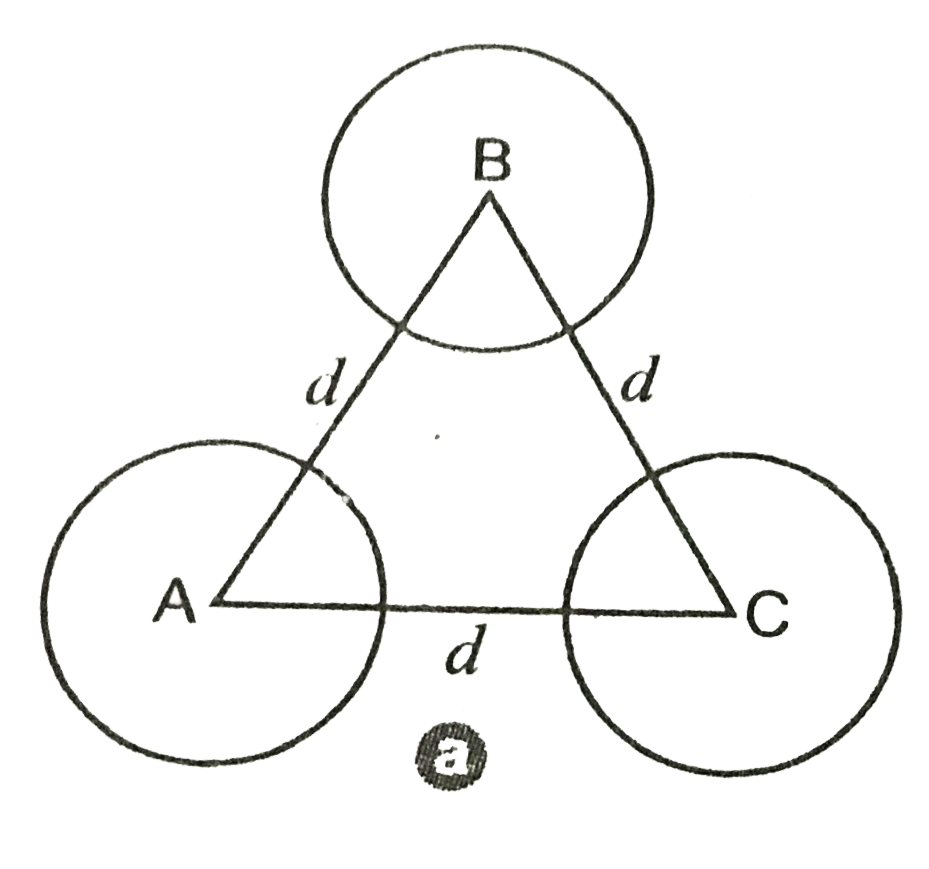 View Solution
View SolutionTwo spheres each of mass M and radius R are separated by a distance of r. The gravitational potential at the midpoint of the line joining the centres of the spheres is
View SolutionA cavity of radius R/2 is made inside a solid sphere of radius R. The centre of the cavity is located at a distance R/2 from the centre of the sphere. The gravitational force on a particle of a mass 'm' at a distance R/2 from the centre of the sphere on the line joining both the centres of sphere and cavity is (opposite to the centre of cavity). [Here g=GM/R2, where M is the mass of the solide sphere]
View SolutionA cavity of radius R/2 is made inside a solid sphere of radius R. The centre of the cavity is located at a distance R/2 from the centre of the sphere. The gravitational force on a particle of a mass 'm' at a distance R/2 from the centre of the sphere on the line joining both the centres of sphere and cavity is (opposite to the centre of cavity). [Here g=GM/R2, where M is the mass of the solide sphere]
View Solution

