Two planets have masses M and 16 M and their radii are a and 2a, respectively. The separation between the centres of the planets is 10a. A body of mass m is fired trom the surface of the larger planet towards the samller planet along the line joining their centres. For the body to be able to reach at the surface of smaller planet, the minimum firing speed needed is :
S
4√GMa
√GM2ma
32√5GMa
To solve the problem, we need to determine the minimum firing speed required for a body of mass m to reach the surface of a smaller planet from the surface of a larger planet. Let's break down the solution step by step.
Step 1: Understand the System
We have two planets:
- Planet 1 (larger): Mass = 16M, Radius = 2a
- Planet 2 (smaller): Mass = M, Radius = a
The distance between the centers of the two planets is 10a.
Step 2: Determine the Gravitational Fields
The gravitational field g due to a planet at a distance r from its center is given by:
g=GMr2
Where G is the gravitational constant.
Step 3: Find the Point Where Gravitational Fields are Equal
Let x be the distance from the center of the smaller planet where the gravitational fields due to both planets are equal. The distance from the center of the larger planet to this point is 10a−x.
Setting the gravitational fields equal:
GMx2=G(16M)(10a−x)2
Cancelling G and M from both sides:
1x2=16(10a−x)2
Cross-multiplying gives:
(10a−x)2=16x2
Step 4: Solve for x
Expanding and rearranging:
100a2−20ax+x2=16x2
100a2−20ax−15x2=0
This is a quadratic equation in x:
15x2+20ax−100a2=0
Using the quadratic formula x=−b±√b2−4ac2a:
Here, a=15, b=20a, and c=−100a2:
x=−20a±√(20a)2−4⋅15⋅(−100a2)2⋅15
x=−20a±√400a2+6000a230
x=−20a±√6400a230
x=−20a±80a30
Taking the positive root:
x=60a30=2a
Step 5: Calculate the Potential Energy at Different Points
1. At the surface of the larger planet (distance = 8a):
U1=−G(16M)m8a−G(M)m2a
2. At the point where gravitational fields are equal (distance = 2a):
U2=−G(M)m2a−G(16M)m8a
Step 6: Apply Conservation of Energy
The total mechanical energy at the starting point equals the total mechanical energy at the point where the gravitational fields are equal:
K.E.+U1=U2
Where K.E.=12mv2 is the kinetic energy at the surface of the larger planet.
Step 7: Solve for the Minimum Speed v
Rearranging gives:
12mv2=U2−U1
Substituting the expressions for U1 and U2 and simplifying will yield an expression for v2.
Final Result
After simplification, we find:
v=32√5GMa
We have two planets:
- Planet 1 (larger): Mass = 16M, Radius = 2a
- Planet 2 (smaller): Mass = M, Radius = a
The gravitational field g due to a planet at a distance r from its center is given by:
g=GMr2
Where G is the gravitational constant.
Let x be the distance from the center of the smaller planet where the gravitational fields due to both planets are equal. The distance from the center of the larger planet to this point is 10a−x.
GMx2=G(16M)(10a−x)2
Cancelling G and M from both sides:
1x2=16(10a−x)2
(10a−x)2=16x2
Expanding and rearranging:
100a2−20ax+x2=16x2
100a2−20ax−15x2=0
15x2+20ax−100a2=0
Here, a=15, b=20a, and c=−100a2:
x=−20a±√(20a)2−4⋅15⋅(−100a2)2⋅15
x=−20a±√400a2+6000a230
x=−20a±√6400a230
x=−20a±80a30
Taking the positive root:
x=60a30=2a
1. At the surface of the larger planet (distance = 8a):
U1=−G(16M)m8a−G(M)m2a
U2=−G(M)m2a−G(16M)m8a
The total mechanical energy at the starting point equals the total mechanical energy at the point where the gravitational fields are equal:
K.E.+U1=U2
Where K.E.=12mv2 is the kinetic energy at the surface of the larger planet.
Rearranging gives:
12mv2=U2−U1
Substituting the expressions for U1 and U2 and simplifying will yield an expression for v2.
After simplification, we find:
v=32√5GMa
Topper's Solved these Questions
Similar Questions
The distance of the two planets from the Sun are 1013m and 1012m, respectively. Find the ratio of time periods of the two planets.
View SolutionTwo planets of masses M and M2 have radii R and R2 respectively. If ratio of escape velocities from their surfaces v1v2 is n4 , then find n :
View SolutionDistance between the centres of two stars is 10a. The masses of these stars are M and 16M and their radii a and 2a respectively. A body of mass m is fired straight from the surface of the larger star towards the surface of the smaller star. What should be its minimum initial speed to reach the surface of the smaller star? Obtain the expression in terms of G, M and a.
View SolutionDistance between the centres of two stars is 10a. The masses of these stars are M and 16M and their radii a and 2a respectively. A body of mass m is fired straight from the surface of the larger star towards the surface of the smaller star. What should be its minimum initial speed to reach the surface of the smaller star? Obtain the expression in terms of G, M and a.
View Solution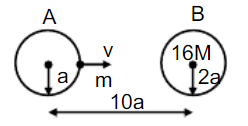
Two planets of mass M and 16M of radius a and 2a respectively, are at distance 10a. Find minimum speed of a particle of mass m at surface of smaller planet so that it can reached from smaller planet to larger planet.
View SolutionThe distance of the two planets from the Sun are 1013m and 1012m, respectively. Find the ratio of time periods of the two planets.
View SolutionIf the angular velocity of a planet about its own axis is halved, the distance of geostationary satellite of this planet from the centre of the centre of the planet will become
View SolutionThe mass and diameter of a planet have twice the value of the corresponding parameters of earth. Acceleration due to gravity on the surface of the planet is
View SolutionThe torque on a planet about the centre of sun is
View SolutionMass and radius of a planet are two times the value of earth. What is the value of escape velocity from the surface of this planet?
View Solution
