A particle of specific charge is projected from the origin of coordinate with initial velocity unifrom electric magnetic fields exist in the region along the +ydirection, of magnitude and The particle will definitely return to the origin once if .
is an integer
is an integer
in an integer
is an integer
The correct Answer is:C
To solve the problem, we need to analyze the motion of a charged particle in the presence of uniform electric and magnetic fields. The particle is projected with an initial velocity and will follow a helical path due to the combined effects of the electric and magnetic fields. We will derive the condition under which the particle returns to the origin.
1. Understanding the Motion:
The particle is projected from the origin with an initial velocity of (ui−vj). The electric field E is in the positive y-direction, and the magnetic field B is also in the positive y-direction. The particle will experience a force due to both fields.
Hint: Identify the direction of the forces acting on the particle due to the electric and magnetic fields.
2. Force on the Particle:
The force F acting on the particle can be expressed using the Lorentz force equation:
F=q(E+v×B)
Since both E and B are in the y-direction, the magnetic force will act perpendicular to the velocity vector.
Hint: Recall that the magnetic force is given by the cross product and will affect the motion in the x and z directions.
3. Helical Motion:
The particle will follow a helical path due to the continuous influence of the magnetic field. The motion in the y-direction will be influenced by the electric field, while the motion in the x and z directions will be circular due to the magnetic field.
Hint: Visualize the helical path and how the particle moves in the y-direction while also revolving around the y-axis.
4. Time Period of Motion:
The time taken for the particle to complete one full revolution in the x−z plane is related to the magnetic field and the charge-to-mass ratio of the particle. The time period T can be expressed as:
T=2πmqB
Hint: Remember that the time period is dependent on the mass of the particle and the magnetic field strength.
5. Condition for Returning to Origin:
For the particle to return to the origin, the time taken to reach the x−z plane must be an integral multiple of the time period T. This can be expressed mathematically as:
t=nT
where n is an integer.
Hint: Relate the distance traveled in the y-direction to the electric field and the time taken.
6. Equating the Time:
The distance traveled in the y-direction under the influence of the electric field E can be expressed as:
dy=vt=qEmt
Setting this equal to the distance traveled in the x−z plane gives:
nT=qEmnT
Hint: Simplify the equation to find a relationship between E, B, and the specific charge q/m.
7. Final Condition:
After simplification, we find that the particle will return to the origin if:
BπE=n
where n is an integer.
Hint: Check the options provided in the question to see which one matches this condition.
Conclusion:
The particle will definitely return to the origin once if the condition BπE is an integer. Therefore, the correct option is C.
The particle is projected from the origin with an initial velocity of (ui−vj). The electric field E is in the positive y-direction, and the magnetic field B is also in the positive y-direction. The particle will experience a force due to both fields.
The force F acting on the particle can be expressed using the Lorentz force equation:
F=q(E+v×B)
Since both E and B are in the y-direction, the magnetic force will act perpendicular to the velocity vector.
The particle will follow a helical path due to the continuous influence of the magnetic field. The motion in the y-direction will be influenced by the electric field, while the motion in the x and z directions will be circular due to the magnetic field.
The time taken for the particle to complete one full revolution in the x−z plane is related to the magnetic field and the charge-to-mass ratio of the particle. The time period T can be expressed as:
T=2πmqB
For the particle to return to the origin, the time taken to reach the x−z plane must be an integral multiple of the time period T. This can be expressed mathematically as:
t=nT
where n is an integer.
The distance traveled in the y-direction under the influence of the electric field E can be expressed as:
dy=vt=qEmt
Setting this equal to the distance traveled in the x−z plane gives:
nT=qEmnT
After simplification, we find that the particle will return to the origin if:
BπE=n
where n is an integer.
The particle will definitely return to the origin once if the condition BπE is an integer. Therefore, the correct option is C.
Topper's Solved these Questions
- MAGNETIC EFFECTS OF CURRENT Book:VMC MODULES ENGLISHChapter:MAGNETIC EFFECTS OF CURRENT Exercise:JEE Main (Archive)
Explore 75 Videos
- MAGNETIC EFFECTS OF CURRENT Book:VMC MODULES ENGLISHChapter:MAGNETIC EFFECTS OF CURRENT Exercise:JEE Advanced (Archive)
Explore 78 Videos
- MAGNETIC EFFECTS OF CURRENT Book:VMC MODULES ENGLISHChapter:MAGNETIC EFFECTS OF CURRENT Exercise:LEVEL 1
Explore 75 Videos
- LIQUIDSBook:VMC MODULES ENGLISHChapter:LIQUIDSExercise:JEE ADVANCED (LEVEL -2)
Explore 55 Videos
- MOCK TEST 1Book:VMC MODULES ENGLISHChapter:MOCK TEST 1Exercise:PART I : PHYSICS (SECTION-2)
Explore 10 Videos
Similar Questions
A particle of specific charge α is projected from origin with velocity v=v0ˆi−v0ˆk in a uniform magnetic field B=−B0ˆk. Find time dependence of velocity and position of the particle.
View SolutionA particle of specific charge q/m=(π)C/kg is projected from the origin towards positive x-axis with a velocity of 10m/s in a uniform magnetic field →B=−2ˆK Tesla. The velocity →V of the particle after time t=1/6 s will be
View SolutionA particle of specific charge q/m=(π)C/kg is projected from the origin towards positive x-axis with a velocity of 10m/s in a uniform magnetic field →B=−2ˆK Tesla. The velocity →V of the particle after time t=1/6 s will be
View SolutionA particle of mass m and charge −q is projected from the origin with a horizontal speed v into an electric field of intensity E directed downward. Choose the wrong statement. Neglect gravity
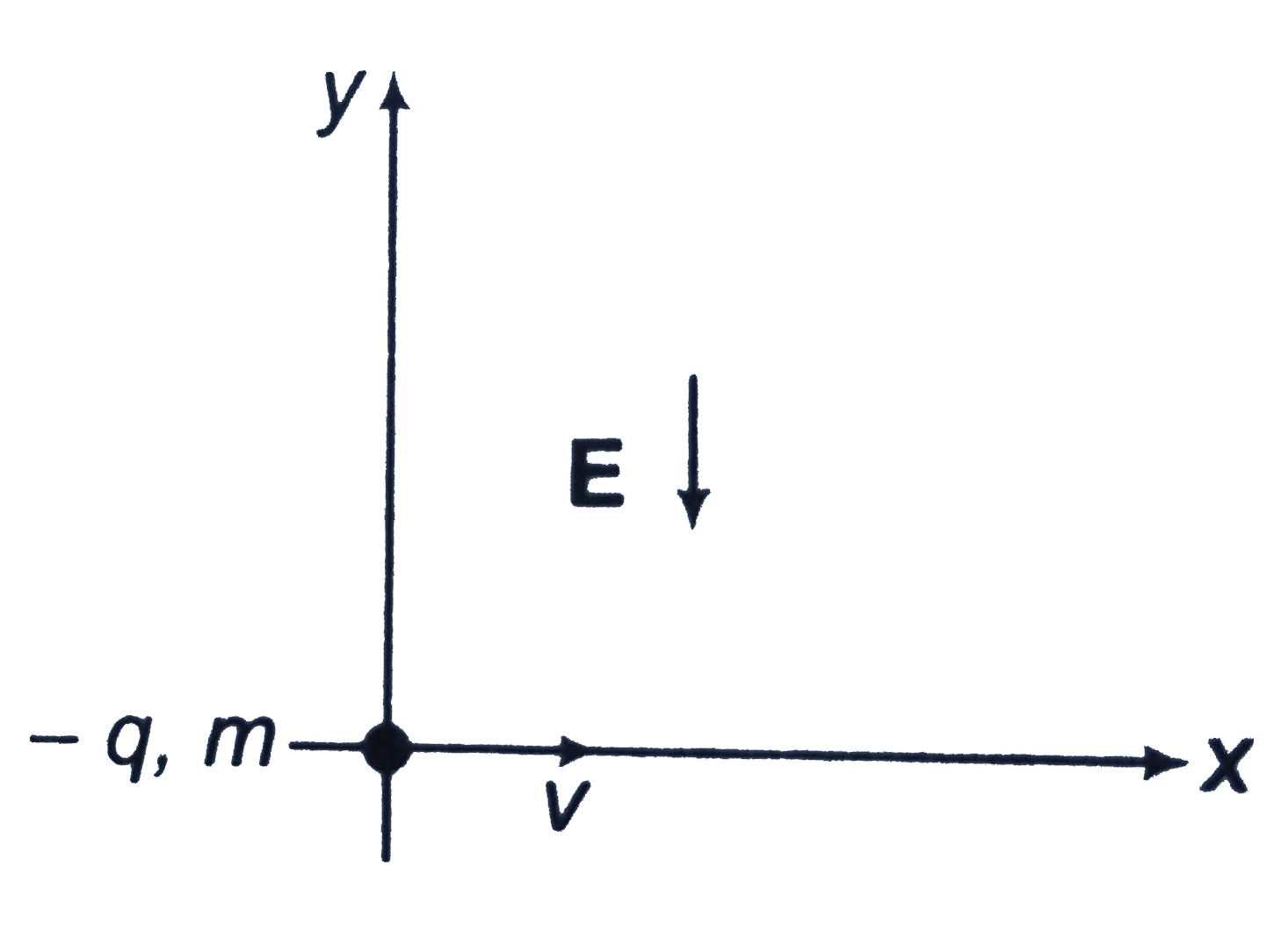
View SolutionA particle of mass m and charge −q is projected from the origin with a horizontal speed v into an electric field of intensity E directed downward. Choose the wrong statement. Neglect gravity

View SolutionA charged particle of specific charge s moves undeflected through a region of space containing mutually perpendicular and uniform electric and magnetic fields, E and B. When the field E is switched off, the particle will move in a circular path of radius
View SolutionA charged particle of specific charge s moves undeflected through a region of space containing mutually perpendicular and uniform electric and magnetic fields, E and B. When the field E is switched off, the particle will move in a circular path of radius
View SolutionA particle of charge q0 and of mass m0 is projected along the y-axis at t=0 from origin with a velocity V0. If a uniform electric field E0 also exist along the x-axis, then the time at which debroglie wavelength of the particle becomes half of the initial value is:
View SolutionA particle of mass m and positive charge q is projected with a speed of v0 in y–direction in the presence of electric and magnetic field are in x–direction. Find the instant of time at which the speed of particle becomes double the initial speed.
View SolutionA particle is released from the origin with a velocity vˆe(i). The electric field in the region is Eˆe(i) and magnetic field is Bˆk. Then
View Solution