A particle whose speed is 50ms−1 moves along the line from A(2,1) to B(9,25). Find its velocity vector in the from of aˆi+bˆj.
(7ˆi+24ˆj)m/s
2(7ˆi+24ˆj)m/s
4(7ˆi+24ˆj)m/s
5(7ˆi+24ˆj)m/s
The correct Answer is:B
To find the velocity vector of a particle moving from point A(2,1) to point B(9,25) with a speed of 50 m/s, we can follow these steps:
Step 1: Determine the position vectors of points A and B
The position vector of point A can be represented as:
→OA=2^i+1^j
The position vector of point B can be represented as:
→OB=9^i+25^j
Step 2: Find the displacement vector from A to B
The displacement vector →AB can be calculated as:
→AB=→OB−→OA=(9^i+25^j)−(2^i+1^j)=(9−2)^i+(25−1)^j=7^i+24^j
Step 3: Calculate the magnitude of the displacement vector
The magnitude of the displacement vector →AB is given by:
|→AB|=√(72)+(242)=√49+576=√625=25
Step 4: Determine the unit vector in the direction of AB
The unit vector ^AB in the direction of →AB is calculated as:
^AB=→AB|→AB|=7^i+24^j25=725^i+2425^j
Step 5: Calculate the velocity vector
The velocity vector →v can be expressed as:
→v=speed×^AB=50×(725^i+2425^j)
Calculating this gives:
→v=50×725^i+50×2425^j=2×7^i+2×24^j=14^i+48^j
Final Result
Thus, the velocity vector in the form of a^i+b^j is:
→v=14^i+48^j
---
The position vector of point A can be represented as:
→OA=2^i+1^j
The position vector of point B can be represented as:
→OB=9^i+25^j
The displacement vector →AB can be calculated as:
→AB=→OB−→OA=(9^i+25^j)−(2^i+1^j)=(9−2)^i+(25−1)^j=7^i+24^j
The magnitude of the displacement vector →AB is given by:
|→AB|=√(72)+(242)=√49+576=√625=25
The unit vector ^AB in the direction of →AB is calculated as:
^AB=→AB|→AB|=7^i+24^j25=725^i+2425^j
The velocity vector →v can be expressed as:
→v=speed×^AB=50×(725^i+2425^j)
Calculating this gives:
→v=50×725^i+50×2425^j=2×7^i+2×24^j=14^i+48^j
Thus, the velocity vector in the form of a^i+b^j is:
→v=14^i+48^j
Topper's Solved these Questions
- BASIC MATHEMATICS & VECTORSBOOK - VMC MODULES ENGLISHCHAPTER - BASIC MATHEMATICS & VECTORSEXERCISE - Impeccable
50 Videos
- BASIC MATHEMATICS & VECTORSBOOK - VMC MODULES ENGLISHCHAPTER - BASIC MATHEMATICS & VECTORSEXERCISE - Enable
50 Videos
- CAPACITORSBOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - JEE Advance ( Archive ) LEVEL 48
1 Videos
Similar Questions
A particle travels with speed 50ms−1 from the point (3,−7) in a direction 7ˆi−24(j). Find its position vector after 3s.
View SolutionA particle is moving with speed 6m/s along the direction of →A=2ˆi+2ˆj−ˆk, then its velocity is :
View SolutionWhen a body moved along a straight line from A to B its velocity vector is represented by a straight line - directed from A to B . But " when the same body rotates in circular path is velocity vector is represented by a straight line perpendicular to the plane of the circular path " . Why ?
View SolutionA particle of mass m is moving along the line y-b with constant acceleration a. The areal velocity of the position vector of the particle at time t is (u=0)
View SolutionA particle moves in a circle of radius 4 m with a linear velocity of 20ms−1. Find the angular velocity.
View SolutionA particle is projected with a velocity ¯v=aˆi+bˆj . Find the radius of curvature of the trajectory of the particle at (i) point of projection (ii) highest point .
View SolutionIf a particle moves from the point A(1,2,3) to the point B(4,6,9), its displacement vector be
View SolutionA particle moves along the curve x29+y24=1, with constant speed v. Express its "velocity vectorially" as a function of x,y.
View SolutionA particle starts motion from rest and moves along a straight line. Its velocity-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View SolutionSystem is shown in fig. and wedge is moving toward left with speed 2ms−1. Find the velocity of the block B.
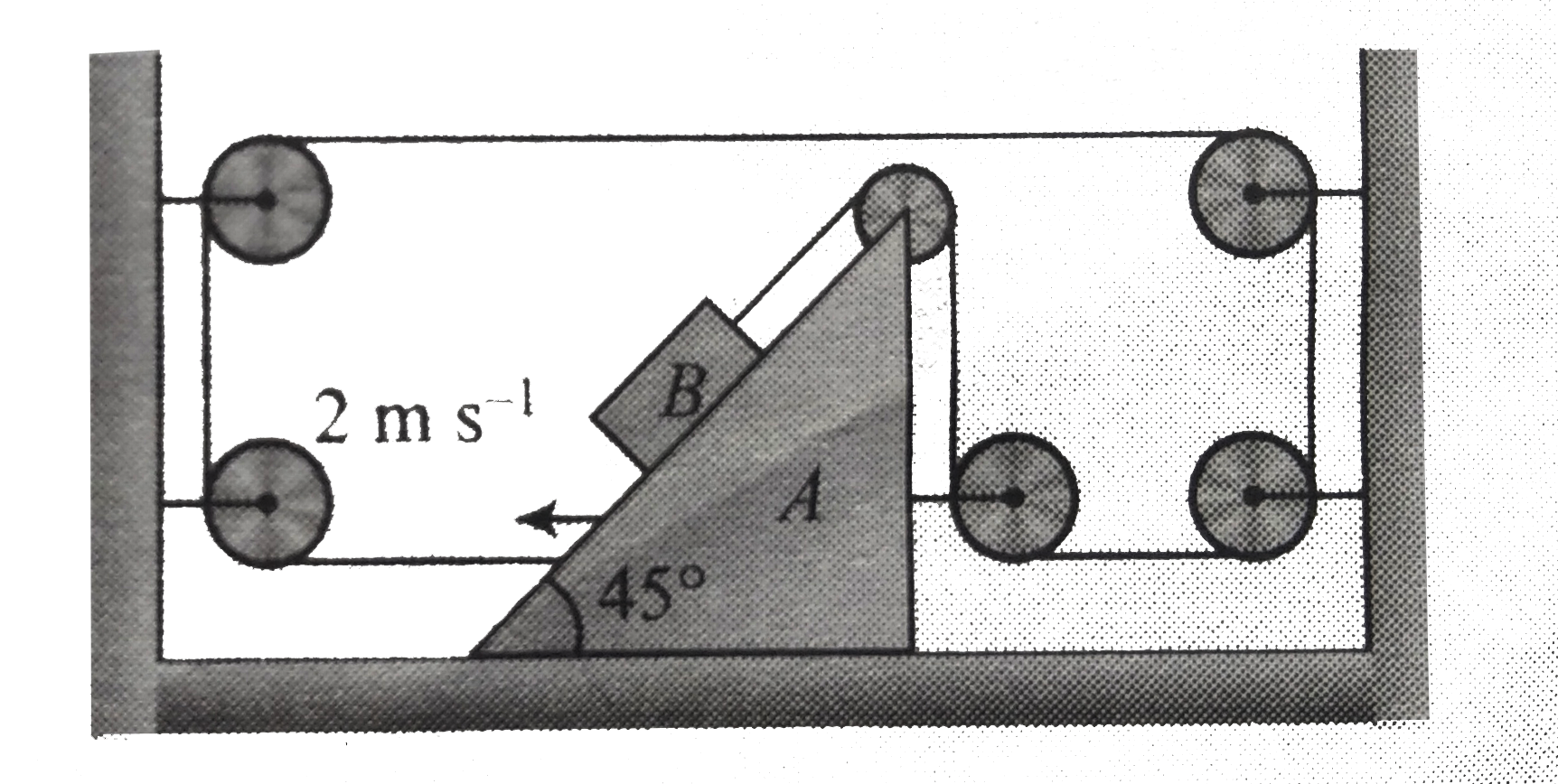
View Solution
