A disc of radius r is removed from the centre of a disc of mass M and radius R. The M.I. About the axis through the centre and normal to the plane will be:
M(R2−r2)/2
M(R2+r2)/2
M(R4−r4)/2R2
M(R2)/2
The correct Answer is:B
To find the moment of inertia (M.I.) of a disc with a smaller disc removed from its center, we can follow these steps:
Step 1: Understand the Moment of Inertia of a Disc
The moment of inertia I of a solid disc about an axis through its center and perpendicular to its plane is given by the formula:
I=12MR2
where M is the mass of the disc and R is its radius.
Step 2: Determine the Mass of the Larger Disc
Let the larger disc have a mass M and radius R. The mass per unit area σ of the larger disc can be expressed as:
σ=MπR2
Step 3: Calculate the Moment of Inertia of the Larger Disc
Using the mass per unit area, the moment of inertia I1 of the larger disc can be calculated as:
I1=12MR2
Step 4: Determine the Mass of the Smaller Disc
Let the smaller disc that is removed have a radius r. The mass of the smaller disc m can be expressed as:
m=σ⋅πr2=(MπR2)⋅πr2=Mr2R2
Step 5: Calculate the Moment of Inertia of the Smaller Disc
The moment of inertia I2 of the smaller disc about the same axis is:
I2=12mr2=12(Mr2R2)r2=12Mr4R2
Step 6: Calculate the Net Moment of Inertia
The net moment of inertia I of the system after removing the smaller disc from the larger disc is:
I=I1−I2=12MR2−12Mr4R2
Factoring out 12M:
I=12M(R2−r4R2)
Step 7: Simplify the Expression
To simplify the expression, we can write it as:
I=12M(R4−r4R2)
Thus, the final expression for the moment of inertia of the system is:
I=M2(R2−r4R2)
Final Answer
The moment of inertia about the axis through the center and normal to the plane is:
I=M2(R2−r4R2)
---
The moment of inertia I of a solid disc about an axis through its center and perpendicular to its plane is given by the formula:
I=12MR2
where M is the mass of the disc and R is its radius.
Let the larger disc have a mass M and radius R. The mass per unit area σ of the larger disc can be expressed as:
σ=MπR2
Using the mass per unit area, the moment of inertia I1 of the larger disc can be calculated as:
I1=12MR2
Let the smaller disc that is removed have a radius r. The mass of the smaller disc m can be expressed as:
m=σ⋅πr2=(MπR2)⋅πr2=Mr2R2
The moment of inertia I2 of the smaller disc about the same axis is:
I2=12mr2=12(Mr2R2)r2=12Mr4R2
The net moment of inertia I of the system after removing the smaller disc from the larger disc is:
I=I1−I2=12MR2−12Mr4R2
Factoring out 12M:
I=12M(R2−r4R2)
To simplify the expression, we can write it as:
I=12M(R4−r4R2)
Thus, the final expression for the moment of inertia of the system is:
I=M2(R2−r4R2)
The moment of inertia about the axis through the center and normal to the plane is:
I=M2(R2−r4R2)
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - ENABLE
50 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EFFICIENT
50 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - IN - CHAPTER EXERCISE - B
10 Videos
Similar Questions
Four similar point masses (each of mass m) are placed on the circumference of a disc of mass M and radius R. The M.I. of the system about the normal axis through the centre O will be:
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionThe M.I. of a thin ring of mass M and radius R about an axis through the diameter in its plane will be:
View SolutionThe moment of inertia of a disc of mass M and radius R about a tangent to its rim in its plane is
View SolutionThe ratio of radii of gyration of a circular ring and a circular disc, of the same mass and radius about an axis passing through their centres and perpendicular to their planes are
View SolutionMoment of inertia of a uniform quarter disc of radius R and mass M about an axis through its centre of mass and perpendicular to its plane is :
View SolutionMoment of inertia of a uniform quarter disc of radius R and mass M about an axis through its centre of mass and perpendicular to its plane is :
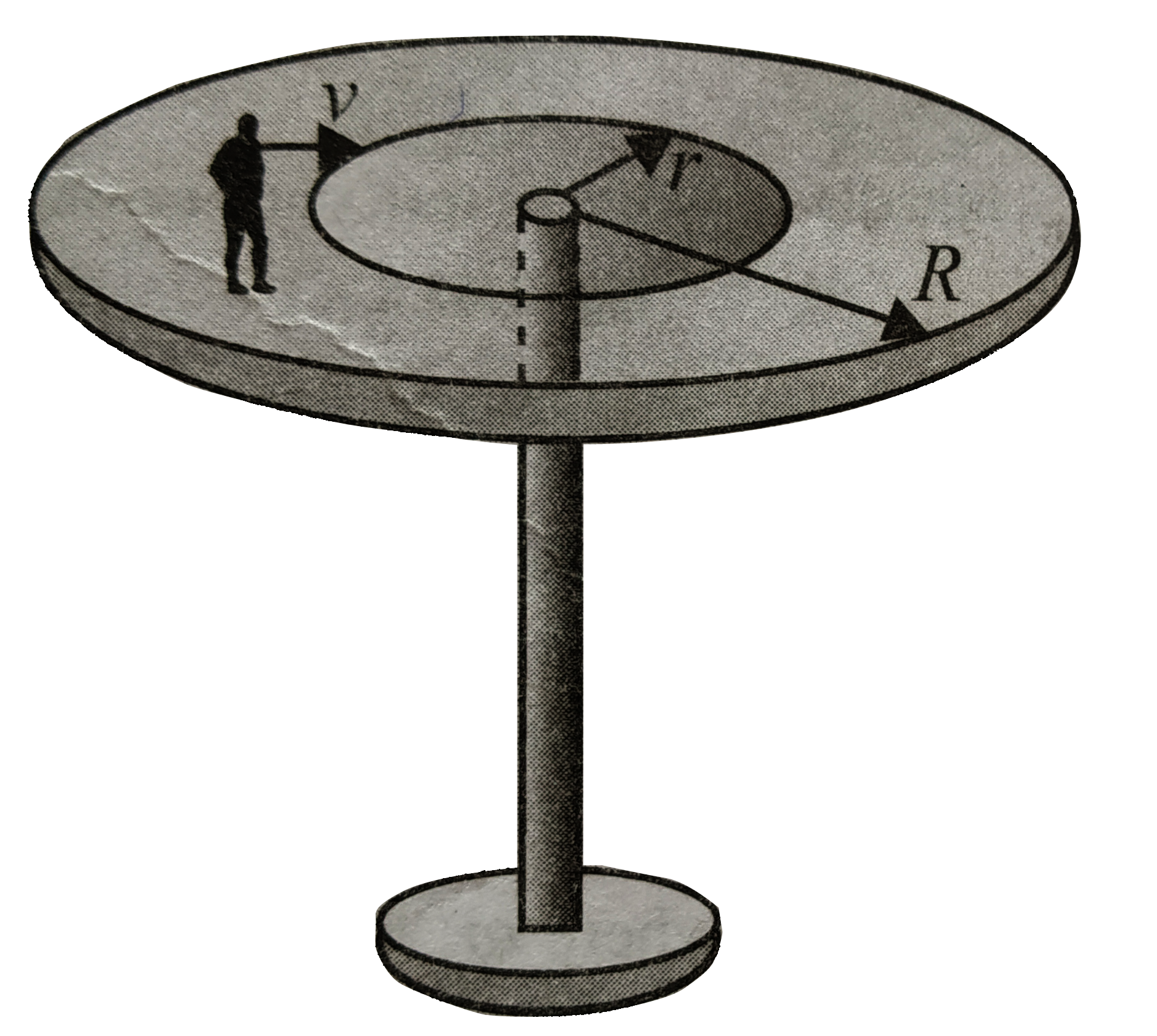
View SolutionA man of mass m stands on a horizontal platform in the shape of a disc of mass m and radius R, pivoted on a vertical axis thorugh its centre about which it can freely rotate. The man starts to move aroung the centre of the disc in a circle of radius r with a velocity v relative to the disc. Calculate the angular velocity of the disc.
View SolutionA disc of radius R has a concentric hole of radius R /2 and its mass is M. Its moment of inertia about an axis through its centre and perpendicular to its plane, is
View SolutionFrom a circular disc of radius R and mass 9 M , a small disc of radius R/3 is removed from the disc. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through O is

View Solution
