A rod standing vertically on a table falls down. What will be the linear velocity of the middle point of the rod at the end of the fall, if the rod is 15 cm long? Assume sufficient friction for no slipping?
1.05m/sec
2.1m/sec
3m/sec
0.1m/sec
The correct Answer is:1
To find the linear velocity of the middle point of a rod that falls from a vertical position to a horizontal position, we can follow these steps:
Step 1: Understand the Problem
The rod is 15 cm long and falls from a vertical position. We need to find the linear velocity of the middle point of the rod just before it hits the ground.
Step 2: Identify Key Points
The center of mass of the rod is located at its midpoint. When the rod falls, it rotates about the point of contact with the table. The height of the center of mass when the rod is vertical is L2, where L is the length of the rod.
Step 3: Calculate the Height of the Center of Mass
Given that the length of the rod L=15 cm, the height of the center of mass from the ground when the rod is vertical is:
h=L2=15 cm2=7.5 cm=0.075 m
Step 4: Apply the Work-Energy Theorem
The work done by gravity when the center of mass falls a height h is equal to the change in kinetic energy:
W=ΔKE
The work done by gravity is:
W=mgh
The change in kinetic energy for the falling rod (initially at rest) is:
ΔKE=12Iω2
where I is the moment of inertia of the rod about the point of contact and ω is the angular velocity.
Step 5: Find the Moment of Inertia
The moment of inertia I of a rod about an axis through one end is given by:
I=13mL2
Step 6: Relate Angular Velocity to Linear Velocity
The linear velocity v of the center of mass is related to the angular velocity ω by:
v=ωL2
Step 7: Combine the Equations
Using the work-energy theorem:
mgh=12Iω2
Substituting I:
mgh=12(13mL2)ω2
Cancelling m from both sides (assuming m≠0):
gh=16L2ω2
Solving for ω:
ω2=6ghL2
Step 8: Substitute for v
Now substituting ω into the equation for v:
v=ωL2=L2√6ghL2=L2⋅√6ghL=√6gh2
Step 9: Substitute Values
Substituting g=9.81m/s2 and h=0.075m:
v=√6⋅9.81⋅0.0752
Calculating this:
v=√4.4132≈2.12≈1.05m/s
Final Answer
The linear velocity of the middle point of the rod at the end of the fall is approximately 1.05m/s.
---
The rod is 15 cm long and falls from a vertical position. We need to find the linear velocity of the middle point of the rod just before it hits the ground.
The center of mass of the rod is located at its midpoint. When the rod falls, it rotates about the point of contact with the table. The height of the center of mass when the rod is vertical is L2, where L is the length of the rod.
Given that the length of the rod L=15 cm, the height of the center of mass from the ground when the rod is vertical is:
h=L2=15 cm2=7.5 cm=0.075 m
The work done by gravity when the center of mass falls a height h is equal to the change in kinetic energy:
W=ΔKE
The work done by gravity is:
W=mgh
The change in kinetic energy for the falling rod (initially at rest) is:
ΔKE=12Iω2
where I is the moment of inertia of the rod about the point of contact and ω is the angular velocity.
The moment of inertia I of a rod about an axis through one end is given by:
I=13mL2
The linear velocity v of the center of mass is related to the angular velocity ω by:
v=ωL2
Using the work-energy theorem:
mgh=12Iω2
Substituting I:
mgh=12(13mL2)ω2
Cancelling m from both sides (assuming m≠0):
gh=16L2ω2
Solving for ω:
ω2=6ghL2
Now substituting ω into the equation for v:
v=ωL2=L2√6ghL2=L2⋅√6ghL=√6gh2
Substituting g=9.81m/s2 and h=0.075m:
v=√6⋅9.81⋅0.0752
v=√4.4132≈2.12≈1.05m/s
The linear velocity of the middle point of the rod at the end of the fall is approximately 1.05m/s.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE-14videos
SYSTEM OF A PARTICLES & ROTATIONAL MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE-25videos
SYSTEM OF A PARTICLES & ROTATIONAL MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F10videos
SYSTEM OF A PARTICLES & ROTATIONAL MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question46videos
SIMPLE HARMONIC MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - IMPECCABLE56videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
Similar Questions
A thin rod of length L and mass M is held vertically with one end on the floor and is allowed to fall. Find the velocity of the other end when it hits the floor, assuming that the end on the floor does not slip?
View SolutionA uniform rod kept vertically on the ground falls from rest. Its foot does not slip on the ground
View SolutionA uniform rod of length l is slightly disturbed from its vertical position. Find the angular velocity of the rod just after if hits the step. (Friction is sufficient everywhere to prevent slipping)
 View Solution
View SolutionA uniform rod of maass m and length L is placed on the fixed cylindrical surface of radius R at an small angular position θ from the vertical (vertical means line joining centre and vertex of the cylindrical path) as shown in the figure and released from rest. Find the angular velocity ω of the rod at the instant when it crosses the horizontal position (Assume that when rod comes at horizontal position its mid point and vertex of the circular surface coincide). Friction is sufficient to prevent any slipping.
 View Solution
View SolutionA uniform rod of length l and mass 2m rests on a smooth horizontal table. A point mass m moving horizontally at right angles to the rod with initial velocity v collides with one end of the rod and sticks to it.
Determine the position of the point on the rod which remains stationary immediately after collision.View SolutionA vertical rod is hit at one end. What kind of wave propagates in the rod if (a) the hit is made vertically (b) the hit is made horizontally ?
View SolutionA very long uniformly charged rod falls with a constant velocity V through the centre of a circular loop. Then the magnitude of induced emf in the loop is
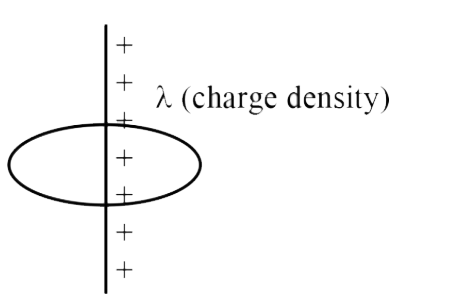 View Solution
View SolutionA bead of mass m is fitted on a rod and can move on it without friction. Initially the bead is at the middle of the rod moves transitionally in the vertical plane with an accleration a0 in direction forming angle α with the rod as shown. The acceleration of bead with respect to rod is:
zView SolutionA uniform thin rod of mass m and length L is standing vertically along the y-axis on a smooth horizontal surface, with its lower end at the origin (0,0). A slight disturbane at t = 0 causes the lower end t slip on the smooth surface along the positive x-axis, and the rod starts falling.
(i) What is the path followed by the centr of mass of the rod during its fall?
(ii) Find the equaiton to the trajectory of a point on the rod shape of the path of this point?View SolutionA uniform steel rod of cross- sectional area A and L is suspended so that it hangs vertically. The stress at the middle point of the rod is
View Solution

