A wheel of radius r rolls without slipping with a speed v on a horizontal road. When it is at a point A on the road, a small blob of mud separates from'the wheel at- its highest point and lands at point B on the road:
(a)AB=v√rg
(b)AB=2v√rg
(c)AB=4v√rg
(d)v>√4rg,the blob of mud will land on the wheel and not on the road
The correct Answer is:2
To solve the problem step by step, we will analyze the motion of the blob of mud that separates from the wheel and lands on the road.
Step 1: Determine the initial conditions of the blob of mud
When the blob of mud separates from the wheel at its highest point, it has two key characteristics:
- Initial velocity (u): Since the wheel is rolling without slipping, the velocity of the blob at the highest point is equal to the linear velocity of the wheel, which is v. However, since it is at the highest point, it also has a vertical component due to the rolling motion. The total velocity of the blob when it separates is 2v (the horizontal component is v and the vertical component is also v).
- Initial height (h): The height from which the blob falls is equal to the diameter of the wheel, which is 2r.
Step 2: Calculate the time of flight (t)
The motion of the blob can be analyzed using the equations of motion under gravity. The vertical motion can be described by the equation:
\(
h = ut + \frac{1}{2}gt^2
\)
where:
- \( h = 2r \) (the height),
- \( u = 0 \) (the initial vertical velocity),
- \( g \) is the acceleration due to gravity.
Substituting the values, we have:
\(
2r = 0 + \frac{1}{2}gt^2
\)
This simplifies to:
\(
2r = \frac{1}{2}gt^2
\)
Multiplying both sides by 2 gives:
\(
4r = gt^2
\)
Rearranging this gives:
\(
t^2 = \frac{4r}{g}
\)
Taking the square root of both sides, we find:
\(
t = 2\sqrt{\frac{r}{g}}
\)
Step 3: Calculate the horizontal distance traveled (s)
The horizontal distance traveled by the blob while it is falling can be calculated using the formula:
\(
s = vt
\)
where:
- \( v = 2v \) (the horizontal velocity of the blob),
- \( t = 2\sqrt{\frac{r}{g}} \) (the time of flight).
Substituting these values into the equation gives:
\(
s = 2v \cdot 2\sqrt{\frac{r}{g}}
\)
This simplifies to:
\(
s = 4v\sqrt{\frac{r}{g}}
\)
Step 4: Conclusion
The horizontal distance from point A to point B where the blob of mud lands is:
\(
AB = 4v\sqrt{\frac{r}{g}}
\)
Final Answer
The distance AB is \( 4v\sqrt{\frac{r}{g}} \).
When the blob of mud separates from the wheel at its highest point, it has two key characteristics:
- Initial velocity (u): Since the wheel is rolling without slipping, the velocity of the blob at the highest point is equal to the linear velocity of the wheel, which is v. However, since it is at the highest point, it also has a vertical component due to the rolling motion. The total velocity of the blob when it separates is 2v (the horizontal component is v and the vertical component is also v).
- Initial height (h): The height from which the blob falls is equal to the diameter of the wheel, which is 2r.
The motion of the blob can be analyzed using the equations of motion under gravity. The vertical motion can be described by the equation:
\(
h = ut + \frac{1}{2}gt^2
\)
where:
- \( h = 2r \) (the height),
- \( u = 0 \) (the initial vertical velocity),
- \( g \) is the acceleration due to gravity.
\(
2r = 0 + \frac{1}{2}gt^2
\)
This simplifies to:
\(
2r = \frac{1}{2}gt^2
\)
Multiplying both sides by 2 gives:
\(
4r = gt^2
\)
Rearranging this gives:
\(
t^2 = \frac{4r}{g}
\)
Taking the square root of both sides, we find:
\(
t = 2\sqrt{\frac{r}{g}}
\)
The horizontal distance traveled by the blob while it is falling can be calculated using the formula:
\(
s = vt
\)
where:
- \( v = 2v \) (the horizontal velocity of the blob),
- \( t = 2\sqrt{\frac{r}{g}} \) (the time of flight).
\(
s = 2v \cdot 2\sqrt{\frac{r}{g}}
\)
This simplifies to:
\(
s = 4v\sqrt{\frac{r}{g}}
\)
The horizontal distance from point A to point B where the blob of mud lands is:
\(
AB = 4v\sqrt{\frac{r}{g}}
\)
The distance AB is \( 4v\sqrt{\frac{r}{g}} \).
Topper's Solved these Questions
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE-1
4 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE-2
5 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question
46 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - IMPECCABLE
56 Videos
Similar Questions
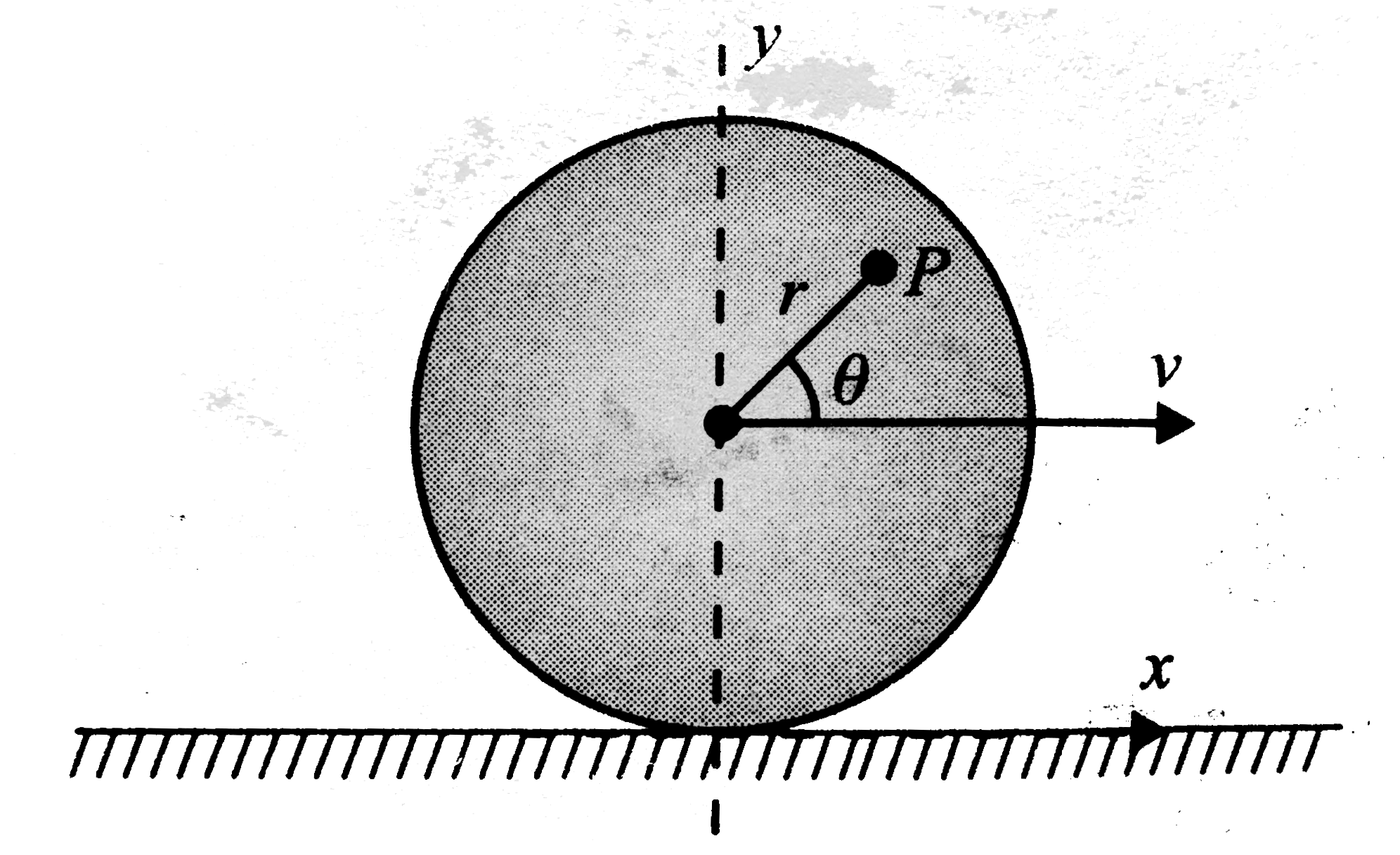
A disc of radius R rolls without slipping at speed v along positive x-axis. Velocity of point P at the instant shown in Fig. is
View Solution
A ring of radius R rolls without slipping on a rough horizontal surface with a constant velocity. The radius of curvature of the path followed by any particle of the ring at the highest point of its path will be :
View SolutionA circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0 . We consider a point A on the surface of the disc. Then, the acceleration of point A is
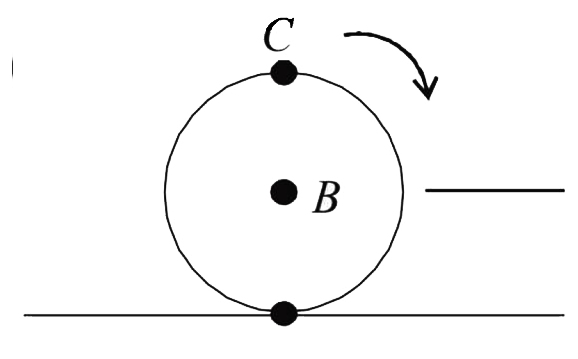
View SolutionA sphere is rolling without slipping on a fixed horizontal plane surface. In the figure, A is the point of contact, B is the centre of the sphere and C is its topmost point. Then
View SolutionThe wheel of radius R rolls wihtout slipping on horizontal rough surface and its centre O has an acceleration a0 in forward direction. A point P on the wheel is a distance r from O and angular position θ from horizontal. Find the angle θ for which point P can have zero acceleration in this position .

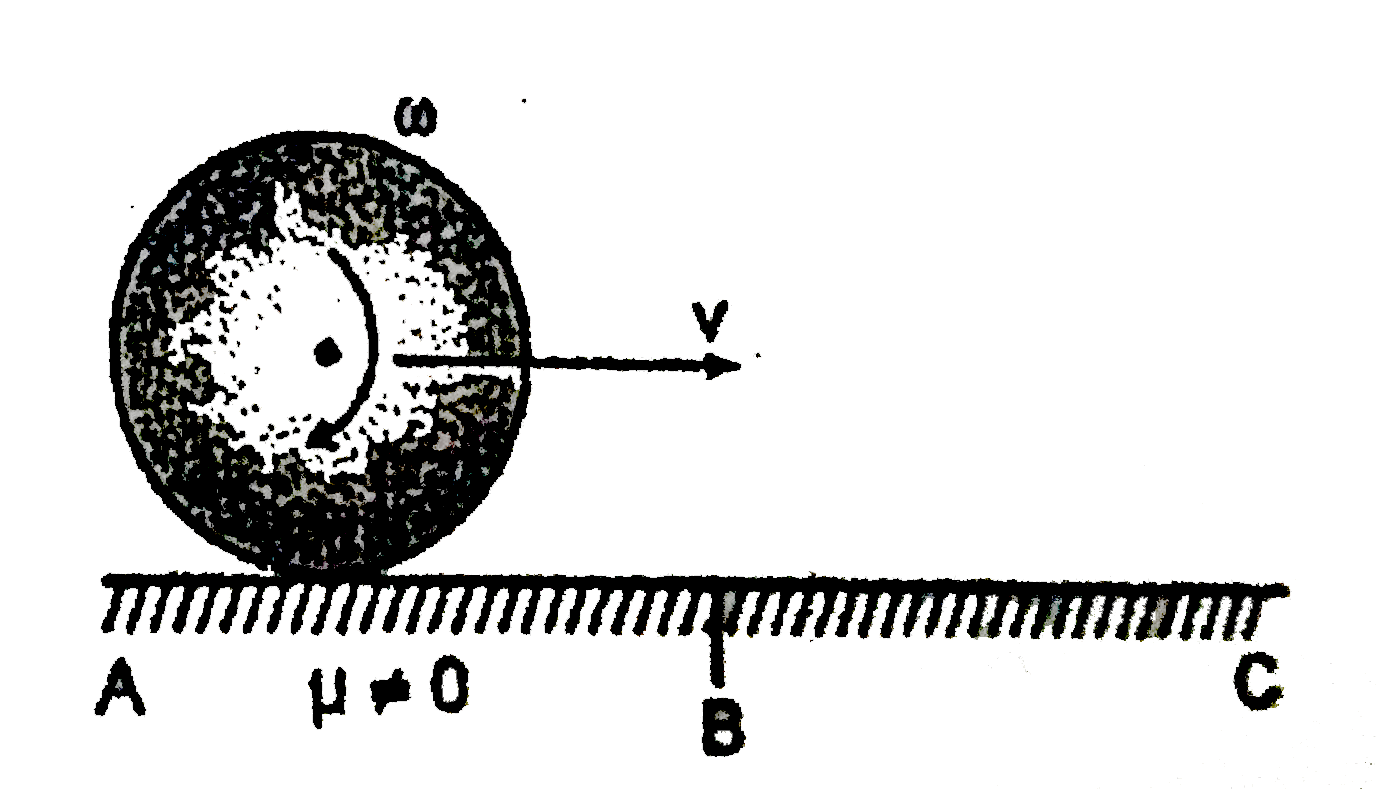
View SolutionAs shown in the figure, a disc of mass m is rolling without slipping with angular velocity ω. When it crosses point B disc will be in
View SolutionA wheel of bicycle is rolling without slipping on a level road. The velocity of the centre of mass is vCM, then true statement is

View SolutionA wheel of bicycle is rolling without slipping on a level road. The velocity of the centre of mass is vCM, then true statement is

View SolutionA wheel of radius R rolls on the ground with a uniform velocity v. The velocity of topmost point relative to the bottommost point is
View Solution
A wheel is rolling without slipping on a horizontal plane with velocity v and acceleration a of centre of mass as shown in figure. Acceleration at
View Solution
