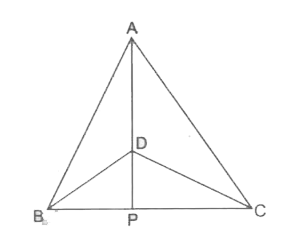
and are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (See Fig.
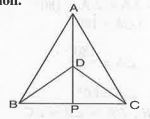 ).If AD is extended to intersect BC at P, show that AP is the perpendicular bisector of BC.Solution in Punjabi
).If AD is extended to intersect BC at P, show that AP is the perpendicular bisector of BC.Solution in Punjabi
Step by step video & image solution for DeltaABC and DeltaDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (See Fig. ).If AD is extended to intersect BC at P, show that AP is the perpendicular bisector of BC. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
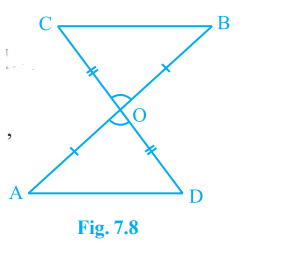
In Fig. 7.8, OA = OB and OD = OC. Show that (i) ΔAOD≅ΔBOC and (ii) AD || BC.
View SolutionMary wants to decorate her Christmas tree. She wants to place the tree on a wooden box covered with coloured paper with picture of Santa Claus on it (see Fig. 13.4). She must know the exact quantity of paper to buy for this purpose. If the box has length, breadth and height as 80 cm, 40 cm and 20 cm respectively how many square sheets of paper of side 40 cm would she require?
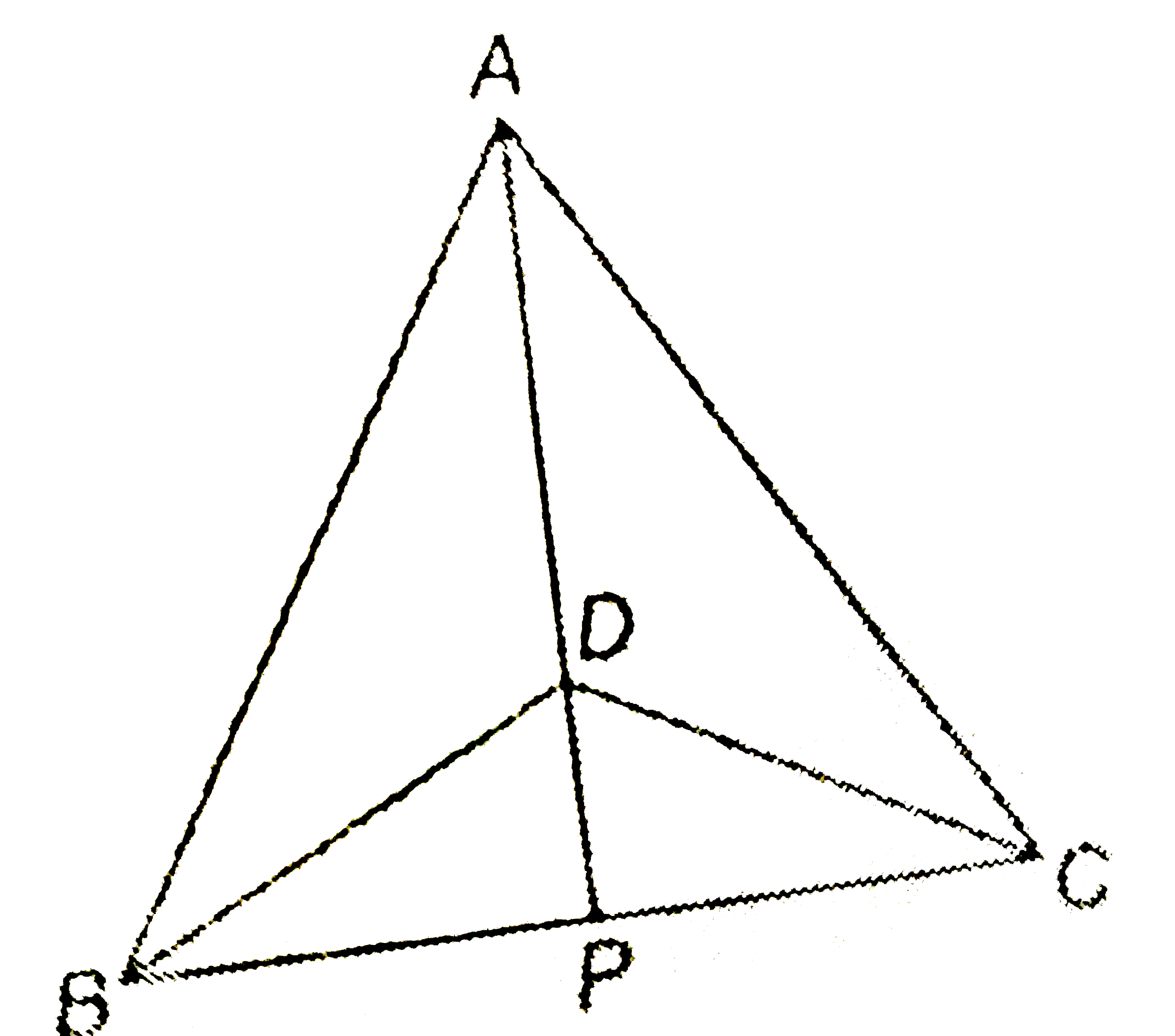
View SolutionΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see figure). If AD is extended to intersect BC at P, show that :
(i) ΔABD≅ΔACD
(ii) ΔABP≅ΔACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC.
View SolutionΔABC और ΔDBC एक ही आधार BC पर बने दो समद्विबाहु त्रिभुज इस प्रकार हैं कि A और D भुजा BC के एक ही ओर स्थित हैं (देखिए चित्र) | यदि AD बढ़ाने पर BC को P पर प्रतिच्छेद करें, तो दर्शाइए कि
AP रेखाखंड BC का लम्ब समद्विभाजक है |
View Solutionत्रिभुज ABC और ΔDBCएक ही आधार BC पर बने दो समद्विबाहु त्रिभुज इस प्रकर हैं कि शीर्ष A और B भुजा BC के एक ही ओर स्थित हैं | यदि AD को बढ़ाने पर वह BC को P पर प्रतिच्छेदित सिध्द करो AP,angleA को समद्विभाजित करता है |
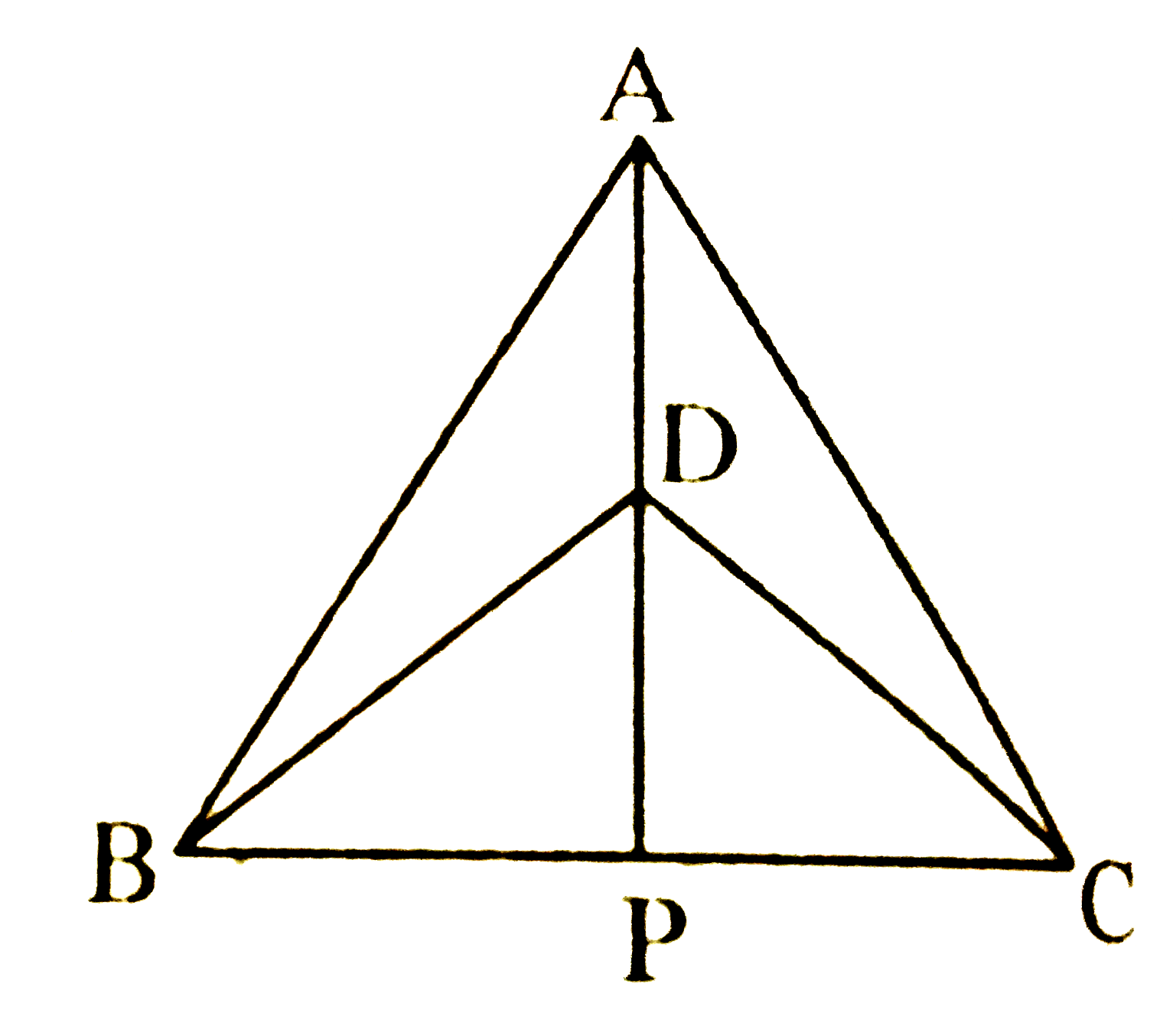
View Solution△ABC and △DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that:
(i) △ABD≅△ACD
(ii) △ABP≅△ACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC.
View SolutionIn the given fig. If DE||BC Find EC.
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/MATH_241_X_SQP_E01_021_Q01.png" width="80%"gt
View SolutionΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (See Fig.
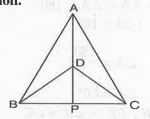 ).If AD is extended to intersect BC at P, show that ΔABD≅ΔACD.
).If AD is extended to intersect BC at P, show that ΔABD≅ΔACD.
View SolutionΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (See Fig.
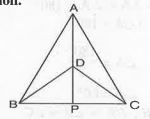 ).If AD is extended to intersect BC at P, show that ΔABP≅ΔACP.
).If AD is extended to intersect BC at P, show that ΔABP≅ΔACP.
View SolutionΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (See Fig.
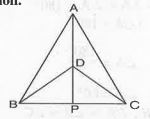 ).If AD is extended to intersect BC at P, show that AP bisects ∠A as well as ∠D.
).If AD is extended to intersect BC at P, show that AP bisects ∠A as well as ∠D.
View SolutionΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (See Fig.
 ).If AD is extended to intersect BC at P, show that AP is the perpendicular bisector of BC.
).If AD is extended to intersect BC at P, show that AP is the perpendicular bisector of BC.
View Solution△ABC ਅਤੇ △DBC ਇੱਕ ਹੀ ਆਧਾਰ BC ਉਤੇ ਦੋ ਸਮਦੋਭੁਜੀ ਤ੍ਰਿਭੁਜਾਂ ਹਨ ਅਤੇ ਸਿਖਰ A ਅਤੇ D ਭੁਜਾ BC ਦੇ ਇਕੋ ਪਾਸੇ ਹਨ (ਵੇਖੋ ਚਿੱਤਰ 7.39)। ਜੇ ਭੁਜਾ AD ਨੂੰ ਇਸ ਤਰ੍ਹਾਂ ਵਧਾਇਆ ਜਾਵੇ ਕਿ ਭੁਜਾ BC ਨੂੰ P 'ਤੇ ਕੱਟੇ ਤਾਂ ਸਿੱਧ ਕਰੋ ਕਿ AP, ਭੁਜਾ BC ਦਾ ਲੰਬ ਸਮਦੁਭਾਜਕ ਹੈ।
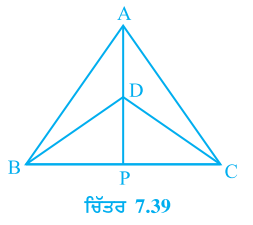
View SolutionTriangles ABC and DBC are two isosceles triangles on the same base BC and their vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that:
ΔABP≅ΔACP
View SolutionTriangles ABC and DBC are two isosceles triangles on the same base BC and their vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that:
AP bisects angle BAC and BDC.
View SolutionΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (See Fig.
 ).If AD is extended to intersect BC at P, show that ΔABD≅ΔACD.
).If AD is extended to intersect BC at P, show that ΔABD≅ΔACD.
View SolutionΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (See Fig.
 ).If AD is extended to intersect BC at P, show that ΔABP≅ΔACP.
).If AD is extended to intersect BC at P, show that ΔABP≅ΔACP.
View Solution
