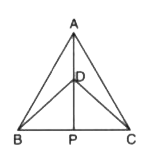
Triangles ABC and DBC are two isosceles triangles on the same base BC and their vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that:
ΔABP≅ΔACP
To show that triangles ABP and ACP are congruent, we will use the properties of isosceles triangles and the congruence criteria.
1. Identify the Isosceles Triangles:
- We have two isosceles triangles: triangle ABC and triangle DBC.
- In triangle ABC, since it is isosceles, we have AB=AC.
- In triangle DBC, since it is also isosceles, we have BD=DC.
2. Extend Line AD to Intersect BC at Point P:
- Extend line AD such that it intersects line BC at point P.
3. Common Side:
- Both triangles ABP and ACP share the common side AP.
4. Establishing Congruence of Triangles ABD and ACD:
- In triangle ABD and triangle ACD:
- AB=AC (since triangle ABC is isosceles)
- AD=AD (common side)
- BD=DC (since triangle DBC is isosceles)
- By the SSS (Side-Side-Side) congruence criterion, triangle ABD is congruent to triangle ACD:
△ABD≅△ACD
5. Angles from Congruent Triangles:
- From the congruence of triangles ABD and ACD, we have:
- ∠BAD=∠CAD
- This implies:
- ∠BAP=∠CAP (since angles BAD and CAD are equal)
6. Establishing Congruence of Triangles ABP and ACP:
- Now, consider triangles ABP and ACP:
- AB=AC (from triangle ABC being isosceles)
- AP=AP (common side)
- ∠BAP=∠CAP (from step 5)
- By the SAS (Side-Angle-Side) congruence criterion, triangle ABP is congruent to triangle ACP:
△ABP≅△ACP
Conclusion:
Thus, we have shown that triangles ABP and ACP are congruent.
- We have two isosceles triangles: triangle ABC and triangle DBC.
- In triangle ABC, since it is isosceles, we have AB=AC.
- In triangle DBC, since it is also isosceles, we have BD=DC.
- Extend line AD such that it intersects line BC at point P.
- Both triangles ABP and ACP share the common side AP.
- In triangle ABD and triangle ACD:
- AB=AC (since triangle ABC is isosceles)
- AD=AD (common side)
- BD=DC (since triangle DBC is isosceles)
- By the SSS (Side-Side-Side) congruence criterion, triangle ABD is congruent to triangle ACD:
△ABD≅△ACD
- From the congruence of triangles ABD and ACD, we have:
- ∠BAD=∠CAD
- This implies:
- ∠BAP=∠CAP (since angles BAD and CAD are equal)
- Now, consider triangles ABP and ACP:
- AB=AC (from triangle ABC being isosceles)
- AP=AP (common side)
- ∠BAP=∠CAP (from step 5)
- By the SAS (Side-Angle-Side) congruence criterion, triangle ABP is congruent to triangle ACP:
△ABP≅△ACP
Thus, we have shown that triangles ABP and ACP are congruent.
Topper's Solved these Questions
- CHAPTERWISE REVISION (STAGE 3) Book:ICSEChapter:CHAPTERWISE REVISION (STAGE 3) Exercise:ISOSCELES TRIANGLES
Explore 2 Videos
- CHAPTERWISE REVISION (STAGE 3) Book:ICSEChapter:CHAPTERWISE REVISION (STAGE 3) Exercise:INEQUALITIES
Explore 7 Videos
- CHAPTERWISE REVISION (STAGE 3) Book:ICSEChapter:CHAPTERWISE REVISION (STAGE 3) Exercise:LOGARITHM
Explore 9 Videos
- CHAPTERWISE REVISION (STAGE 1) Book:ICSEChapter:CHAPTERWISE REVISION (STAGE 1) Exercise:Graphical solution
Explore 10 Videos
- CIRCLEBook:ICSEChapter:CIRCLEExercise:EXERCISE 17(D)
Explore 12 Videos
Similar Questions
Triangles ABC and DBC are two isosceles triangles on the same base BC and their vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that:
AP bisects angle BAC and BDC.
View SolutionΔABCand ΔDBCare two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig. 7.39). If AD is extended to intersect BC at P, show that (i) ΔABD≅ΔACD
(ii) ΔABP≅ΔACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC

View SolutionABC and DBC are two isosceles triangles on the same base BC (see Fig. 7.33). Show that∠ABD =∠ACD

View SolutionABC and DBC are two triangle on the same base BC such that A and D lie on the opposite sides of BC,AB=AC and DB =DC ,Show that AD is the perpedicular bisector of BC.
View SolutionIn figure ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that ar(ABC)ar(DBC)=AODO.

View SolutionThe given figure shows two isosceles triangles ABC and DBC with common base BC. AD is extended to intersect BC at point P. Show that:
ΔABD=ΔACD
(ii) ΔABP=ΔACP
(iii) AP bisects ∠BDC
(iv) AP is perpendicular bisector of BC.
View SolutionΔABCandΔDBC are on same base BC and their vertices A and D are on opposite sides of BC. It is given that:
area of ΔABC= area of ΔDBC
Prove that BC bisects the line segment AD.
View Solution△ABCand△DBC are two triangles on the same base BC. A and D lies on opposite sides of BC. Prove that ar(△ABC)ar(△DBC)=AODO
View SolutionConstruct two isosceles triangles ABC and DBC, with common base BC and the points A and D on opposite sides of BC, given that BC = 8 cm, AB = AC = 4.5 cm, and DB = DC = 5 cm.
View SolutionIn isosceles triangle ABC, D is a point on the base BC produced. Prove that AD>AB.

View Solution
