Question

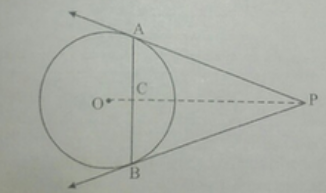
From an external point P, tangents PA and PB are drawn to a circle. CE is a tangent to the circle at D which intersect PA and PB at point E and C respectvely. If AP=15 cm, find the permeter of the $△PEC.$

Answer

30cm

Explanation

To solve the problem step by step, we will use the properties of tangents to a circle from an external point.

Step 1: Understand the Configuration
We have a circle with an external point P from which two tangents PA and PB are drawn to the circle. The lengths of these tangents are equal due to the property of tangents from an external point.

Step 2: Assign Known Values
Given that \( AP = 15 \, \text{cm} \), we can denote:
- \( PA = 15 \, \text{cm} \)
- Since \( PA = PB \), we also have \( PB = 15 \, \text{cm} \).

Step 3: Use the Tangent Properties
From the point P, the lengths of the tangents to the circle are equal:
- Let \( EA = ED = x \)
- Let \( CB = CD = y \)

Step 4: Find the Lengths of Segments
We need to find the lengths of segments \( PC \), \( CE \), and \( PE \):
- \( PC = PB - CB = 15 - y \)
- \( CE = CD + DE = y + x \)
- \( PE = PA - AE = 15 - x \)

Step 5: Calculate the Perimeter of Triangle PEC
The perimeter of triangle PEC is given by:
\( \text{Perimeter} = PC + CE + PE \)
Substituting the lengths we found:
\( \text{Perimeter} = (15 - y) + (y + x) + (15 - x) \)
Simplifying this expression:
\( \text{Perimeter} = 15 - y + y + x + 15 - x = 15 + 15 = 30 \, \text{cm} \)

Final Answer
Thus, the perimeter of triangle PEC is \( 30 \, \text{cm} \).

---