The escape velocity for a planet is . A particle starts from rest at a large distance from the planet, reaches the planet only under gravitational attraction, and passes through a smooth tunnel through its centre. Its speed at the centre of the planet will be
The correct Answer is:C
To find the speed of a particle at the center of a planet when it starts from rest at a large distance and falls under gravitational attraction, we can use the principle of conservation of energy. Here’s a step-by-step solution:
Step 1: Understand the initial conditions
- The particle starts from rest at a large distance from the planet.
- At this point, the potential energy (PE) is considered to be zero, and the kinetic energy (KE) is also zero because the particle is at rest.
Step 2: Define the escape velocity
- The escape velocity ve for a planet is given by the formula:
ve=√2GMR
where G is the gravitational constant, M is the mass of the planet, and R is the radius of the planet.
Step 3: Apply conservation of energy
- As the particle falls towards the planet, its potential energy decreases while its kinetic energy increases.
- At a large distance (point A), the total energy is:
EA=KEA+PEA=0+0=0
- At the center of the planet (point O), the total energy is:
EO=KEO+PEO=12mv2+UO
where v is the speed at the center and UO is the potential energy at the center.
Step 4: Calculate potential energy at the center
- The potential energy at the center of the planet can be derived from gravitational potential energy:
UO=−GMmR(for a uniform sphere)
where m is the mass of the particle.
Step 5: Set up the energy conservation equation
- From conservation of energy, we have:
0=12mv2−GMmR
- Rearranging gives:
12mv2=GMmR
Step 6: Solve for v
- Dividing both sides by m and multiplying by 2:
v2=2GMR
- Taking the square root:
v=√2GMR=ve
Step 7: Adjust for the gravitational potential inside the planet
- However, we need to consider that the particle is not just falling freely but also passing through the tunnel. The effective potential energy inside the planet is given by:
U=−3GM2R
- Thus, the speed at the center becomes:
v=√3GMR=√32ve
Final Answer
- Therefore, the speed of the particle at the center of the planet is:
v=√32ve
- The particle starts from rest at a large distance from the planet.
- At this point, the potential energy (PE) is considered to be zero, and the kinetic energy (KE) is also zero because the particle is at rest.
- The escape velocity ve for a planet is given by the formula:
ve=√2GMR
where G is the gravitational constant, M is the mass of the planet, and R is the radius of the planet.
- As the particle falls towards the planet, its potential energy decreases while its kinetic energy increases.
- At a large distance (point A), the total energy is:
EA=KEA+PEA=0+0=0
- At the center of the planet (point O), the total energy is:
EO=KEO+PEO=12mv2+UO
where v is the speed at the center and UO is the potential energy at the center.
- The potential energy at the center of the planet can be derived from gravitational potential energy:
UO=−GMmR(for a uniform sphere)
where m is the mass of the particle.
- From conservation of energy, we have:
0=12mv2−GMmR
- Rearranging gives:
12mv2=GMmR
- Dividing both sides by m and multiplying by 2:
v2=2GMR
- Taking the square root:
v=√2GMR=ve
- However, we need to consider that the particle is not just falling freely but also passing through the tunnel. The effective potential energy inside the planet is given by:
U=−3GM2R
- Thus, the speed at the center becomes:
v=√3GMR=√32ve
- Therefore, the speed of the particle at the center of the planet is:
v=√32ve
Similar Questions
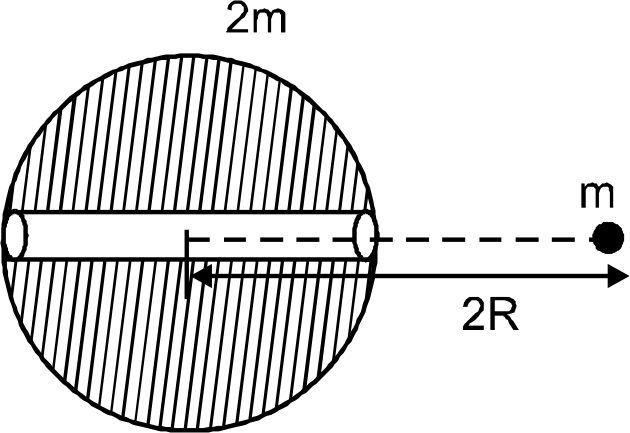
A solid spherical planet of mass 2m and radius 'R' has a very small tunnel along its diameter. A small cosmic particle of mass m is at a distance 2R from the centre of the planet as shown. Both are initially at rest, and due to gravitational attraction, both start moving toward each other. After some time, the cosmic particle passes through the centre of the planet. (Assume the planet and the cosmic particle are isolated from other planets)
View SolutionIf the angular velocity of a planet about its own axis is halved, the distance of geostationary satellite of this planet from the centre of the centre of the planet will become
View SolutionShow that the temperature of a planet varies inversely as the square root of its distance from the sum.
View SolutionEscape velocity from a planet is ve. If its mass is increased to 16 times and its radius is increased to 4 times, then the new escape velocity would be
View SolutionA point P lies on the axis of a fixed ring of mass M and radius a, at a distance a from its centre C. A small particle starts from P and reaches C under gravitational attraction only. Its speed at C will be.
View SolutionA point P lies on the axis of a fixed ring of mass M and radius a, at a distance a from its centre C. A small particle starts from P and reaches C under gravitational attraction only. Its speed at C will be.
View SolutionAn artificial satellite relolves around a planet for which gravitational force (F) varies with distance r from its centres as F∝r2 If v0 its orbital speed , then
View SolutionStatement I: In free space a uniform spherical planet of mass M has a smooth narrow tunnel along its diameter. This planet and another superdense small particle of mass M start approaching towards each other from rest under action of their gravitational forces. When the particle passes through the centre of the planet, sum of kinetic energies of both the bodies is maximum.
Statement II: When the resultant of all forces acting on a particle or a particle like object (initially at rest) is constant in direction, the kinetic energy of the particle keeps on increasing.
View SolutionWhich of the following graphs represents the motion of the planet moving about the Sun. T is the periode of revolution and r is the average distance (from centre to centre) between the sun and the planet
View SolutionA far off planet is estimated to be at a distance D from the earth. If its diametrically opposite extremes subtend an angle θ at an observatory situated on the earth, the approximate diameter of the planet is
View Solution
