A battery has an open circuit potential difference 10 V between the terminals. When loads 9Ωand4Ω are connected one by one across the battery , the power in the load resistance is the same. The amount of heat approximately generated in one second in the load when a load of 5Ω Is connected across the battery will be
10.4J
9 J
6.8J
4.13J
The correct Answer is:D
To solve the problem step by step, we will follow the logical sequence of calculations as outlined in the video transcript.
Step 1: Identify Given Values
- Open circuit potential difference (EMF, E) = 10 V
- Load resistances: R1 = 9 Ω, R2 = 4 Ω
- Load resistance for which we need to find heat (RL) = 5 Ω
Step 2: Key Concept for Heat Generation
The heat generated in a resistor can be calculated using the formula:
H=V⋅I⋅t
Since V=I⋅R, we can also express heat as:
H=I2⋅R⋅t
Where:
- H = heat generated
- I = current through the load
- R = resistance of the load
- t = time (in seconds)
Step 3: Find Internal Resistance
To find the internal resistance of the battery, we need to use the condition that the power in the load resistances (9 Ω and 4 Ω) is the same when connected one by one.
Using the power formula:
P=I2⋅R
For the two loads:
P1=I21⋅R1
P2=I22⋅R2
Since P1=P2:
I21⋅9=I22⋅4
Step 4: Express Currents in Terms of EMF and Resistances
Using Ohm's law, we can express the currents as:
I1=ER1+r=109+r
I2=ER2+r=104+r
Step 5: Set Up the Equation
Substituting I1 and I2 into the power equation:
(109+r)2⋅9=(104+r)2⋅4
Step 6: Cross Multiply and Simplify
Cross-multiplying gives:
100⋅9⋅(4+r)2=100⋅4⋅(9+r)2
This simplifies to:
9(4+r)2=4(9+r)2
Step 7: Expand and Solve for r
Expanding both sides:
9(16+8r+r2)=4(81+18r+r2)
This leads to:
144+72r+9r2=324+72r+4r2
Simplifying gives:
5r2−180=0
Thus:
r2=36⟹r=6Ω
Step 8: Calculate Current for 5 Ω Load
Now, we can find the current when a 5 Ω load is connected:
I=ERL+r=105+6=1011A
Step 9: Calculate Heat Generated
Using the heat formula:
H=I2⋅RL⋅t=(1011)2⋅5⋅1
Calculating this gives:
H=100121⋅5≈4.13J
Final Answer
The amount of heat approximately generated in one second in the load when a load of 5 Ω is connected across the battery is approximately 4.13 J.
- Open circuit potential difference (EMF, E) = 10 V
- Load resistances: R1 = 9 Ω, R2 = 4 Ω
- Load resistance for which we need to find heat (RL) = 5 Ω
The heat generated in a resistor can be calculated using the formula:
H=V⋅I⋅t
Since V=I⋅R, we can also express heat as:
H=I2⋅R⋅t
Where:
- H = heat generated
- I = current through the load
- R = resistance of the load
- t = time (in seconds)
To find the internal resistance of the battery, we need to use the condition that the power in the load resistances (9 Ω and 4 Ω) is the same when connected one by one.
P=I2⋅R
For the two loads:
P1=I21⋅R1
P2=I22⋅R2
Since P1=P2:
I21⋅9=I22⋅4
Using Ohm's law, we can express the currents as:
I1=ER1+r=109+r
I2=ER2+r=104+r
Substituting I1 and I2 into the power equation:
(109+r)2⋅9=(104+r)2⋅4
Cross-multiplying gives:
100⋅9⋅(4+r)2=100⋅4⋅(9+r)2
This simplifies to:
9(4+r)2=4(9+r)2
Expanding both sides:
9(16+8r+r2)=4(81+18r+r2)
This leads to:
144+72r+9r2=324+72r+4r2
Simplifying gives:
5r2−180=0
Thus:
r2=36⟹r=6Ω
Now, we can find the current when a 5 Ω load is connected:
I=ERL+r=105+6=1011A
Using the heat formula:
H=I2⋅RL⋅t=(1011)2⋅5⋅1
Calculating this gives:
H=100121⋅5≈4.13J
The amount of heat approximately generated in one second in the load when a load of 5 Ω is connected across the battery is approximately 4.13 J.
Similar Questions
A battery has an open circuit potential difference of 6V between its terminals. When a load resistance of 60Ω is connected across the battery,the total power supplied by the bttery is 0.4W. What should be the load resistance R, so that maximum power will be dissipated in R. Calculated this power. What is the total power supplied by the battery when such a load is connected?
View SolutionThe potential difference between the terminals of a battery of emf 6.0V and internal resistance1(Ω)drops to 5.8 V when connected across an external resistor. Find the resistance of the external resistor.
View SolutionTwo resistors of resistances 2Ωand4Ω when connected to a battery will have :
View SolutionA battery supplies 150W and 196 W power to two resistance of 6Ω and 4Ω when they are connected separately to it. The internal resistance of the battery is:-
View SolutionA battery of e.m.f. 15 V and internal resistance 3Ω is connected to two resistors 3 Ω and 6 Ω connected in parallel. Find :
the p.d. between the terminals of the battery,
View SolutionA battery of EMF E produces currents 4 A and 3 A when connected to external resistance 1Ω and 2Ω respectively. The internal resistance of the battery is
View SolutionA 5V battery with internal resistance 2Ω and a 2V battery with internal resistance1Ω are connected to a 10Ω resistor as shown in the figure.
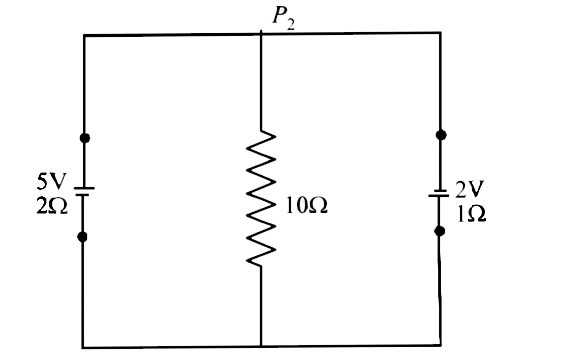
The current in the 10Ω resistor is
View SolutionA 5 V battery with internal resistance 1Ω and a 2 V battery with internal resistance 0.5Ω are connected to a 3Ω resistor as shown in the figure.The current in the 3Ω resistor is
View SolutionTwo cells having an internal resistance of 0.2Ω and 0.4Ω are connected in parallel (same polarity) the voltage across the battery is 1.5 V. If the emf of one cell is 1.2 V, then the emf of second cell is
View SolutionThere identical point masses, each of mass 1 kg lie in the xy-plane at points (0, 0), (0, 0.2 m) and (0, 2m, 0). The net gravitational force on the mass at the origin is:
View Solution

