Which of the following graphs correctly represents the relation between In (E) and In (T), where E is the amount of radiation emitted per unit time from a unit area of the body and T is the absolute temperature ?
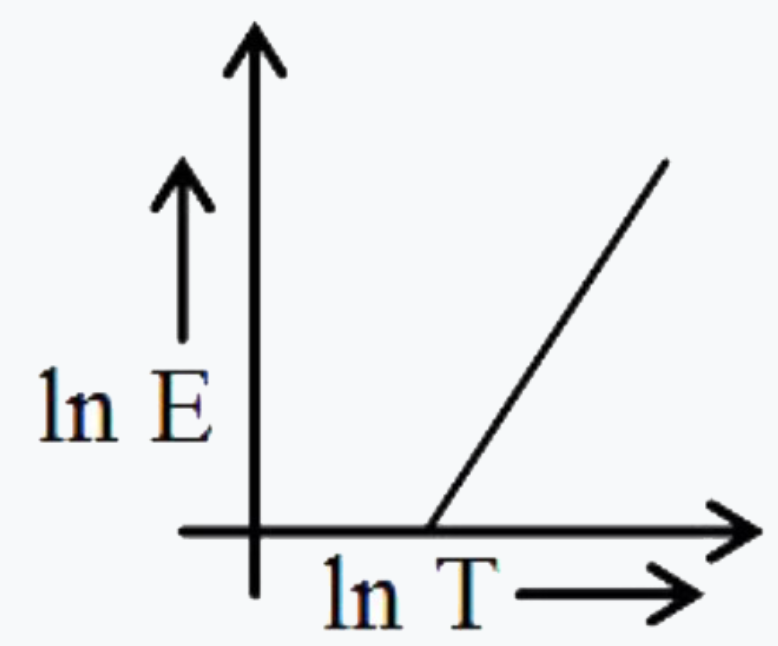
The correct Answer is:C
To solve the problem, we need to establish the relationship between the amount of radiation emitted per unit time from a unit area of the body (E) and the absolute temperature (T). We will use Stefan-Boltzmann Law, which states that the power (P) emitted by a black body per unit area is proportional to the fourth power of its absolute temperature (T).
1. Understand the Stefan-Boltzmann Law:
The law states that:
P=σAT4
where P is the power emitted, A is the area, and σ is the Stefan-Boltzmann constant.
2. Define E:
The amount of radiation emitted per unit time from a unit area (E) can be expressed as:
E=PA
Substituting the expression for P:
E=σAT4A=σT4
3. Take the Natural Logarithm:
To find the relationship in terms of natural logarithms, we take the natural logarithm of both sides:
ln(E)=ln(σT4)
4. Apply Logarithmic Properties:
Using the properties of logarithms, we can expand the right side:
ln(E)=ln(σ)+ln(T4)=ln(σ)+4ln(T)
5. Rearranging the Equation:
We can rearrange this equation to match the form y=mx+c:
ln(E)=4ln(T)+ln(σ)
Here, y=ln(E), x=ln(T), the slope m=4, and the y-intercept c=ln(σ).
6. Analyze the y-intercept:
Since σ is a constant between 0 and 1, ln(σ)<0. This means that the y-intercept is negative.
7. Conclusion about the Graph:
The graph of ln(E) versus ln(T) will be a straight line with a slope of 4 and a negative y-intercept.
Final Answer:
The correct graph will be a straight line with a positive slope (4) and a negative y-intercept.
The law states that:
P=σAT4
where P is the power emitted, A is the area, and σ is the Stefan-Boltzmann constant.
The amount of radiation emitted per unit time from a unit area (E) can be expressed as:
E=PA
Substituting the expression for P:
E=σAT4A=σT4
To find the relationship in terms of natural logarithms, we take the natural logarithm of both sides:
ln(E)=ln(σT4)
Using the properties of logarithms, we can expand the right side:
ln(E)=ln(σ)+ln(T4)=ln(σ)+4ln(T)
We can rearrange this equation to match the form y=mx+c:
ln(E)=4ln(T)+ln(σ)
Here, y=ln(E), x=ln(T), the slope m=4, and the y-intercept c=ln(σ).
Since σ is a constant between 0 and 1, ln(σ)<0. This means that the y-intercept is negative.
The graph of ln(E) versus ln(T) will be a straight line with a slope of 4 and a negative y-intercept.
The correct graph will be a straight line with a positive slope (4) and a negative y-intercept.
Similar Questions
Which of the following series correctly represents relations between the elements from X to Y ?
X→Y

View SolutionWhich of the following graph represents the variation of resistivity (ρ) with temperature (T) for copper?
View SolutionWhich of the following graph correctly represents velocity-time relationship for a particle released from rest to fall freely under gravity ?
View SolutionWhich of the following graph(s) represent retardation ? [v : velocity, t : time, x : position]
View SolutionWhich of the following x-t graphs represents the distance-time variation of a body released from the top of a buiding?
View SolutionWhich graph correctly represents variation between relaxation time (t) of gas molecules with absolute temperature of gas T.
View SolutionWhich graph correctly represents variation between relaxation time (t) of gas molecules with absolute temperature of gas T.
View SolutionWhich of the following is the graph between the height (h) of a projectile and time (t), when it is projected from the ground
View SolutionA body is thrown vertically upwards. Which one of the following graphs correctly represent the velocity vs time?
View SolutionWhich one of the following is vm−T graph for perfectly black body ? vm is the frequency of radition with maximum intensity. T is the absolute temperature
View Solution
