The wavelength of Kα X-rays of two metals AandB are4/1875Rand1/675R, respectively , where R is rydberg 's constant. The number of electron lying between AandB according to this lineis
3
6
5
4
The correct Answer is:D
To solve the problem, we need to determine the number of electrons (or atomic numbers) lying between two metals A and B based on their K-alpha X-ray wavelengths. The wavelengths are given as:
- For metal A: λA=41875R
- For metal B: λB=1675R
Where R is Rydberg's constant.
Step 1: Use the Rydberg formula for K-alpha X-rays
The wavelength of the K-alpha X-rays can be related to the atomic number Z of the metal using the Rydberg formula:
1λ=R(Z−1)2(1n21−1n22)
For K-alpha transitions, n1=1 and n2=2. Thus, the formula simplifies to:
1λ=R(Z−1)2(1−14)=R(Z−1)2(34)
Step 2: Calculate Z for metal A
For metal A, substituting λA:
1λA=18754R
Equating this to the Rydberg formula:
18754R=R(ZA−1)2(34)
Cancelling R from both sides:
18754=(ZA−1)2(34)
Multiplying both sides by 43:
625=(ZA−1)2
Taking the square root:
ZA−1=25⟹ZA=26
Step 3: Calculate Z for metal B
For metal B, substituting λB:
1λB=675R
Equating this to the Rydberg formula:
675=R(ZB−1)2(34)
Cancelling R:
675=(ZB−1)2(34)
Multiplying both sides by 43:
900=(ZB−1)2
Taking the square root:
ZB−1=30⟹ZB=31
Step 4: Determine the number of electrons between metals A and B
Now we have:
- ZA=26
- ZB=31
The number of electrons (or atomic numbers) lying between A and B is:
ZA+1,ZA+2,ZA+3,ZA+4(which are 27, 28, 29, 30)
Thus, the number of electrons between metals A and B is:
31−26−1=4
Final Answer
The number of electrons lying between metals A and B is 4.
---
- For metal B: λB=1675R
- ZB=31
Similar Questions
The radii of circular orbits of two satellite A and B of the earth are 4R and R, respectively. If the speed of satellite A is 3v, then the speed of satellite B will be
View SolutionThe densitis of two solid spheres A and B of the same radii R very with radial distance raspA(r)=k(rR)andpB(r)=k(r(R)5, respectively, where k is a constant . The moments of inertia of the inividual spheres about axes passing throgh their centres are IAandIB respectively. if IBIA=n10, the value of n is
View Solution
Knowledge Check
Two soap bubbles A and B have radii r1andr2 respectively. If r1<r2 than the excess pressure inside
Abubbles A and B will be equalBbubbles A will be less than that in bubbles BCbubbles A will be greater than that in bubble BDbubbles A and B will be zero.
If voltage applied to X -ray tube increased fromV=10kV to 20kV. The wave length interval between Kα−line and short wave cut off continuous X-ray increases by factor of 3Rhce=13.6V where R is Rydberg constant h is plank's constant c is speed of light in vacuum and e is charge on electron
View SolutionA,B and C are voltmeters of resistances R,1.5R and 3R respectively. When some potential difference is applied between x and y the voltmeter readings are VA,VBandVC, then
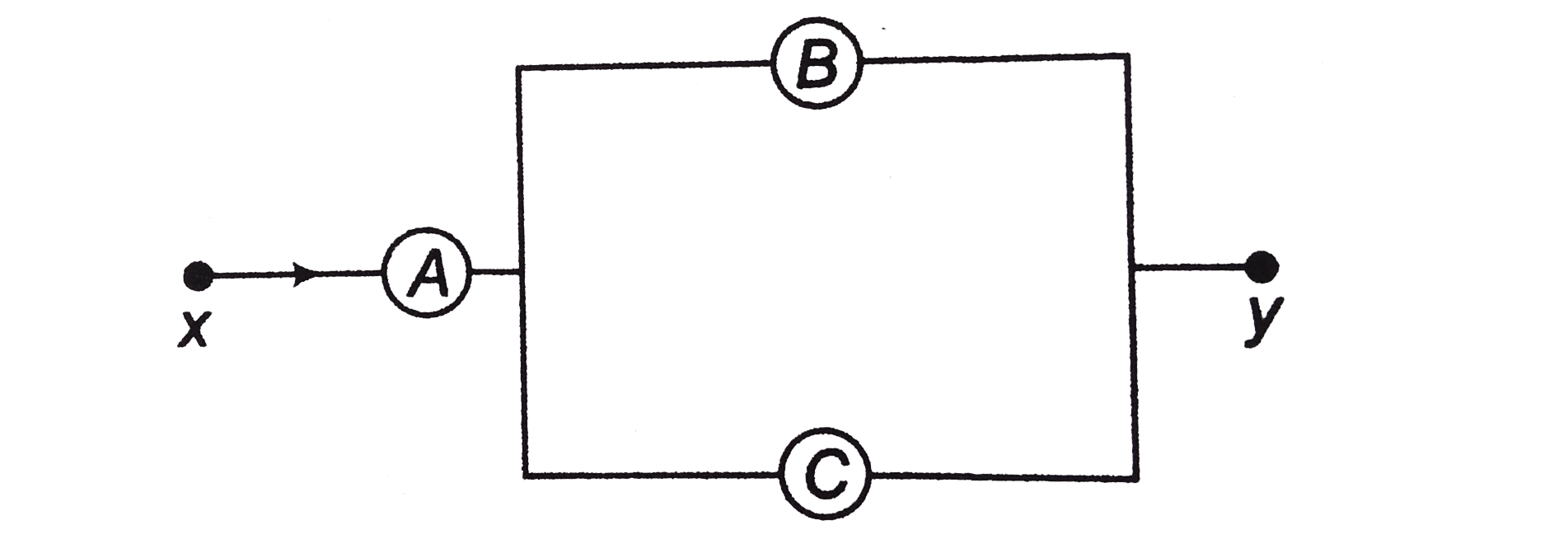
View SolutionA,B and C are voltmeters of resistances R,1.5R and 3R respectively. When some potential difference is applied between x and y the voltmeter readings are VA,V_BandV_C, then

View SolutionA,B and C are voltmeters of resistances R,1.5R and 3R respectively. When some potential difference is applied between x and y the voltmeter readings are VA,V_BandV_C, then

View SolutionA,B and C are voltmeters of resistances R,1.5R and 3R respectively. When some potential difference is applied between x and y the voltmeter readings are VA,V_BandV_C, then

View SolutionIf A and B are coefficients of xr and xn−r respectively in the expansion of (1+x)n, then
View SolutionThe value of resistance of an unknown resistor is calculated using the formula R=V/I where V and I are the readings of the voltmeter and the ammeter, respectivley. Consider the circuits below. The internal resistances of the voltmeter and the ammeter (RVandRG,respectively) are finite and nonzero.
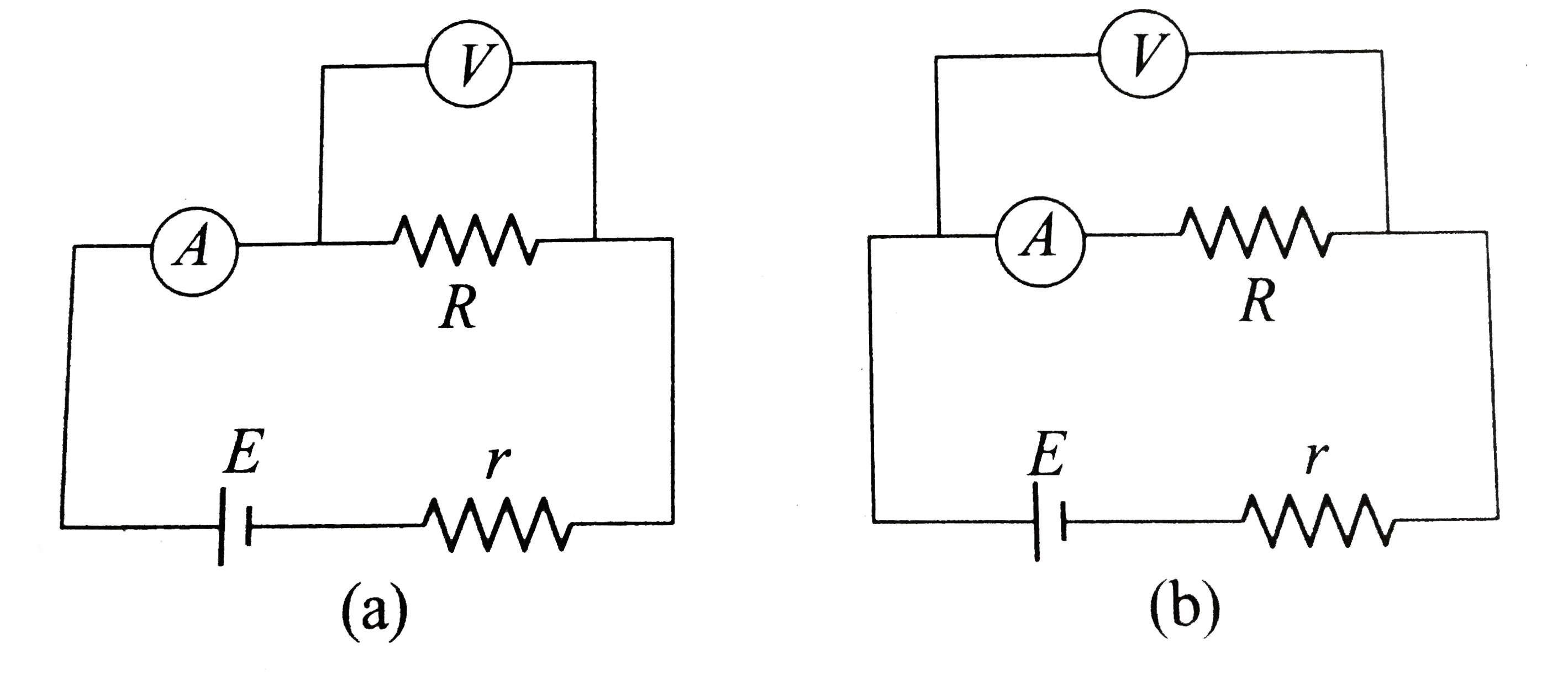 ,
,
Let RAandRB be the calculated values in the two cases A and B , respectively.
If the resistance of voltmeter is RV=1kΩ and the percentage error in the measurement of R (the value of R is nearly 10Ω)is
View Solution
