Doubtnut Promotions Sticky

Let z 1 and z 2 be complex numbers such that z 1 2 - 4 z 2 = 16 + 20 i and the roots α and β of x 2 + z 1 x + z 2 + m = 0 for some complex number m satisfies | α - β | = 2 7 . The value of | m | , when a r e ( m ) is maximum

A

7

B

28 - 41

C

41

D

2 6 - 4

Text Solution
Verified by Experts

The correct Answer is:D

( d ) We have α + β = - z 1 and α β = z 2 + m
( α - β ) 2 = ( α + β ) 2 - 4 α β
= z 1 ( ^ ( 2 ) - 4 z 1 - 4 m
= 16 + 20 i - 4 m
Since | α - β | = 2 7 , we have | 4 + 5 i - m | = 7 …… ( i )
Therfore, m lies on a circle having centre at ( 4 , 5 ) and radius = 7 .
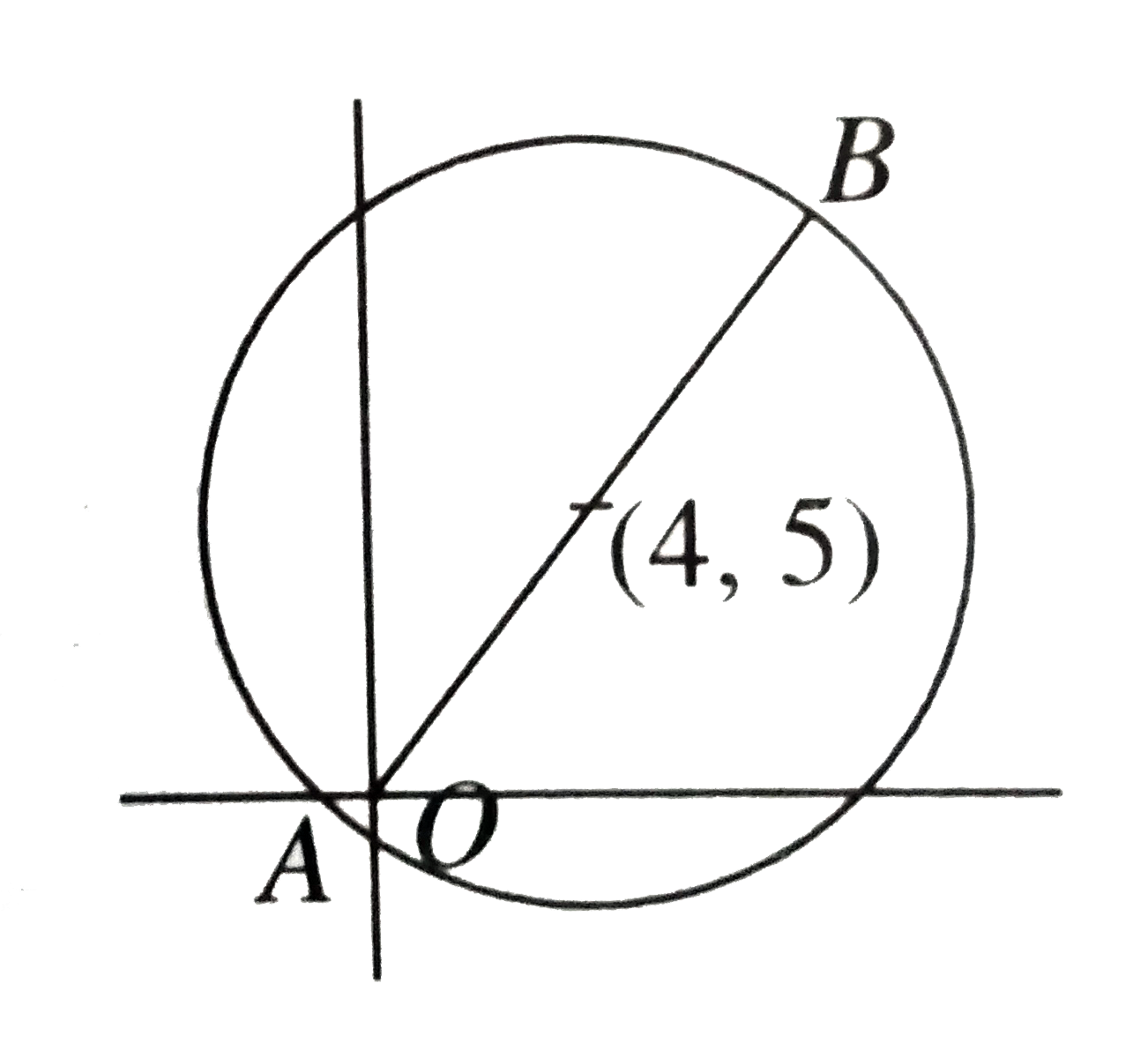
ocr_image
| m | max = O B = 7 + 41
Maximum argument of m is π which occurs at point A ,
Let m = x + i y
For point A , m = x
So from ( i ) , | 4 + 5 i - x | = 7
or ( x - 4 ) 2 = 24
⇒ x - 4 = ± 2 6
⇒ x = 4 ± 2 6
For point A , x = 2 6 - 4

Doubtnut Promotions Banner
|
Updated on:21/07/2023
Class 12MATHSCOMPLEX NUMBERS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    Let z1 and z2 be complex numbers such that z21−4z2=16+20i and the roots α and β of x2+z1x+z2+m=0 for some complex number m satisfies |α−β|=2√7.
    The maximum value of |m| is

    A14
    B2√7
    C7+√41
    D2√6−4
  • Question 2 - Select One

    Let z1andz2 be complex numbers such that z21−4z2=16+20i and the roots αandβ of x2+z1x+z2+m=0 for some complex number m satisfies |α−β|=2√7.
    The minimum value of |m| is

    A7
    B28−√41
    C√41
    D7−√41
  • Question 3 - Select One

    Let z1 and z2 be complex numbers such that z21−4z2=16+20i and the roots α and β of x2+z1x+z2+m=0 for some complex number m satisfies |α−β|=2√7.
    The locus of the complex number m is a curve

    Astraight line
    Bcircle
    Cellipse
    Dhyperbola