State whether the statements are true (T) or (F) false.
All rhombuses are squares.
To determine whether the statement "All rhombuses are squares" is true or false, we need to analyze the properties of rhombuses and squares.
1. Definition of a Rhombus:
A rhombus is a quadrilateral with all four sides of equal length. However, the angles in a rhombus are not necessarily right angles. The diagonals of a rhombus bisect each other at right angles but are not equal in length.
2. Definition of a Square:
A square is also a quadrilateral with all four sides of equal length, but it has additional properties: all angles are right angles (90 degrees), and the diagonals are equal in length and bisect each other at right angles.
3. Comparison of Properties:
- Both shapes have equal side lengths.
- In a square, all angles are equal (90 degrees), while in a rhombus, the angles can vary.
- The diagonals of a square are equal, while the diagonals of a rhombus are not necessarily equal.
4. Conclusion:
Since a rhombus does not have all the properties of a square (specifically, the angles and the equality of diagonals), we can conclude that not all rhombuses are squares.
Therefore, the statement "All rhombuses are squares" is False (F).
A rhombus is a quadrilateral with all four sides of equal length. However, the angles in a rhombus are not necessarily right angles. The diagonals of a rhombus bisect each other at right angles but are not equal in length.
A square is also a quadrilateral with all four sides of equal length, but it has additional properties: all angles are right angles (90 degrees), and the diagonals are equal in length and bisect each other at right angles.
- Both shapes have equal side lengths.
- In a square, all angles are equal (90 degrees), while in a rhombus, the angles can vary.
- The diagonals of a square are equal, while the diagonals of a rhombus are not necessarily equal.
Since a rhombus does not have all the properties of a square (specifically, the angles and the equality of diagonals), we can conclude that not all rhombuses are squares.
Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - APPLICATIONS, GAMES AND PUZZLES
3 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTBOOK - NCERT EXEMPLARCHAPTER - SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTEXERCISE - Exercise
52 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
Similar Questions
State whether the statements are true (T) or (F) false.
All kites are squares.
View SolutionState whether the statements are true (T) or (F) false.
All squares are rectangles.
View SolutionState whether the statements are true (T) or (F) false.
A rhombus can be constructed uniquely if both diagonals are given.
View SolutionState whether the statements are true (T) or (F) false.
All rectangles are parallelograms.
View SolutionState whether the statements are true (T) or (F) false.
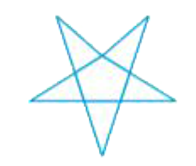 is a polygon.
is a polygon.
View SolutionState whether the statements are true or falsea−b=b−a
View SolutionState whether the statements are true (T) or (F) false.
Every rhombus is a trapezium.
View SolutionState whether the statements are true (T) or (F) false.
Diagonals of a rhombus are equal and perpendicular to each other.
View SolutionState whether the statements are true or falsea×b=b×a
View SolutionState whether the statements are true or falsea÷a=b÷a
View Solution
