State whether the statements are true (T) or (F) false.
The sum of interior angles and the sum of exterior angles taken in an order are equal in case of quadrilaterals only.
To determine whether the statement "The sum of interior angles and the sum of exterior angles taken in an order are equal in case of quadrilaterals only" is true or false, we can follow these steps:
Step 1: Understand the sum of interior angles of a quadrilateral.
The formula for the sum of the interior angles of a polygon is given by:
Sum of interior angles=180(n−2)
where n is the number of sides of the polygon. For a quadrilateral, n=4.
Step 2: Calculate the sum of interior angles for a quadrilateral.
Substituting n=4 into the formula:
Sum of interior angles=180(4−2)=180×2=360 degrees
Step 3: Understand the sum of exterior angles of any polygon.
The sum of the exterior angles of any polygon is always:
Sum of exterior angles=360 degrees
Step 4: Compare the sums of interior and exterior angles.
For a quadrilateral:
- Sum of interior angles = 360 degrees
- Sum of exterior angles = 360 degrees
Step 5: State the conclusion.
Since both the sum of interior angles and the sum of exterior angles for a quadrilateral are equal (both are 360 degrees), the statement is True (T).
Final Answer:
The statement is True (T).
---
The formula for the sum of the interior angles of a polygon is given by:
Sum of interior angles=180(n−2)
where n is the number of sides of the polygon. For a quadrilateral, n=4.
Substituting n=4 into the formula:
Sum of interior angles=180(4−2)=180×2=360 degrees
The sum of the exterior angles of any polygon is always:
Sum of exterior angles=360 degrees
For a quadrilateral:
- Sum of interior angles = 360 degrees
- Sum of exterior angles = 360 degrees
Since both the sum of interior angles and the sum of exterior angles for a quadrilateral are equal (both are 360 degrees), the statement is True (T).
The statement is True (T).
Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - APPLICATIONS, GAMES AND PUZZLES
3 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTBOOK - NCERT EXEMPLARCHAPTER - SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTEXERCISE - Exercise
52 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
Similar Questions
State whether the statements are true (T) or (F) false.
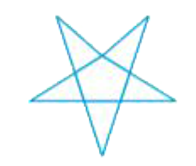 is a polygon.
is a polygon.
View SolutionState whether the statements are true (T) or (F) false.
If the sum of interior angles is double the sum of exterior angles taken in an order of a polygon, then it is a hexagon.
View SolutionState whether the statements are true (T) or (F) false.
All squares are rectangles.
View SolutionState whether the statements are true (T) or (F) false.
 is a concave pentagon.
is a concave pentagon.
View SolutionState whether the statements are true (T) or (F) false.
All kites are squares.
View SolutionState whether the statements are true (T) or (F) false.
Every square is a trapezium.
View SolutionState whether the statements are true (T) or (F) false.
All rhombuses are squares.
View SolutionState whether the statements are true (T) or (F) false.
Diagonals of a rectangle are equal.
View SolutionState whether the statements are true (T) or (F) false.
Every kite is a parallelogram.
View SolutionState whether the statements are true (T) or (F) false.
Every trapezium is a rectangle.
View Solution