Question

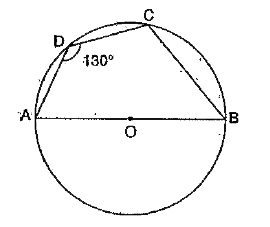
ABCD is a quadrilateral whose side AB is the diameter of a circle through A, B, C and D. If $\angle ADC={130}^{\circ }$, then the measure of $\angle BAC$ is:
ABCD एक चतुर्भुज है जिसकी भुजा AB, A, B, C और D से गुजरने वाले वृत्त का व्यास है | यदि $\angle ADC={130}^{\circ }$ है, तो कोण BAC का मान ज्ञात करें |

Options

A. `40^@`
B. `45^@`
C. `35^@`
D. `50^@`

Explanation

To find the measure of angle $$\angle BAC$$ in the quadrilateral $$ABCD$$ where $$AB$$ is the diameter of a circle and $$\angle ADC={130}^{\circ }$$, we can follow these steps:

1. Understanding the Circle and Quadrilateral:
- Since \( AB \) is the diameter of the circle, any angle subtended by \( AB \) at the circumference (like \( \angle ACB \) or \( \angle ADC \)) will be a right angle (90 degrees) due to the property of the circle.

2. Finding \( \angle ABC \):
- Given \( \angle ADC = 130^\circ \), we can find \( \angle ABC \) using the property of cyclic quadrilaterals, which states that opposite angles sum up to \( 180^\circ \).
- Therefore, \( \angle ABC = 180^\circ - \angle ADC = 180^\circ - 130^\circ = 50^\circ \).

3. Analyzing Triangle \( ABC \):
- Now, we consider triangle \( ABC \). We know:
- \( \angle ACB = 90^\circ \) (since \( AB \) is the diameter).
- \( \angle ABC = 50^\circ \) (calculated in the previous step).

4. Finding \( \angle BAC \):
- The sum of angles in triangle \( ABC \) is \( 180^\circ \). Thus, we can write:
\( \angle BAC + \angle ABC + \angle ACB = 180^\circ \)
\( \angle BAC + 50^\circ + 90^\circ = 180^\circ \)
\( \angle BAC + 140^\circ = 180^\circ \)
\( \angle BAC = 180^\circ - 140^\circ = 40^\circ \)

Final Answer:
The measure of \( \angle BAC \) is \( 40^\circ \).

---