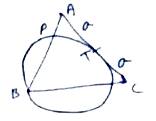
In ∠ABC, AB=AC . A circle drawn through B touches AC at D and intersects AB at P. If D is the mid point of AC and AP = 2.5 cm, then AB is equal to:
त्रिभुज ABC में, AB = AC है | B से खींचा गया एक वृत्त AC को D पर स्पर्श करता है तथा AB को P पर
काटता है | यदि D, AC का मध्य बिंदु है एवं AP = 2.5 सेमी है, तो AB का मान किसके बराबर है ?
9 cm
7.5 cm
12.5 cm
10 cm
To solve the problem step by step, we will use the properties of triangles and circles, particularly focusing on the relationships between the segments defined in the problem.
1. Identify the Given Information:
- In triangle ABC, we have AB = AC.
- A circle drawn through point B touches line AC at point D and intersects line AB at point P.
- D is the midpoint of AC.
- AP = 2.5 cm.
2. Define the Lengths:
- Since D is the midpoint of AC, we can denote the lengths as follows:
- Let AD = DC = x.
- Therefore, AC = AD + DC = x + x = 2x.
3. Use the Tangent-Secant Theorem:
- According to the tangent-secant theorem, we know that:
AD2=AP×AB
- Substituting the known values:
x2=2.5×AB
4. Express AB in Terms of x:
- Since we also have AC = 2x, and we know AB = AC, we can write:
AB=2x
- Now substituting this into the equation from the tangent-secant theorem:
x2=2.5×(2x)
- Simplifying gives:
x2=5x
5. Rearranging the Equation:
- Rearranging the equation:
x2−5x=0
- Factoring out x:
x(x−5)=0
- This gives us two solutions: x = 0 or x = 5. Since x represents a length, we take x = 5.
6. Calculate AB:
- Now substituting back to find AB:
AB=2x=2×5=10 cm
Final Answer:
AB = 10 cm.
- In triangle ABC, we have AB = AC.
- A circle drawn through point B touches line AC at point D and intersects line AB at point P.
- D is the midpoint of AC.
- AP = 2.5 cm.
- Since D is the midpoint of AC, we can denote the lengths as follows:
- Let AD = DC = x.
- Therefore, AC = AD + DC = x + x = 2x.
- According to the tangent-secant theorem, we know that:
AD2=AP×AB
- Substituting the known values:
x2=2.5×AB
- Since we also have AC = 2x, and we know AB = AC, we can write:
AB=2x
- Now substituting this into the equation from the tangent-secant theorem:
x2=2.5×(2x)
- Simplifying gives:
x2=5x
- Rearranging the equation:
x2−5x=0
- Factoring out x:
x(x−5)=0
- This gives us two solutions: x = 0 or x = 5. Since x represents a length, we take x = 5.
- Now substituting back to find AB:
AB=2x=2×5=10 cm
AB = 10 cm.
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the figure, ABC is a triangle in which AB=AC. A circle through B touches AC at D and intersects AB at P. If D is the mid - point of AC, Find the value of AB :
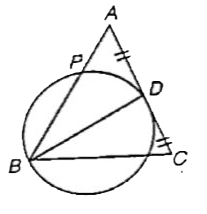 A2APB3APC4APD4√2cm
A2APB3APC4APD4√2cmIf in a triangle AB=a,AC=b and D,E are the mid-points of AB and AC respectively, then DE is equal to
Aa4−b4Ba2−b2Cb4−a4Db2−a2ABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point intersects AB at P. Then, AP : AB is
A3:5B1:4C4:1D2:3
In △ABC, AD bisects ∠A and intersects BC at D. If BC = a ,AC = b and AB = c, then BD = ?
त्रिभुज ABC में, AD कोण A को समद्विभाजित करता है तथा BC को D पर काटता है | यदि BC = a, AC = b और AB = c है, तो BD = ?
View SolutionIn △ABC AB = 7 cm, BC =10cm, and AC = 8 cm. If AD is the angle bisector of ∠BAC, where D is a point on BC, then BD is equal to:
यदि त्रिभुज ABC में, AB = 7 सेमी, BC = 10 सेमी तथा AC =8 सेमी है | यदि AD कोण BAC का समद्विभाजक है, जहाँ D, BC पर स्थित एक बिंदु है, तो BD का मान किसके बराबर है ?
View SolutionIn △ABC ,∠A=50∘. Its sides AB and AC are produced to the point D and E. If the bisectors of the ∠CBD and ∠BCE meet at the point O, then ∠BOC will be equal to:
त्रिभुज ABC में, ∠A=50∘ है | इसकी भुजाओं AB और AC को बिंदु D तथा E तक बढ़ाया जाता है | यदि कोण CBD और कोण BCE के द्विभाजक बिंदु O पर मिलते हैं, तो ∠BOC का मान किसके बराबर होगा ?
View SolutionABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point intersects AB at P. Then AP : AB is :
View SolutionABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point Intersects AB at P. Then AP: AB is :
View Solution