ABCD is a cyclic quadrilateral in which sides AD and BC are produced to meet at P, and sides DC and AB meet at Q when produced. If ∠A=60∘ and ∠ABC=72∘ then ∠DPC−∠BQC=
ABCD एक चक्रीय चतुर्भुज है जिसमें भुजाओं AD तथा BC को बढ़ाया जाता है जो P पर मिलती हैं तथा
भुजाओं DC और AB को बढ़ाया जाता है जो Q पर मिलती हैं | यदि ∠A=60∘ तथा ∠ABC=72∘ है, तो ∠DPC−∠BQC= ?
24∘
40∘
36∘
30∘
To solve the problem, we will follow these steps:
Step 1: Understand the given information
We have a cyclic quadrilateral ABCD with:
- Angle A = 60°
- Angle ABC = 72°
We need to find the difference between angle DPC and angle BQC.
Step 2: Find angle DPC
In triangle APB, we can use the fact that the sum of angles in a triangle is 180°.
Angle PAB+Angle ABP+Angle APB=180°
Substituting the known values:
60°+72°+Angle APB=180°
Now, solve for angle APB:
Angle APB=180°−60°−72°=48°
Since angle DPC is equal to angle APB, we have:
Angle DPC=48°
Step 3: Find angle BQC
In cyclic quadrilateral ABCD, we know that the sum of opposite angles is 180°:
Angle ABC+Angle ADC=180°
Substituting the known value of angle ABC:
72°+Angle ADC=180°
Now, solve for angle ADC:
Angle ADC=180°−72°=108°
Next, consider triangle ADQ:
Angle DAQ+Angle ADQ+Angle AQD=180°
Substituting the known values:
60°+108°+Angle AQD=180°
Now, solve for angle AQD (which is equal to angle BQC):
Angle AQD=180°−60°−108°=12°
Thus, we have:
Angle BQC=12°
Step 4: Calculate the difference
Now we can find the difference between angle DPC and angle BQC:
Angle DPC−Angle BQC=48°−12°=36°
Final Answer
The difference Angle DPC−Angle BQC=36°.
---
We have a cyclic quadrilateral ABCD with:
- Angle A = 60°
- Angle ABC = 72°
In triangle APB, we can use the fact that the sum of angles in a triangle is 180°.
60°+72°+Angle APB=180°
Angle APB=180°−60°−72°=48°
Angle DPC=48°
In cyclic quadrilateral ABCD, we know that the sum of opposite angles is 180°:
Angle ABC+Angle ADC=180°
72°+Angle ADC=180°
Angle ADC=180°−72°=108°
Angle DAQ+Angle ADQ+Angle AQD=180°
60°+108°+Angle AQD=180°
Angle AQD=180°−60°−108°=12°
Angle BQC=12°
Now we can find the difference between angle DPC and angle BQC:
Angle DPC−Angle BQC=48°−12°=36°
The difference Angle DPC−Angle BQC=36°.
Topper's Solved these Questions
Similar Questions
Sides AB and DC of a cyclic quadrilateral ABCD are produced to meet at E, and sides AD and BC are produced to meet at F. If ∠ADC=75∘ and ∠BEC=52∘, then the difference between ∠BAD and ∠AFB ? /
एक चक्रीय चतुर्भुज ABCD की भुजाओं AB और DC को बढ़ाया जाता है जो E पर मिलती हैं तथा भुजाओं AD और BC को बढ़ाया जाता है जो F पर मिलती हैं | यदि ∠ADC=75∘ तथा ∠BEC=52∘ हैं, तो ∠BAD तथा ∠AFB के बीच क्या अंतर है?
View SolutionABCD is a cyclic quadrilateral such that its sides AD and BC produced meet at P and sides AB and DC produced meet at Q. If ∠A=62∘ and ∠ABC=74∘, then the difference between ∠P and ∠Q is:
ABCD एक चक्रीय चतुर्भुज है जो इस प्रकार है कि इसकी भुजाओं AD और BC को बढ़ाने पर वे P पर मिलती हैं तथा AB और DC को बढ़ाने पर वे Q पर मिलती हैं | यदि ∠=62∘ और ∠ABC=74∘ है, तो ∠P और ∠Q के बीच अंतर ज्ञात करें |
View Solution
Knowledge Check
ABCD is cyclic quadrilateral. Sides AB and DC, when produced meet at the point P and sides AD and BC, when produced meet at the point Q. If ∠ADC=85∘and∠BPC=40∘, then ∠CQD is equal to
A30∘B40∘C55∘D85∘A dog takes 3 leaps for every 5 leaps of a hare. If one leap of the dog is equal to 3 leaps of the hare, the ratio of the speed of the dog to that of the hare is :
AA) 9:5BB) 2:3CC) 4:7DD) 5:6The sides AB and CD of a cyclic quadrilateral ABCD are produced to meet at P. The sides AD and BC are produced to meet at Q. If ∠ADC=85∘and∠BPC=40∘, then ∠BAD is equal to
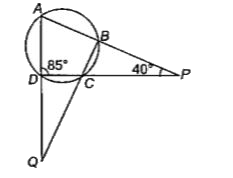 A45∘B30∘C95∘D55∘
A45∘B30∘C95∘D55∘
ABCD is a cyclic quadrilateral such that its sides AD and BD produced meet at P and sides AB and DC produced meet at Q. If ∠A=62∘ and ∠ABC=74∘ then the difference between ∠P and ∠Q is :
ABCD एक चक्रीय चतुर्भज है, जिसकी भुजाएं AD और BCबढ़कर बिंदु P पर मिलती है और भुजा AB और DCबढ़कर बिंदु Q पर मिलती है| यदि ∠A=62∘ और ∠ABC=74∘ है तो ∠P और ∠Q के बीच का अंतर हैः
View SolutionABCD is cyclic quadrilateral. Sides AB and DC, when produced , meet at E and sides BC and AD, when produced, meet at F. If ∠BFA=60∘ and ∠AED=30∘ , then the measure of ∠ABC is :
View SolutionABCD is a cyclic quadrilateral. AB and DC are produced to meet at P. If ∠ADC=70∘ and ∠DAB=60∘, then the ∠PBC+∠PCB is
View SolutionABCD is a cyclic quadrilateral. AB and DC are produced to meet at P. If ∠ADC=70degreeand∠DAB=60degree, then the ∠PBC+∠PCB is
View SolutionSides AB and DC of a cyclic quadrilateral ABCD are produced to meet at E, and sides AD and BC are produced to meet at F. If ∠ADC=75∘ and ∠BEC=52∘, then the difference between ∠BAD and ∠AFB ? /
एक चक्रीय चतुर्भुज ABCD की भुजाओं AB और DC को बढ़ाया जाता है जो E पर मिलती हैं तथा भुजाओं AD और BC को बढ़ाया जाता है जो F पर मिलती हैं | यदि ∠ADC=75∘ तथा ∠BEC=52∘ हैं, तो ∠BAD तथा ∠AFB के बीच क्या अंतर है?
View Solution
