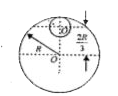
A thin disc of mass 9 M and radius R from which a disc of radius R/3 is cut. Moment ofinertia,of the disc about an axis passing through O and perpendicular to plane of disc is
409MR2
379MR2
9MR2
4MR2
The correct Answer is:D
As the mass is uniformly distributed on the disc, so mass density = =9MπR2
Mass of removed portion = 9MπR2×π(R3)2=M.
So the moment of inertia of removed portion about the stated axis by theorem of parallel axis.
I1=M2(R3)2+M(2R3)2 (1)
If the disc would not have been removed, then the moment of inertia of complete disc about the stated axis is I2
So the moment of inertia of the disc is I2−I1⇒I2−I1=4MR2
Similar Questions
From a disc of mass 2kg and radius 4m a small disc of radius 1 m with center O' is extracted . The new moment of inertia,about an axis passing through O perpendicular to plane of disc is

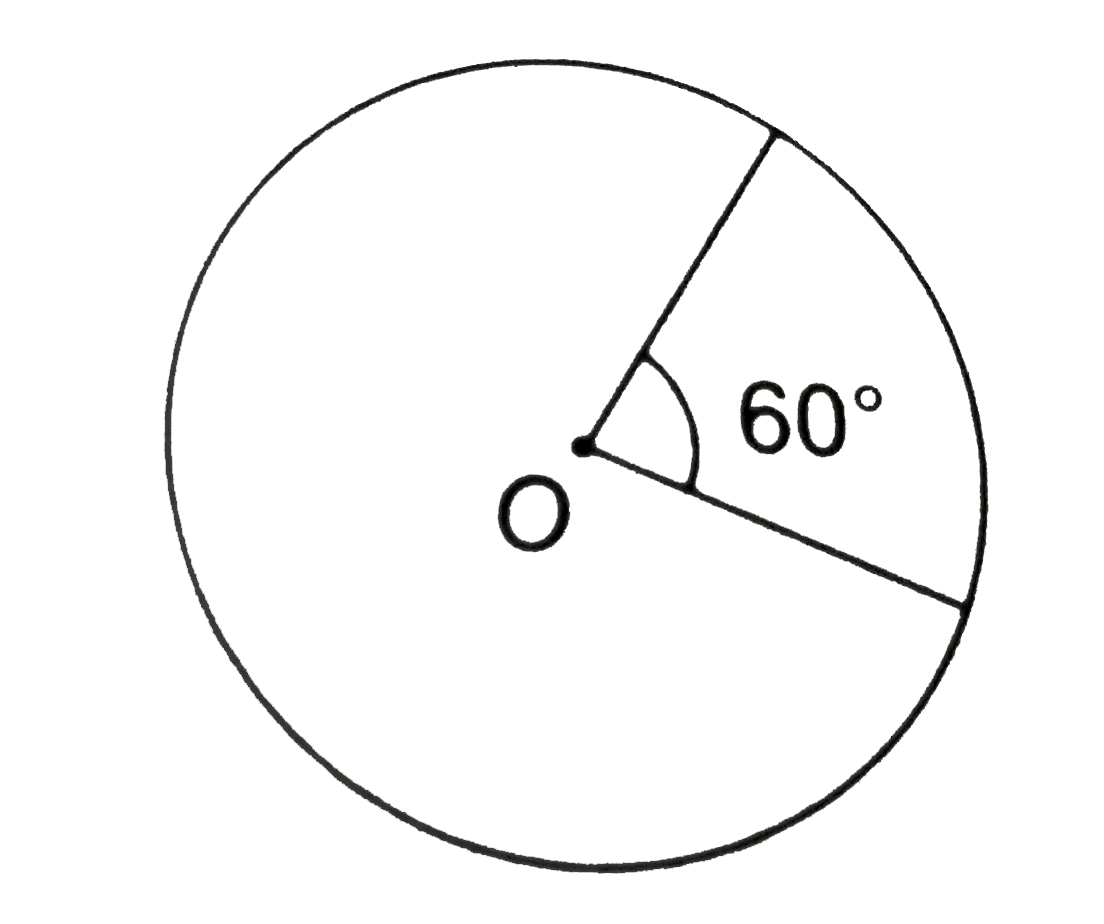
View SolutionFrom a circular disc of mass M and radius R, a part of 60∘ is removed. The M.I. of the remaining portion of disc about an axis passing through the center and perpendicular to plane of disc is
View Solution
Knowledge Check
A thin disc of mass 9M and radius R from which a disc of radius R/3 is cut shown in figure. Then moment of inertia of the remaining disc about O, perpendicular to the plane of disc is -
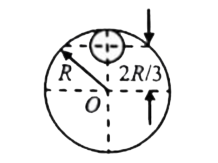 A4MR2B9MR2C379MR2D409MR2
A4MR2B9MR2C379MR2D409MR2From a disc of mass 2kg and radius 4m a small disc of radius 1 m with center O' is extracted . The new moment of inertia,about an axis passing through O perpendicular to plane of disc is
 A16kgm2B12kgm2C25516kgm2D24716kgm2
A16kgm2B12kgm2C25516kgm2D24716kgm2Mass of bigger disc having radius 2R is M. A disc of radius R is cut from bigger disc. Moment of intertia of disc about an axis passing through periphery and perpendicular to plane is
A27MR28B29MR28C3.5 MRD2 MR2
Surface mass density of disc of mass m and radius R is Kr2. Then its moment of inertia w.r.t. axis of rotation passing through center and perpendicular to the plane of disc is :
View SolutionThe diagram shows a uniform disc of mass M & radius 'a', if the moment of inertia of the disc about the axis XY is I, its moment of inertia about an axis through O and perpendicular to the plane of the disc is

View SolutionFour similar point masses (m each) are symmetrically placed on the circumference of a disc of mass M and radius R. Moment of inertia of the system about an axis passing through centre O and perpendicular to the plane of the disc will be
View SolutionFrom a circular disc of mass M and radius R, a part of 60∘ is removed. The M.I. of the remaining portion of disc about an axis passing through the center and perpendicular to plane of disc is

View SolutionSurface mass density of disc of mass m and radius R is α−Kr2. Then its moment of inertia w.r.t. axis of rotation passing through center and perpendicular to the plane of disc is :
View Solution
